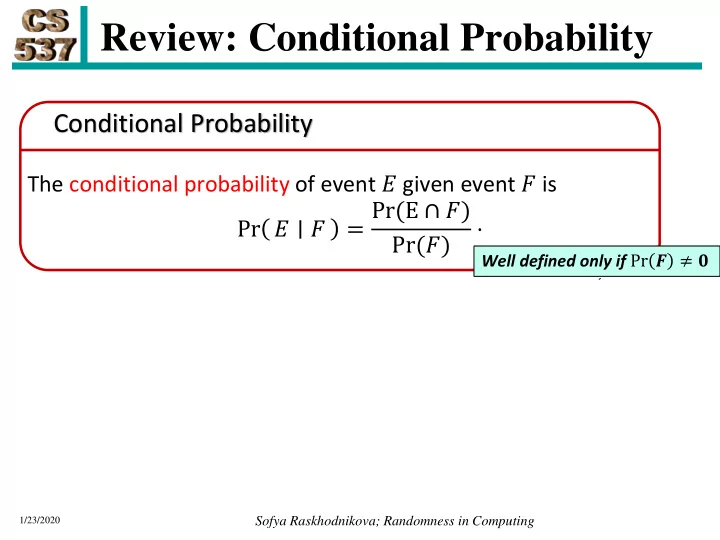
Review: Conditional Probability Conditional Probability The - PowerPoint PPT Presentation

Review: Conditional Probability Conditional Probability The conditional probability of event 𝐹 given event 𝐺 is = Pr(E ∩ 𝐺) Pr 𝐹 𝐺 ⋅ Pr(𝐺) Well defined only if Pr 𝑮 ≠ 𝟏 Sofya Raskhodnikova; Randomness in Computing 1/23/2020

Review question Card dealing We deal two cards. What is the probability that the second card is an ace, given that the first is an ace? A. 3/52 B. 3/51 C. 4/52 D. 5/52 E. None of the answers above are correct. Sofya Raskhodnikova; Randomness in Computing 1/23/2020

Product rule For any two events 𝐹 1 and 𝐹 2 , Pr 𝐹 1 ∩ 𝐹 2 = Pr(𝐹 1 ) ⋅ Pr 𝐹 2 |𝐹 1 . For all events 𝐹 1 , … , 𝐹 𝑜 , 𝑜−1 𝐹 𝑗 ) 𝑜 Pr(∩ 𝑗=1 𝐹 𝑗 ) = Pr 𝐹 1 ⋅ Pr 𝐹 2 𝐹 1 ⋅ … ⋅ (𝐹 𝑜 | ∩ 𝑗=1 Sofya Raskhodnikova; Randomness in Computing 1/23/2020

Law of Total Probability For any two events 𝐵 and 𝐹, Pr 𝐵 = Pr(𝐵 ∩ 𝐹) + Pr(𝐵 ∩ 𝐹) = Pr(𝐵|𝐹) ⋅ Pr 𝐹 + Pr(𝐵|𝐹) ⋅ Pr 𝐹 Let A be an event and let 𝐹 1 , … , 𝐹 𝑜 be mutually disjoint events whose union is Ω . Pr(𝐵) = Pr(𝐵 ∩ 𝐹 𝑗 ) = Pr(𝐵 ∣ 𝐹 𝑗 ) ⋅ Pr(𝐹 𝑗 ) . 𝑗∈ 𝑜 𝑗∈ 𝑜 Sofya Raskhodnikova; Randomness in Computing 1/23/2020

Bayes’ Law For any two events 𝐵 and 𝐹 with Pr(A) ≠ 0, Pr 𝐹|𝐵 = Pr(𝐵|𝐹) ⋅ Pr(𝐹) Pr(𝐵) Let A be an event with Pr(A) ≠ 0 and let 𝐹 1 , … , 𝐹 𝑜 be mutually disjoint events whose union is Ω . 𝑘 |𝐵) = Pr(𝐹 𝑘 ∩ 𝐵) Pr(𝐵|𝐹 𝑘 ) ⋅ Pr(𝐹 𝑘 ) Pr(𝐹 = 𝑗∈ 𝑜 Pr(𝐵 ∣ 𝐹 𝑗 ) ⋅ Pr(𝐹 𝑗 ) Pr(𝐵) Sofya Raskhodnikova; Randomness in Computing 1/23/2020