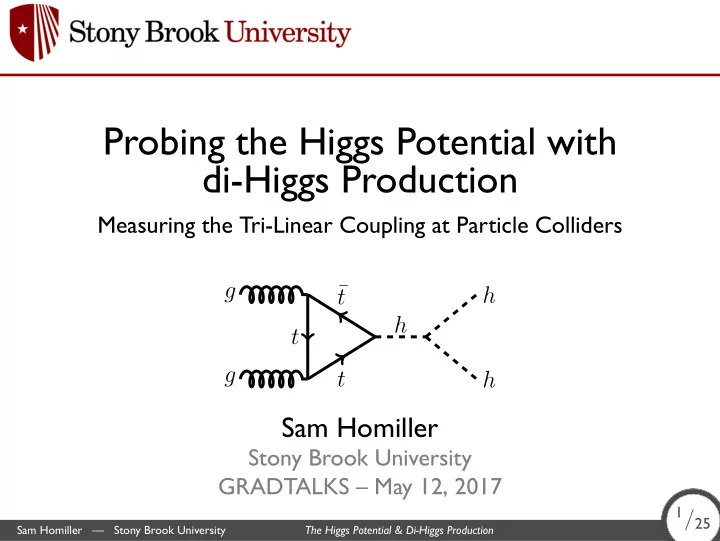
Probing the Higgs Potential with di-Higgs Production Measuring the - PowerPoint PPT Presentation

Probing the Higgs Potential with di-Higgs Production Measuring the Tri-Linear Coupling at Particle Colliders g ¯ h t h t g t h Sam Homiller Stony Brook University GRADTALKS – May 12, 2017 1 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 1/25

Outline • The Standard Model Higgs. The Standard Model, the Higgs potential, spontaneous symmetry breaking, and Higgs interactions. • The Electroweak Phase Transition. The finite temperature Higgs potential, behavior of the phase transition, and implications for baryogenesis. • Measuring di-Higgs production at colliders. Higgs decays and detection, LHC phenomenology, and prospects at colliders 2 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 2/25

The Standard Model 3 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 3/25

The Standard Electroweak Theory The Standard Model is a Gauge Theory G SM = SU (3) c × SU (2) L × U (1) Y Describes electroweak interactions (e.g., β decay!) u e e d Z e W − ν e ¯ ν e ν e But W ± , Z boson masses are forbidden by gauge symmetry... ⇒ spontaneous symmetry breaking! = 4 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 4/25

quantum-bits.org The Higgs Potential Mexican Hats! In the Standard Model, the Higgs potential takes a simple form: V ( ϕ ) = − µ 2 ϕ † ϕ + λ ( ϕ † ϕ ) 2 where ( w + ) ϕ ( x ) = 1 2 ( v + h + iw 0 ) √ √ and v = µ 2 / 2 λ . 5 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 5/25

Higgs Potential in “Unitary Gauge” In unitary gauge, w ± , w 0 become the longitudinal polarizations of W ± , Z . ( ) ϕ ( x ) = 1 0 √ v + h ( x ) 2 The Higgs potential becomes: V ( h ) = − 1 h h 2 − λ 2 m h h 3 − 1 2 m 2 4 λh 4 √ √ where m h = 2 λv . 2 µ = Note: There are only two free parameters: m h = 125 GeV and v = 246 ∼ GeV, both have been measured! 6 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 6/25

Higgs Interactions What’s the Big Deal with Scalars? The Higgs is the only particle with self-interactions: V int ( h ) = λ 3 3! h 3 + λ 4 4! h 4 where in the SM, λ 3 = 3 m 2 h /v , h /v 2 . λ 4 = 3 m 2 λ 3 λ 4 7 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 7/25

ctc.cam.ac.uk The Higgs and Cosmology CMB Measurements (Planck, etc.) T ∼ 1 eV Light Element Abundances (He fraction, etc.) T ∼ 1 MeV EWPT (Particle Colliders!) T ∼ 100 GeV 8 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 8/25

The Electroweak Phase Transition In the early universe interactions with the plasma give the Higgs an effective potential: V eff ( h, T ) ≊ 1 c ) h 2 − eT h 2 ) 3 / 2 + λ ( 2 c ( T 2 − T 2 4 h 4 12 π Note that at T > T c , the mass term is positive: SU (2) L × U (1) Y is unbroken. We therefore expect a phase transition at high temperatures in the early universe. 9 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 9/25

The Electroweak Phase Transition The phase transition can be second order (driven by thermal fluctuations) or first order (starts with tunneling). Credit: A. Banerjee second order first order The barrier between phases is created by other particles coupled to the Higgs! 10 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 10/25

Baryogenesis at the Electroweak Phase Transition For a strong first order transition tunneling nucleates bubbles that expand faster than the Hubble rate. Processes at the bubble wall can lead to CP and Baryon number violation – potentially the source of the baryon asymmetry! arXiv:0205279 11 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 11/25

Probing Baryogenesis via the Higgs Potential The strength of the transition is determined by the shape of the potential, with the order parameter given by ∼ h 3 term ( e/ 4 π ) v ( T c ) ≊ T c λ Fixed to SM value which is large enough only for m h ≲ 48 GeV. To get a strong first order EWPT, we need additional contributions to the Higgs potential – these can be explored via colliders! 12 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 12/25

Probing the Higgs Potential The Trillion Dollar Argument Clearly we need to understand the Higgs potential beyond the mass term. The only way to do this is to look for Higgs couplings to itself ⇒ di-Higgs Production! = We could also look for h → hhh , but this is much harder. How do we look for di-Higgs production at colliders? 13 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 13/25

Di-Higgs Production The λ 3 vertex contributes to di-Higgs production: g ¯ h t h t g t h There’s also an interference term: t g h t t g h t 14 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 14/25

Higgs Decay Modes Higgs couples to particles proportional to their mass. Final State Branching Ratio b ¯ 0.58 b 0.215 W + W − 0.09 gg 0.06 τ + τ − 0.026 ZZ 0.00228 γγ Table: Branching ratios of various decay modes for a 125 GeV Higgs boson. 15 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 15/25

Higgs Decay Modes Loop induced couplings ( H → gg , H → γγ ) are also γ important. h γ Diphoton channel has incredibly tiny branching ratio – 0 . 228% of decays! γ h But photon mass resolution γ is extremely good – the diphoton channel was γ essential to the original Higgs discovery. h γ 16 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 16/25

atlasexperiment.org Some LHC Phenomenology (A Theorist’s Understanding) Muon Spectrometer Hadronic Calorimeter (Jets, etc.) Electronic Calorimeter (Photons & Electrons) Tracker (Four-Momenta via Magnets) Pixel Detector (Interaction Pts., Vertices) 17 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 17/25

B-Tagging Making Sense of QCD Madness b / ¯ b quarks form B -mesons, which have lifetimes ∼ 10 − 12 s Travel a few millimeters before decaying! Tagging efficiency roughly 75% , changes with p T and η . c -jets and light jets can sometimes be mis-tagged! Credit: Nazar Bartosik 18 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 18/25

How do we search for two Higgs? If One Wasn’t Hard Enough Two Higgs bosons have a variety of possible decay combinations: • hh → b ¯ bb ¯ b Dominant decay mode – 1/3 of events! Poor mass resolution – difficult to distinguish from ZZ • hh → γγγγ Great mass resolution. BR ∼ 8 × 10 − 6 ! Not even one will be produced at the LHC. hh → b ¯ bγγ Best combination of mass resolution and large branching ratio! The “golden channel”. Other Possibilities: hh → ( b ¯ b )( ZZ ∗ ) → ( b ¯ b )( ℓ + ℓ − ℓ + ℓ − ) , hh → ( b ¯ b )( µ + µ − ) hh → ( b ¯ b )( τ + τ − /W + W − ) , hh → ( b ¯ b )( Zγ ) 19 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 19/25

Search Strategy Backgrounds Now that we’ve chosen a decay channel, have to tabulate backgrounds. What other processes lead to b ¯ g ¯ bγγ ? b • b ¯ bγγ h • Z ( → b ¯ b ) + H ( → γγ ) • b ¯ b + H ( → γγ ) g t ( → b ¯ • t ¯ b b + X ) + H ( → γγ ) Note: Ordinary single Higgs production is now a background! Also other backgrounds from mistagging jets as b -jets, jets faking photons... 20 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 20/25

Search Strategy Cut and Count.. Now that we’ve identified all the backgrounds, we have to play a game of statistics. Come up with a set of cuts: which events do we want to count? Once you’ve chosen cuts, count number of signal events you expect ( S ) and number of backgrounds ( B ). √ The “significance” is then σ = S/ S + B Goal: Come up with set of cuts that optimizes signal / background. 21 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 21/25

Simulations! All the ROOT Plots γ γ γ γ h(b b )h( ) 0.18 h(b b )h( ) 0.5 γ γ γ γ t t h( ) t t h( ) 0.16 γ γ γ γ b b h( ) b b h( ) γ γ γ γ b b 0.14 b b 0.4 0.12 0.3 0.1 0.08 0.2 0.06 0.04 0.1 0.02 0 0 0 50 100 150 200 250 0 50 100 150 200 250 M (GeV/c^2) M (GeV/c^2) γ γ b b γ γ γ γ h(b b )h( ) h(b b )h( ) 0.08 0.07 γ γ γ γ t t h( ) t t h( ) γ γ γ γ b b h( ) 0.07 b b h( ) 0.06 γ γ γ γ b b b b 0.06 0.05 0.05 0.04 0.04 0.03 0.03 0.02 0.02 0.01 0.01 0 − − − − − 0 50 100 150 200 250 300 350 400 450 500 1 0.8 0.6 0.4 0.2 0 0.2 0.4 0.6 0.8 1 θ Jet Pair P (GeV/c) cos T H 22 / 25 Sam Homiller — Stony Brook University The Higgs Potential & Di-Higgs Production 22/25