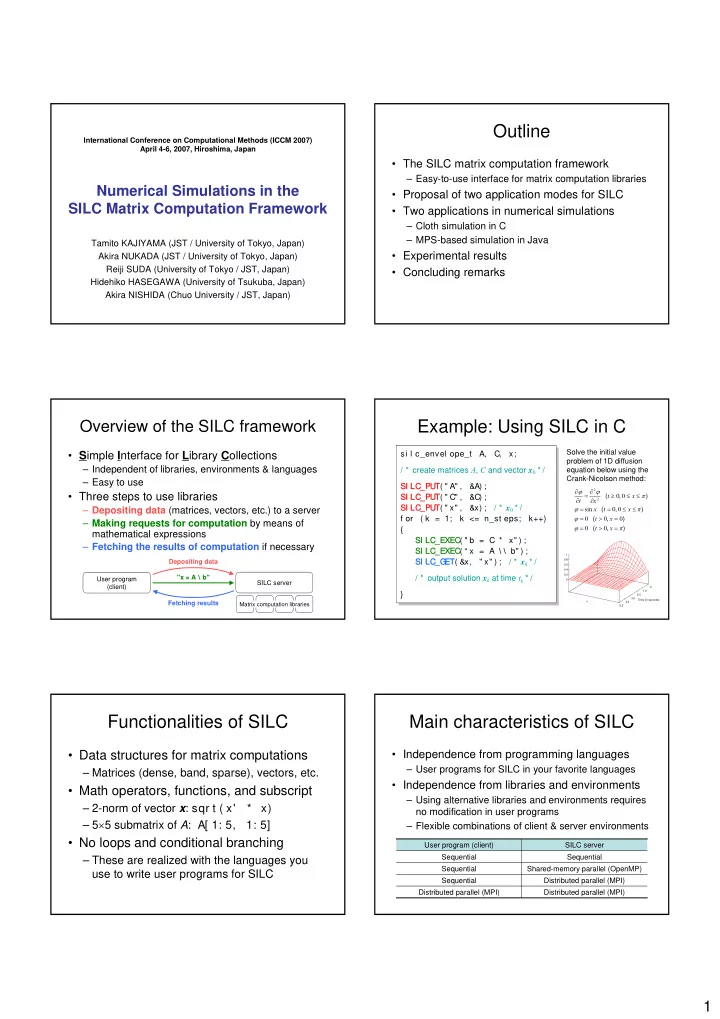

Outline International Conference on Computational Methods (ICCM 2007) April 4-6, 2007, Hiroshima, Japan • The SILC matrix computation framework – Easy-to-use interface for matrix computation libraries Numerical Simulations in the • Proposal of two application modes for SILC SILC Matrix Computation Framework • Two applications in numerical simulations – Cloth simulation in C – MPS-based simulation in Java Tamito KAJIYAMA (JST / University of Tokyo, Japan) • Experimental results Akira NUKADA (JST / University of Tokyo, Japan) Reiji SUDA (University of Tokyo / JST, Japan) • Concluding remarks Hidehiko HASEGAWA (University of Tsukuba, Japan) Akira NISHIDA (Chuo University / JST, Japan) Overview of the SILC framework Example: Using SILC in C Solve the initial value • S imple I nterface for L ibrary C ollections si l c_envel ope_t A, C, x; si l c_envel ope_t A, C, x; problem of 1D diffusion – Independent of libraries, environments & languages / * create matrices A , C and vector x 0 * / equation below using the / * create matrices A , C and vector x 0 * / Crank-Nicolson method: – Easy to use SI LC_PUT( " A" , &A) ; SI LC_PUT SI LC_PUT( " A" , &A) ; SI LC_PUT ∂ ϕ ∂ ϕ 2 • Three steps to use libraries = t ≥ ≤ x ≤ π ( 0 , 0 ) SI LC_PUT( " C" , &C) ; SI LC_PUT SI LC_PUT SI LC_PUT( " C" , &C) ; ∂ t ∂ x 2 SI LC_PUT( " x" , &x) ; / * x 0 * / SI LC_PUT ϕ = x t = ≤ x ≤ π – Depositing data (matrices, vectors, etc.) to a server sin ( 0 , 0 ) SI LC_PUT( " x" , &x) ; / * x 0 * / SI LC_PUT f or ( k = 1; k <= n_st eps; k++) ϕ = t > x = 0 ( 0 , 0 ) f or ( k = 1; k <= n_st eps; k++) – Making requests for computation by means of ϕ = t > x = π { 0 ( 0 , ) { mathematical expressions SI LC_EXEC SI LC_E XEC( " b = C * x" ) ; SI LC_EXEC SI LC_E XEC( " b = C * x" ) ; – Fetching the results of computation if necessary XEC( “ x = A ∖ ∖ b" ) ; SI LC_E SI LC_EXEC XEC( “ x = A ∖ ∖ b" ) ; SI LC_E SI LC_EXEC 1 SI LC_G SI LC_G ET( &x, " x" ) ; / * x k * / ET 0.8 Depositing data SI LC_G SI LC_G ET ET( &x, " x" ) ; / * x k * / 0.6 0.4 "x = A \ b" / * output solution x k at time t k * / 0.2 User program / * output solution x k at time t k * / 0 SILC server (client) 0 1.0 } } 2.0 3.0 Time (in seconds) Fetching results x 4.0 Matrix computation libraries 5.0 Functionalities of SILC Main characteristics of SILC • Data structures for matrix computations • Independence from programming languages – User programs for SILC in your favorite languages – Matrices (dense, band, sparse), vectors, etc. • Independence from libraries and environments • Math operators, functions, and subscript – Using alternative libraries and environments requires – 2-norm of vector x : sqr t ( x' * x) no modification in user programs – 5 × 5 submatrix of A : A[ 1: 5, 1: 5] – Flexible combinations of client & server environments • No loops and conditional branching User program (client) SILC server Sequential Sequential – These are realized with the languages you Sequential Shared-memory parallel (OpenMP) use to write user programs for SILC Sequential Distributed parallel (MPI) Distributed parallel (MPI) Distributed parallel (MPI) 1
Proposal of two application modes Limited application mode • Limited application mode Use SILC only in the most time-consuming, Use SILC only in the most time-consuming, – Use SILC only in the most time-consuming, computationally intensive part of a program computationally intensive part of a program computationally intensive part of a program • Comprehensive application mode • Pros – Move all relevant data to a SILC server, and – Easy to apply (especially to existing user programs) implement overall simulations using SILC's • Cons mathematical expressions – Smaller data size due to a limited amount of main memory in client environments Abbreviations: – Frequent data communications (larger overheads) • LTD for the limited application mode • CMP for the comprehensive application mode Application #1 Original code For each time step: • Time-dependent simulation of cloth motion 1. Calculate force f and its derivatives ∂ f / ∂ x and 1. Calculate force f and its derivatives ∂ f / ∂ x and – Mass-spring model ∂ f / ∂ v (Jacobian matrices). ∂ f / ∂ v (Jacobian matrices). – Implicit Euler method 2. Solve a linear system A ∆ v = b , where – Solving a sparse linear 2. Solve a linear system A ∆ v = b , where ∂ ∂ system is necessary ⎧ ⎫ f f = − Δ − Δ A ⎨ M t 2 t ⎬ ∂ ∂ for each time step ⎩ ⎭ x v ⎧ ∂ ⎫ • Original code f = + Δ Δ ⎨ t ⎬ t b f v ⎩ 0 ∂ 0 ⎭ x – Sequential program in C – Linear solver: CG method 3. Update particle motion. 3. Update particle motion. = + Δ v v v – Visualization: OpenGL 0 = + Δ t x x v 0 New code in the LTD mode Comprehensive application mode Original code using Lis* New code using SILC Move all relevant data to a SILC server, Move all relevant data to a SILC server, LI S_M ATRI X A; LI S_VECTO R b, dv; si l c_envel ope_t A, b, dv; and implement overall simulations using LI S_M ATRI X A; LI S_VECTO R b, dv; si l c_envel ope_t A, b, dv; and implement overall simulations using SILC's mathematical expressions f or ( k = 1; k <= n_st eps; k++) f or ( k = 1; k <= n_st eps; k++) SILC's mathematical expressions f or ( k = 1; k <= n_st eps; k++) f or ( k = 1; k <= n_st eps; k++) { { { { / * 1. Calculate f , ∂ f / ∂ x and ∂ f / ∂ v * / / * 1. Calculate f , ∂ f / ∂ x and ∂ f / ∂ v * / / * 1. Calculate f , ∂ f / ∂ x and ∂ f / ∂ v * / / * 1. Calculate f , ∂ f / ∂ x and ∂ f / ∂ v * / ⋮ ⋮ ⋮ ⋮ / * 2. Solve A ∆ v = b * / / * 2. Solve A ∆ v = b * / • Pros / * 2. Solve A ∆ v = b * / / * 2. Solve A ∆ v = b * / l ve( A, b, dv, / * ∆ v * / l i s_ i s_so sol ve l ve( A, b, dv, / * ∆ v * / SI LC I LC_P _PUT UT( " A" , &A) ; l i s_ i s_so sol ve SI LC I LC_P _PUT UT( " A" , &A) ; – User programs are free from massive data l i s_par am s, SI LC I LC_P _PUT UT( " b" , &b) ; l i s_par am s, SI LC I LC_P _PUT UT( " b" , &b) ; EXEC( " dv = A ∖ ∖ b" ) ; l i s_opt i ons, SI L SI LC_ C_EX EXEC( " dv = A ∖ ∖ b" ) ; l i s_opt i ons, SI LC_ SI L C_EX – Reduced data communications (smaller overheads) ET( &dv, " dv" ) ; / * ∆ v * / l i s_st at us) ; SI L SI LC_ C_G G E ET( &dv, " dv" ) ; / * ∆ v * / l i s_st at us) ; SI LC_ SI L C_G G E • Cons / * 3. Update particle motion * / / * 3. Update particle motion * / / * 3. Update particle motion * / / * 3. Update particle motion * / ⋮ ⋮ ⋮ ⋮ – Existing user programs may require a major rewrite } } } } * An iterative solvers library written in C. 2
Recommend
More recommend