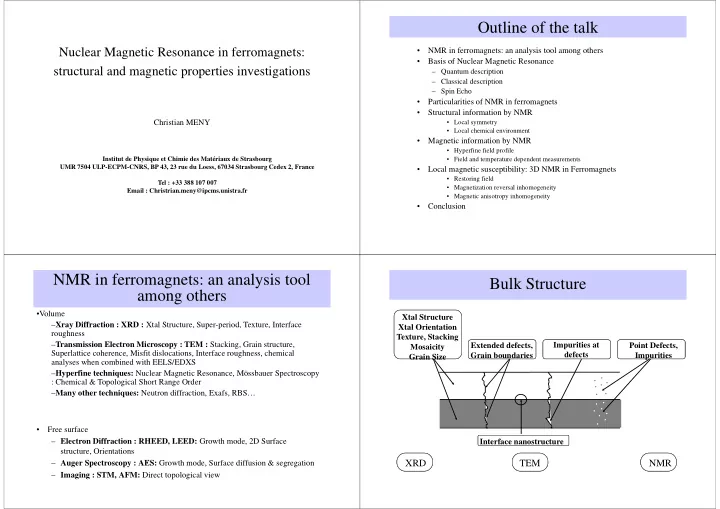
Outline of the talk Nuclear Magnetic Resonance in ferromagnets: - PowerPoint PPT Presentation

Outline of the talk Nuclear Magnetic Resonance in ferromagnets: • NMR in ferromagnets: an analysis tool among others • Basis of Nuclear Magnetic Resonance structural and magnetic properties investigations – Quantum description – Classical description – Spin Echo • Particularities of NMR in ferromagnets • Structural information by NMR Christian MENY • Local symmetry • Local chemical environment • Magnetic information by NMR • Hyperfine field profile Institut de Physique et Chimie des Matériaux de Strasbourg • Field and temperature dependent measurements UMR 7504 ULP-ECPM-CNRS, BP 43, 23 rue du Loess, 67034 Strasbourg Cedex 2, France • Local magnetic susceptibility: 3D NMR in Ferromagnets • Restoring field Tel : +33 388 107 007 • Magnetization reversal inhomogeneity Email : Christrian.meny@ipcms.unistra.fr • Magnetic anisotropy inhomogeneity • Conclusion NMR in ferromagnets: an analysis tool Bulk Structure among others •Volume Xtal Structure – Xray Diffraction : XRD : Xtal Structure, Super-period, Texture, Interface Xtal Orientation roughness Texture, Stacking – Transmission Electron Microscopy : TEM : Stacking, Grain structure, Extended defects, Impurities at Point Defects, Mosaicity Superlattice coherence, Misfit dislocations, Interface roughness, chemical defects Grain boundaries Impurities Grain Size analyses when combined with EELS/EDXS – Hyperfine techniques: Nuclear Magnetic Resonance, Mössbauer Spectroscopy : Chemical & Topological Short Range Order – Many other techniques: Neutron diffraction, Exafs, RBS… • Free surface – Electron Diffraction : RHEED, LEED: Growth mode, 2D Surface Interface nanostructure structure, Orientations XRD TEM NMR – Auger Spectroscopy : AES: Growth mode, Surface diffusion & segregation – Imaging : STM, AFM: Direct topological view

Outline of the talk Interface Structure • NMR in ferromagnets: an analysis tool among others • Basis of Nuclear Magnetic Resonance – Quantum description TEM XRD – Classical description – Spin Echo • Particularities of NMR in ferromagnets • Structural information by NMR • Local symmetry Long Range Alloyed Interface • Local chemical environment Dislocations Roughness Interface Compound • Magnetic information by NMR • Hyperfine field profile • Field and temperature dependent measurements • Local magnetic susceptibility: 3D NMR in Ferromagnets • Restoring field • Magnetization reversal inhomogeneity Surface • Magnetic anisotropy inhomogeneity AFM • Conclusion STM NMR AES LEED RHEED Basis of Nuclear Magnetic Resonance Basis of Nuclear Magnetic Resonance • Characteristics of nuclei: • Quantum mechanics: Resonant – Spin Absorption of Photon • Has to be non zero :from ½ ( 1 H) to 7 ( 176 Lu) – Odd number of neutrons or protons  1/2 – gyromagnetic ratio  Photon Spin 1/2   L Resonance:  L H 0 = 0 • The ratio of magnetic dipole moment to the angular momentum H 1 (  )  1/2 H 0  0 – M=  L – Characterizes the motion in a magnetic field H 0 : Static magnetic field: Zeeman Effect H 1 : Radiofrequency field : Resonant Absorption

Observable Elements & Sensitivity Outline of the talk • NMR signal can be obtained for all nuclei with non zero nuclear spin • NMR in ferromagnets: an analysis tool among others • Basis of Nuclear Magnetic Resonance – Quantum description Medium Bad No Good He H – Classical description Be C N O Ne Li B F – Spin Echo Na Mg Si S Cl Ar Al P • Particularities of NMR in ferromagnets K Ca Sc Ti Cr Mn Fe Co Ni Cu Zn Ga Ge As Se Br Kr V • Structural information by NMR Rb Sr Y Zr Nb Mo Tc Ru Rh Pd Ag Cd In Sn Sb Te I Xe • Local symmetry Cs Ba La Hf Ta W Re Os Ir Pt Au Hg Tl Pb Bi Po At Rn • Local chemical environment Ce Pr Nd Pm Sm Eu Gd Tb Dy Ho Er Tm Yb Lu • Magnetic information by NMR • Hyperfine field profile In practice : • Field and temperature dependent measurements Organic materials: H, F, P ( 17 O, 4 N, 13 C) • Local magnetic susceptibility: 3D NMR in Ferromagnets Ga, B, As, In (Si, Se, Te) Metalloids: Li, Na, Al (Be, K) • Restoring field Normal metals: • Magnetization reversal inhomogeneity V, Mn, Co, Cu, Nb, La, Re (Y, Pt, Ta) Transition Metals: • Magnetic anisotropy inhomogeneity Pr, Eu, Tb, Ho (Nd, Gd) Rare Earths: • Conclusion To increase sensitivity: Low Temperature : NMR signal increases as 1/T (Curie law) NMR signal increases as  2 =  2 H 2 High Field : NMR Classical description Motion of a particle rotating about an axis • We consider a particle moving about an axis • The Newton’s second law:   d p  • Why is it possible to use a classical description.  dt F – NMR measurements are done simultaneously on a very • If we multiply both sides by the cross product with the position vector large number of nuclei  r we obtain:     d p • NMR processes much easier to explain    r F r dt      d p    r F r dt    d p    r dt    ( ) d r p    dt

Motion of an object rotating about an axis The case of a nuclear spin      Now a consider a nuclear spin of magnetic moment M in a magnetic L r p field Is called the angular momentum of the particle From the magnetic field results a torque:   So we can write:        d L M H   dt And its magnetic moment is defined through is gyromagnetic ratio The sum of the torques is equal to the time derivative of the angular M=  L momentum. So the spin equation of motion becomes This is the rotational analog of the Newton’s second law    d L   dt    d M    M H dt Motion of an object rotating about an axis Motion of an object rotating about an axis The case of a nuclear spin The case of a nuclear spin  • Classical view point: Nuclear Precession   d M    M H d M /dt =  M  H dt H 0 The direction of the motion is perpendicular to the plan defined by M and H This is a precession motion.

NMR Classical description NMR Classical description • How to give a classical description of this quantum process? • A rf field is the sum of 2 fields rotating in opposite directions  1/2 Photon Spin 1/2   L Resonance:  L H 0 = 0 H 1 (  )  1/2 H 0  0 We need to consider 2 fields: H 0 : Static magnetic field: Zeeman Effect (OK with what we know) H 1 : Radiofrequency field : Resonant Absorption ??????? NMR - Macroscopic Viewpoint NMR - Macroscopic Viewpoint Rotating frame (  L ) Rotating frame (  L ) Rotating frame (  ) Laboratory frame Laboratory frame z z At Resonance H’ 0 = H 0 -  Off - Resonance z H 0 Z Z H 0 H’ 0 =0 H 0 -  H e H 0 -  M n  L  M n  L   M n    M n  L  M n is Static M n  e  y y y y Y Y H 1  x x x  L  L x H 1 H 1 X X In a frame rotating at the same angular velocity  L as Pulsed H 1 Pulse Duration:  Turn Angle:    the spin , the spin looks static.  2 :  M n  H 0  : M n Reversal Particular Turn Angles In the frame rotating at velocity  L the apparent static magnetic field is ZERO

NMR - Macroscopic Viewpoint NMR - Macroscopic Viewpoint Classical (Hahn) Spin Echo Pulse Sequence          What is the NMR signal? Free Induction Spin Echo Decay The component of the nuclear magnetization in the xy plane H 1 on H 1 on 90° 180° t NMR signal is maximum when the turning angle is  2     d  d M Dephasing Rephasing Dephasing A way to measure the NMR signal : Spin Echo (Spread of  L ) R E Z V Slow Slow Fast E M n R M n M n S A Y Y Y L H 1 Fast Slow Fast X X Rotating Frame View - XY Plane X Pulsed NMR Setup NMR – Relaxation times • After a spin echo; the spin system has to go back to Transmitter Receiver equilibrium: RF pulse (µS) Absorption Emission • 2 relaxation processes: NMR 1 to 1000 MHz Magnet • vanishing of the nuclear magnetization in the xy Induction Sample coil (  scan) (H scan) Signal plane • Reorientation of the magnetization towards the Z axis. FID or Spin Echo integral intensity in Frequency Domain Free Induction Decay Relaxation along the laboratory Z direction: in Time Domain spin lattice relaxation time T1 (10 ms) Relaxation into the xy plane : spin-spin relaxation time T2 (10  s) H or  t Fourier transform NMR Field or Frequency Scan NMR