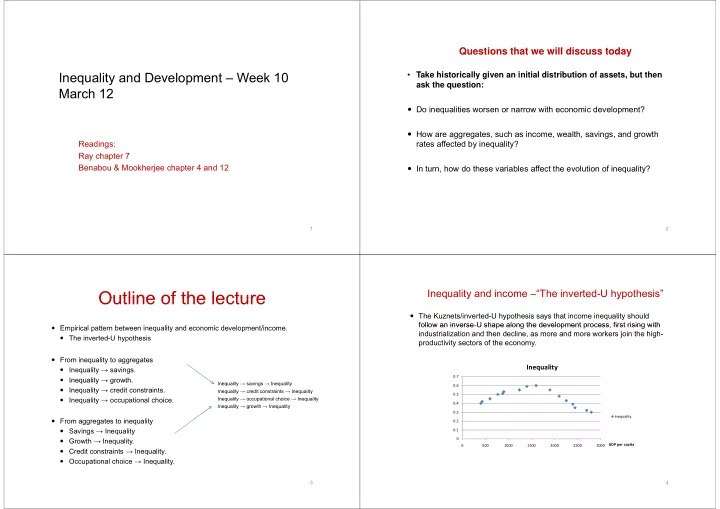

Questions that we will discuss today • Take historically given an initial distribution of assets, but then Inequality and Development – Week 10 T k hi i ll i i i i l di ib i f b h ask the question: March 12 Do inequalities worsen or narrow with economic development? How are aggregates, such as income, wealth, savings, and growth Readings: rates affected by inequality? Ray chapter 7 Ray chapter 7 Benabou & Mookherjee chapter 4 and 12 In turn, how do these variables affect the evolution of inequality? 1 2 Outline of the lecture Outline of the lecture Inequality and income –“The inverted-U hypothesis” q y yp The Kuznets/inverted-U hypothesis says that income inequality should follow an inverse U shape along the development process, first rising with follow an inverse-U shape along the development process first rising with Empirical pattern between inequality and economic development/income. E i i l tt b t i lit d i d l t/i industrialization and then decline, as more and more workers join the high- The inverted-U hypothesis productivity sectors of the economy. From inequality to aggregates Inequality Inequality → savings. 0.7 Inequality → growth Inequality → growth. Inequality → savings → Inequality 0.6 Inequality → credit constraints. Inequality → credit constraints → Inequality 0.5 Inequality → occupational choice. Inequality → occupational choice → Inequality 0.4 Inequality → growth → Inequality 0.3 inequality From aggregates to inequality 0.2 Savings → Inequality 0.1 Growth → Inequality. 0 GDP per capita 0 500 1000 1500 2000 2500 3000 Credit constraints → Inequality. Occupational choice → Inequality. p q y 3 4
First uneven, then compensatory? , p y Income and inequality: Uneven and compensatory changes Income and inequality: Uneven and compensatory changes 1. Economic development: Large transfers of people from relatively poor to relatively advanced sectors of the economy advanced sectors of the economy. • When a country experience an increase in per capita income, the Wh i i i i i h change might stem roughly from three sources. 2. Technical progress initially benefits the (relatively) small industrial sector. Technical progress is likely to have a more uneven character at low levels of income. progress is likely to have a more uneven character at low levels of income 1. Steady sequence of annual growth. 3. Technical progress is initially biased against unskilled labor and tends to drive down these wages. these wages. 2. Uneven change 4. Industrialization brings profits to a minority that possess the financial endowments and entrepreneurial drive to take advantage of the new opportunities that open up. p g pp p p 3 3. Compensatory change Compensatory change 5. These gains ultimately find their way to everybody, as the increased demand for labor • Inverted-U: Uneven changes occur at low levels of income, whereas g , drives up wages. compensatory changes occur at higher levels of income. 5 6 Why did Kuznets suggest an inverted-U? y gg Testing the inverted U hypothesis Testing the inverted-U hypothesis Observed inequality decline in the United States between 1913 and 1948 1948. There are two ways to test the inverted-U: No data prior to the creation of the federal income tax in 1913, but the general presumption was that inequality had been rising during the nineteenth century. 1. Cross-section study: examine variations in inequalities across countries that are at different stages in the development Kuznets used the ratio of the income share of the richest 20% of the process. population to that of the poorest 60% of the population. 2. Study an individual country over time and note the resulting Kuznets (1963): changes in inequality that occurs with development. Eighteen countries Eighteen countries The income shares of upper income groups in developed countries where lower than in poorer developing countries, and highest in middle income countries. 7 8
An inverted U in the cross section? An inverted-U in the cross section? An inverted-U in the cross section? An inverted U in the cross section? Paukert (1973): P k t (1973) • The table reveals two things: 56 countries were classified into different income categories according to their per capita GDP in 1965, in U.S dollars. 1 1. Fi First, there appears to be a relationship between inequality t th t b l ti hi b t i lit and GDP of the kind predicted by Kuznets. Income category Average Gini Range of Gini Less than 100 0.419 0.33 ‐ 0.51 2. Second, that the variation within a particular category is 101 ‐ 200 0.468 0.26 ‐ 0.50 high. 201 ‐ 300 4.499 0.36 ‐ 0.62 301 ‐ 500 0.494 0.30 ‐ 0.64 501 ‐ 1000 0.438 0.38 ‐ 0.58 1001 2000 1001 ‐ 2000 0 401 0.401 0.30 ‐ 0.50 0 30 0 50 2001 and higher 0.365 0.34 ‐ 0.39 9 10 An inverted-U in the cross section? • Ahluwalia (1976) analyzed a sample of sixty countries: 40 Ahluwalia (1976) analyzed a sample of sixty countries: 40 developing, fourteen developed, and six socialist. • The population of each country is divided into quintiles. • For each quintile we have the following regression: 2 D • S A b S i =A+by i +cy i 2 +D i +error i 11 12
An inverted-U in the cross section Inverted-U Words of Caution Inverted U ”Words of Caution” • S i =A i +by i +cy i 2 +D i +error i • • The data exhibit too much variation to support some law of The data exhibit too much variation to support some law of economic change. Income share Constant y y 2 Socialist R 2 Top 20% ‐ 57.58* 89.95* ‐ 17.56* ‐ 20.15* 0.58 Middle 40% 87.03* ‐ 45.59* 9.25* 8.31* 0.47 • A regression of the form estimated in the example is not the only functional form that can deliver an inverted U shape functional form that can deliver an inverted-U shape. Lowest 20% L t 20% 27.31* 27 31* ‐ 16.97* 16 97* 3.06* 3 06* 5 54* 5.54* 0.54 0 54 • The inverted-U is, to some extent, an artifact of the statistical • For all quintiles but the highest, income shares tends to fall initially methodology that is used in inequality measurement (see next page) with a rise in per capita GNP and then rises beyond a certain point. • There seems to be an inverted-U in the cross section, or is there? 13 14 Initially all five groups have 100. Inverted-U ”Words of Caution” What happens if one after one get an adittional 100? pp g 100 • A deep problem with cross-sectional studies: Implicit assumption 90 that all countries have the same inequality-income relationship. that all countries have the same inequality income relationship 80 70 ome 60 60 Cumulative Inco • Not only are we to believe that they follow the same qualitative 50 pattern, but the same quantitative pattern as well – the income- 40 all same income inequality curve is the same curve for all countries. inequality curve is the same curve for all countries 1 with 200 30 2 with 200 3 with 200 20 4 with 200 10 10 • The opposite extreme is to say that every country is completely 0 different: one country might have one sort of curve and the other 0 10 20 30 40 50 60 70 80 90 100 might have another and there is no relationship between the two might have another, and there is no relationship between the two. Cumulative Population p 15 16
Inverted-U ”Words of Caution” Inverted U Words of Caution A “L ti A “Latin effect”? ff t”? • • Suppose that income affects inequality in the same way across Suppose that income affects inequality in the same way across • Is the inverted-U that we see in the cross section driven by the countries, so that b and c are all the same, but that some countries observation have some separate structural reason for higher or lower inequality. a) that middle-income countries have high inequality. • This is the same as saying that the curves (by country) are all b) b) or that middle-income countries are largely Latin American and or that middle income countries are largely Latin American and parallel to one another, but we allow for different intercept terms. parallel to one another, but we allow for different intercept terms. that Latin American countries have higher inequalities for other, structural reasons. 17 18 A “Latin effect”? A Latin effect ? Latin American .4 • One sensible way to check whether this makes sense is to put in a dummy variable for Latin American countries in the regression. .3 .2 .1 • Th The estimated coefficient on the dummy can then be interpreted as ti t d ffi i t th d th b i t t d 0 the “importance” as far as inequality is concerned of being Latin 0 500 1000 1500 2000 2500 y American. 95% CI % C Fitted values ineq 19 20
Recommend
More recommend