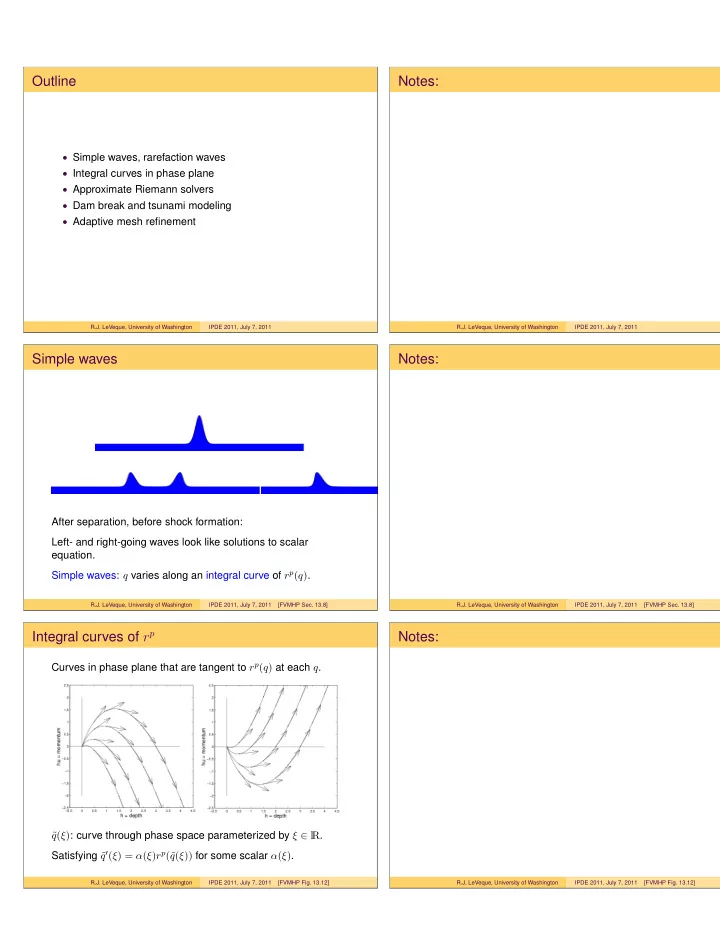

Outline Notes: • Simple waves, rarefaction waves • Integral curves in phase plane • Approximate Riemann solvers • Dam break and tsunami modeling • Adaptive mesh refinement R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 Simple waves Notes: After separation, before shock formation: Left- and right-going waves look like solutions to scalar equation. Simple waves: q varies along an integral curve of r p ( q ) . R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8] Integral curves of r p Notes: Curves in phase plane that are tangent to r p ( q ) at each q . q ( ξ ) : curve through phase space parameterized by ξ ∈ lR . ˜ q ′ ( ξ ) = α ( ξ ) r p (˜ Satisfying ˜ q ( ξ )) for some scalar α ( ξ ) . R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.12] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.12]
Integral curves of r p versus Hugoniot loci Notes: R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.7] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.7] The Riemann problem Notes: Dam break problem for shallow water equations h t + ( hu ) x = 0 hu 2 + 1 2 gh 2 � � ( hu ) t + x = 0 R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 13] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 13] 2-shock Riemann solution for shallow water Notes: Colliding with u l = − u r > 0 : Dam break: Entropy condition: Characteristics should impinge on shock: λ 1 should decrease going from q l to q m , λ 2 should increase going from q r to q m , This is satisfied along solid portions of Hugoniot loci above, not satisfied on dashed portions (entropy-violating shocks). R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.10] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.10]
Entropy-violatiing Riemann solution for dam break Notes: � Characteristic curves X ′ ( t ) = u ( X ( t ) , t ) ± gh ( X ( t ) , t ) Slope of characteristic is constant in regions where q is constant. Note that 1-characteristics do not impinge on 1-shock, 2-characteristics impinge on 2-shock. R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.11] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.11] Integral curves of r p versus Hugoniot loci Notes: Solution to Riemann problem depends on which state is q l , q r . Also need to choose correct curve from each state. R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.7] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.7] Rarefaction waves Notes: Centered rarefaction wave: Similarity solution with piecewise constant initial data: q l if x/t ≤ ξ 1 if ξ 1 ≤ x/t ≤ ξ 2 q ( x, t ) = ˜ q ( x/t ) q r if x/t ≥ ξ 2 , where q l and q r are two points on a single integral curve with λ p ( q l ) < λ p ( q r ) . Required so that characteristics spread out as time advances. Also want λ p ( q ) monotonically increasing from q l to q r . This genuine nonlinearity generalizes convexity of scalar flux. R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.5] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.5]
Genuine nonlinearity Notes: For scalar problem q t + f ( q ) x = 0 , want f ′′ ( q ) � = 0 everywhere. This implies that f ′ ( q ) is monotonically increasing or decreasing between q l and q r . Shock if decreasing, Rarefaction if increasing. For system we want λ p ( q ) to be monotonically varying along integral curve of r p ( q ) . If so then this field is genuinely nonlinear. This requires ∇ λ p ( q ) · r p ( q ) � = 0 . R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.4] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.4] Genuine nonlinearity of shallow water equations Notes: Integral curves (heavy line) and contours of λ 1 : R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.13] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Fig. 13.13] Genuine nonlinearity of shallow water equations Notes: 1-waves: Requires ∇ λ 1 ( q ) · r 1 ( q ) � = 0 . λ 1 = u − gh = q 2 /q 1 − � � gq 1 , − q 2 / ( q 1 ) 2 − 1 � � � g/q 1 ∇ λ 1 = 2 , 1 /q 1 � � 1 r 1 = , q 2 /q 1 − � gq 1 and hence ∇ λ 1 · r 1 = − 3 g/q 1 = − 3 � � g/h 2 2 < 0 for all h > 0 . R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.4] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.4]
Linearly degenerate fields Notes: Scalar advection: q t + uq x = 0 with u = constant. Characteristics X ( t ) = x 0 + ut are parallel. Discontinuity propagates along a characteristic curve. Characteristics on either side are parallel so not a shock! For system the analogous property arises if ∇ λ p ( q ) · r p ( q ) ≡ 0 holds for all q , in which case λ p is constant along each integral curve. Then p th field is said to be linearly degenerate. R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.4] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.8.4] The Riemann problem Notes: Dam break problem for shallow water equations h t + ( hu ) x = 0 hu 2 + 1 2 gh 2 � � ( hu ) t + x = 0 R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 13.12.1] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 13.12.1] Shallow water with passive tracer Notes: Let φ ( x, t ) be tracer concentration and add equation φ t + uφ x = 0 = ⇒ ( hφ ) t + ( uhφ ) x = 0 . Gives: � h � q 1 q 2 � � � hu � � � hu 2 + 1 ( q 2 ) /q 1 + 1 q = hu = q 2 , f ( q ) = 2 gh 2 = 2 g ( q 1 ) 2 . hφ q 3 uhφ q 2 q 3 /q 1 Jacobian: 0 1 0 − u 2 + gh . f ′ ( q ) = 2 u 0 − uφ φ u R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.12.1] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.12.1]
Shallow water with passive tracer Notes: � 0 1 0 � − u 2 + gh f ′ ( q ) = 2 u 0 . − uφ φ u λ 1 = u − √ gh, λ 3 = u + √ gh, λ 2 = u, � 0 1 1 � � � � u − √ gh � u + √ gh r 1 = r 2 = r 3 = 0 , , . 1 φ φ − u/h λ 2 = u = ( hu ) /h = ⇒ ∇ λ 2 = ⇒ λ 2 · r 2 ≡ 0 . = 1 /h 0 So 2nd field is linearly degenerate. (Fields 1 and 3 are genuinely nonlinear.) R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.12.1] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Sec. 13.12.1] Euler equations of gas dynamics Notes: Conservation of mass, momentum, energy: q t + f ( q ) x = 0 with ρ ρu ρu 2 + p , q = ρu f ( q ) = E u ( E + p ) where p = pressure = p ( ρ, E ) (Equation of state) The Jacobian f ′ ( q ) has eigenvalues u − c, u, u + c where � dp c = dρ at constant entropy Eigenvalues vary with q = ⇒ shocks, rarefactions. R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 14] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 14] Riemann Problem for Euler equations Notes: Initial data: � q l if x < 0 q ( x, 0) = q r if x > 0 Shock tube problem: u l = u r = 0 , jump in ρ and p . Pressure: This is also solution to dam break problem for shallow water equations. R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 14] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 14]
Riemann Problem for gas dynamics Notes: Waves propagating in x – t space: Similarity solution (function of x/t alone). Waves can be approximated by discontinuties: Approximate Riemann solvers R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 14] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [FVMHP Chap. 14] Approximate Riemann Solvers Notes: Approximate true Riemann solution by set of waves consisting of finite jumps propagating at constant speeds. Local linearization: Replace q t + f ( q ) x = 0 by q t + ˆ Aq x = 0 , where ˆ A = ˆ A ( q l , q r ) ≈ f ′ ( q ave ) . Then decompose r 1 + · · · α m ˆ q r − q l = α 1 ˆ r m to obtain waves W p = α p ˆ r p with speeds s p = ˆ λ p . R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [Sec. 15.3] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [Sec. 15.3] Approximate Riemann Solvers Notes: How to use? One approach: determine Q ∗ = state along x/t = 0 , Q ∗ = Q i − 1 + � W p , F i − 1 / 2 = f ( Q ∗ ) , p : s p < 0 A + ∆ Q = f ( Q i ) − F i − 1 / 2 . A − ∆ Q = F i − 1 / 2 − f ( Q i − 1 ) , Or, sometimes can use: � s p W p , A + ∆ Q = � s p W p . A − ∆ Q = p : s p < 0 p : s p > 0 Conservative only if A − ∆ Q + A + ∆ Q = f ( Q i ) − f ( Q i − 1 ) . This holds for Roe solver. R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [Sec. 15.3] R.J. LeVeque, University of Washington IPDE 2011, July 7, 2011 [Sec. 15.3]
Recommend
More recommend