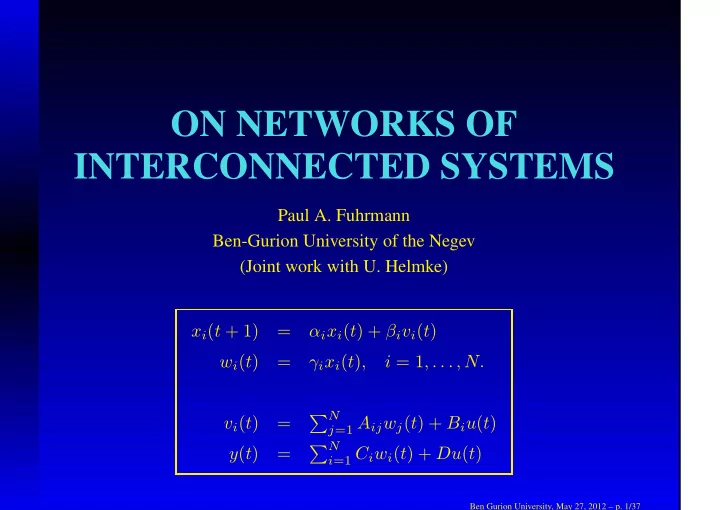
ON NETWORKS OF INTERCONNECTED SYSTEMS Paul A. Fuhrmann Ben-Gurion - PowerPoint PPT Presentation

ON NETWORKS OF INTERCONNECTED SYSTEMS Paul A. Fuhrmann Ben-Gurion University of the Negev (Joint work with U. Helmke) x i ( t + 1) = α i x i ( t ) + β i v i ( t ) w i ( t ) = γ i x i ( t ) , i = 1 , . . . , N. � N v i ( t ) = j =1 A ij w j ( t ) + B i u ( t ) � N y ( t ) = i =1 C i w i ( t ) + Du ( t ) Ben Gurion University, May 27, 2012 – p. 1/37

MOTIVATION • Study controllability and observability properties of networks of, homogeneous or nonhomogeneous, node linear systems. • Allow flexibility in the nature (discrete time, continuous time) and representation (first order, high order) of both the node systems as well as the interconnections (constant, dynamic). • Checking controllability by use of the Kalman controllability and observability matrices is impractical. These we replace by compact coprimeness conditions. Ben Gurion University, May 27, 2012 – p. 2/37

EXAMPLES • Formation Control of Autonomous Distributed Systems. (Common Dynamics), Flocks of Birds. • Traffic Control; Truck Convoys. • (Interconnecting) Biological Systems; Cicadas. • Synchronization Phenomena; Ciliary Beating. • Control of Electrical Networks (Grids). Ben Gurion University, May 27, 2012 – p. 3/37

DIFFICULTIES • Linearity is too restrictive. • (Ballistic) Controllability may not be the correct concept. • Study self organizing systems (organizations). A variational approach? What do we optimize? Efficiency? What is it? Ben Gurion University, May 27, 2012 – p. 4/37

REFERENCES P.A. Fuhrmann and U. Helmke, ”Strict equivalence, controllability and observability of networks of linear systems”, submitted to Mathematics of Control, Signals and Systems . P.A. Fuhrmann, “On strict system equivalence and similarity”, Int. J. Contr. , 25, 5-10, (1977). H.H. Rosenbrock and A.C. Pugh, “Contributions to a hierarchical theory of systems”, Int. J. Contr. , 19, 845-867, (1974). F.M. Callier, and C.D. Nahum, “Necessary and sufficient conditions for the complete controllability and observability of systems in series using the coprime decomposition of a rational matrix”, IEEE Trans. Circuits and System , Ben Gurion University, May 27, 2012 – p. 5/37

FROM POLYNOMIALS TO MODEL SPACES Polynomial Arithmetic: D ( z ) ∈ F [ z ] m × m , det D ( z ) � = 0 Geometry: Polynomial Model: π D : F [ z ] m −  → F [ z ] m   π D f = Dπ − D − 1 f X D = Im π D ≃ F [ z ] m /D ( z ) F [ z ] m   Rational Model: D ( σ ) : z − 1 F [[ z − 1 ]] m −  → z − 1 F [[ z − 1 ]] m   D ( σ ) h = π − Dh, π D h = π − D − 1 π + Dh Ker D ( σ ) = Im π D = X D ≃ X D   Ben Gurion University, May 27, 2012 – p. 6/37

FACTORIZATIONS AND INVARIANT SUBSPACES D ( z ) ∈ F [ z ] m × m nonsingular. V ⊂ X D & S D V ⊂ V ⇔ V = D 1 X D 2 V ⊂ X D & S D V ⊂ V ⇔ V = X D 2 D ( z ) = D 1 ( z ) D 2 ( z ) , D 1 ( z ) , D 2 ( z ) ∈ F [ z ] m × m CONNECTION BETWEEN ALGEBRA AND GEOMETRY DIRECT LINK TO GEOMETRIC CONTROL AND BEHAVIORS Ben Gurion University, May 27, 2012 – p. 7/37

MODEL HOMOMORPHISMS Let D 1 ( z ) ∈ F [ z ] m × m and D 2 ( z ) ∈ F [ z ] p × p be nonsingular. S D = σ | X D Z : X D 1 − → X D 2 is an F [ z ] -homomorphism, i.e. ZS D 1 = S D 2 Z if and only if there exist N 1 ( z ) , N 2 ( z ) ∈ F [ z ] p × m such that N 2 ( z ) D 1 ( z ) = D 2 ( z ) N 1 ( z ) Zh = π − N 1 h = N 1 ( σ ) h. INVERTIBILITY AND COPRIMENESS Ben Gurion University, May 27, 2012 – p. 8/37

THE SHIFT REALIZATION    A B G ( z ) = V ( z ) T ( z ) − 1 U ( z ) + W ( z ) =  C D  A = S T   Bξ = π T Uξ,  Cf = ( V T − 1 f ) − 1   D = G ( ∞ ) .  CA j − 1 Bξ = ( V T − 1 π T z j − 1 π T Uξ ) − 1 = ( V π − T − 1 z j − 1 Uξ ) − 1 = ( z j − 1 ( V T − 1 U + W ) ξ ) − 1 Realization is reachable ⇔ T ( z ) and U ( z ) left coprime. Realization is observable ⇔ T ( z ) and V ( z ) right coprime. Ben Gurion University, May 27, 2012 – p. 9/37

SYSTEM EQUIVALENCE Rosenbrock, Fuhrmann G ( z ) = V i ( z ) T i ( z ) − 1 U i ( z ) + W i ( z ) , i = 1 , 2 (no coprimeness assumptions); Σ i the associated shift realizations � T i ( z ) − U i ( z ) � P i = V i ( z ) W i ( z ) P 1 ≃ FSE P 2 if Σ 1 ≃ Σ 2 P 1 ≃ FSE P 2 ⇔ ∃ M ( z ) , X ( z ) , N ( z ) , Y ( z ) , such that M ( z ) ∧ L T 2 ( z ) = I, & N ( z ) ∧ R T 1 ( z ) = I          M ( z ) 0  T 1 ( z ) − U 1 ( z )  T 2 ( z ) − U 2 ( z )  N ( z ) Y ( z )  =    X ( z ) I V 1 ( z ) W 1 ( z ) V 2 ( z ) W 2 ( z ) 0 I Ben Gurion University, May 27, 2012 – p. 10/37

NODE REPRESENTATIONS G i ( z ) = γ i ( zI − α i ) − 1 β i = Q i ( z ) − 1 P i ( z ) = P i ( z ) Q i ( z ) − 1 = V i ( z ) T i ( z ) − 1 U i ( z ) + W i ( z ) . Assume all representations are minimal, i.e., δ ( G i ) = deg det Q i = deg det Q i = deg det T i . This is equivalent to the controllability and observability of the shift realizations associated with these representations, i.e., to the left coprimeness of Q i ( z ) , P i ( z ) , the right coprimeness of P i ( z ) , Q i ( z ) , the left coprimeness of T i ( z ) , U i ( z ) and the right coprimeness of T i ( z ) , V i ( z ) . Ben Gurion University, May 27, 2012 – p. 11/37

INTERCONNECION MODEL x i ( t + 1) = α i x i ( t ) + β i v i ( t ) w i ( t ) = γ i x i ( t ) , i = 1 , . . . , N. v i ( t ) = � N j =1 A ij w j ( t ) + B i u ( t ) y ( t ) = � N i =1 C i w i ( t ) + Du ( t ) x ( t + 1) = αx ( t ) + βv ( t ) w ( t ) = γx ( t ) v ( t ) = Aw ( t ) + Bu ( t ) y ( t ) = Cw ( t ) + Du ( t ) x ( t + 1) = A x ( t ) + B u ( t ) y ( t ) = C x ( t ) + D u ( t ) A := α + βAγ, B := βB, C := Cγ, D := D. Ben Gurion University, May 27, 2012 – p. 12/37

Node transfer function G ( z ) := diag ( G 1 ( z ) , . . . , G N ( z )) = γ ( zI − α ) − 1 β. Interconnection transfer function ϕ ( z ) = C ( zI − A ) − 1 B + D. Global network transfer function Φ( z ) = C ( zI − A ) − 1 B + D ; = Cγ ( zI − α − βAγ ) − 1 βB + D. Ben Gurion University, May 27, 2012 – p. 13/37

MATRIX FRACTION DESCRIPTIONS (MFD) Q i ( σ ) ξ i = P i ( σ ) v i w i = ξ i v i ( t ) = � N j =1 A ij w j ( t ) + B i u ( t ) y ( t ) = � N i =1 C i w i ( t ) + Du ( t ) Φ( z ) = C ( Q ( z ) − P ( z ) A ) − 1 P ( z ) B + D. Here Q ( z ) = diag ( Q 1 ( z ) , . . . , Q N ( z )) , etc. Ben Gurion University, May 27, 2012 – p. 14/37

POLYNOMIAL MATRIX DESCRIPTION (PMD) T i ( σ ) ξ i = U i ( σ ) v i w i = V i ( σ ) ξ i + W i ( σ ) v i v i ( t ) = � N j =1 A ij w j ( t ) + B i u ( t ) y ( t ) = � N i =1 C i w i ( t ) + Du ( t ) For the case W ( z ) = 0 : Φ( z ) = CV ( z )( T ( z ) − U ( z ) AV ( z )) − 1 U ( z ) B + D. Here T ( z ) = diag ( T 1 ( z ) , . . . , T N ( z )) , etc. Ben Gurion University, May 27, 2012 – p. 15/37

EQUIVALENCE UNDER INTERCONNECTION Given N FSE node systems in PMD form � � T ( ν ) − U ( ν ) ( z ) ( z ) i i , ν = 1 , 2 ; i = 1 , . . . , N . V ( ν ) W ( ν ) ( z ) ( z ) i i � E ( σ ) v = A ( σ ) w + B ( σ ) u Dynamic interconnection: = C ( σ ) w + Du. y     T (1) − U (1) T (2) − U (2) 0 0 0 0     V (1) W (1) V (2) W (2) − I 0 − I 0         ≃ F SE     0 E − A − B 0 E − A − B         0 0 0 0 C D C D Ben Gurion University, May 27, 2012 – p. 16/37

STANDARD INTERCONNECTIONS • SERIES CONNECTION � � � � 0 0 I � � A = , B = , C = 0 I I 0 0 • PARALLEL CONNECTION � � � � 0 0 I � � A = , B = , C = I I 0 0 I • FEEDBACK CONNECTION � � � � 0 I I � � A = , B = , C = I 0 I 0 0 Ben Gurion University, May 27, 2012 – p. 17/37

SERIES CONNECTION Given systems Σ i , i = 1 , 2 , with transfer functions � A i B i � G i ( z ) = . C i D i G 1 ( z ) ∈ F ( z ) k × m , G 2 ( z ) ∈ F ( z ) p × k . The series coupling Σ 1 ∧ Σ 2 , is defined by:  x (1) A 1 x (1) = + B 1 u t t +1 t    C 1 x (1)  y ′ = + D 1 u t  t t   x (2) A 2 x (2) = + B 2 u ′ t +1 t t  C 2 x (2) y t = + D 2 u ′   t t    u ′ = y ′ t .  t Ben Gurion University, May 27, 2012 – p. 18/37

Schematically, we have the following diagram: u y ✲ ✲ ✲ G 1 G 2 � A 2 B 2 � A 1 B 1 � � G 2 ( z ) G 1 ( z ) = × C 2 D 2 C 1 D 1  A 1 0 B 1   . B 2 C 1 A 2 B 2 D 1 =  D 2 C 1 C 1 D 2 D 1 Controllability and observability are difficult to deduce from this representation. Ben Gurion University, May 27, 2012 – p. 19/37

SERIES CONNECTION AND COPRIMENESS • Let G 1 ( z ) ∈ F ( z ) k × m , G 2 ( z ) ∈ F ( z ) p × k have the coprime matrix fraction representations G 1 ( z ) = D L 1 ( z ) − 1 N L 1 ( z ) = N R 1 ( z ) D R 1 ( z ) − 1 G 2 ( z ) = D L 2 ( z ) − 1 N L 2 ( z ) = N R 2 ( z ) D R 2 ( z ) − 1 Then δ ( G 2 G 1 ) ≤ δ ( G 1 ) + δ ( G 2 ) . • The series coupling of the shift realizations associated with N R 2 ( z ) D R 2 ( z ) − 1 and N R 1 ( z ) D R 1 ( z ) − 1 is controllable if and only if N R 1 ( z ) and D R 2 ( z ) are left coprime. Ben Gurion University, May 27, 2012 – p. 20/37