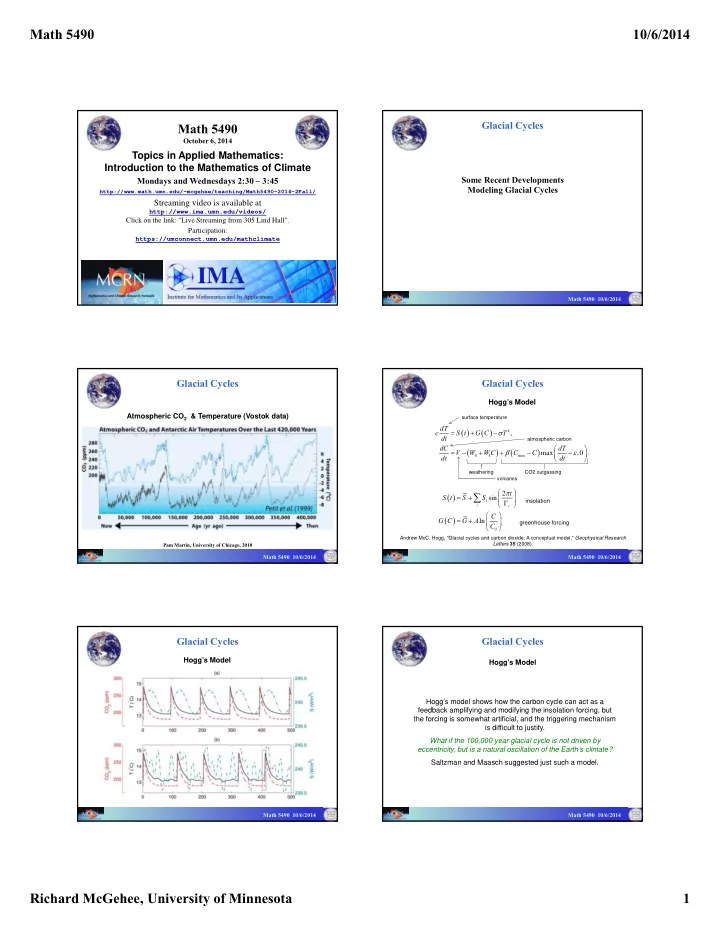
Math 5490 10/6/2014 Glacial Cycles Math 5490 October 6, 2014 - PDF document

Math 5490 10/6/2014 Glacial Cycles Math 5490 October 6, 2014 Topics in Applied Mathematics: Introduction to the Mathematics of Climate Some Recent Developments Mondays and Wednesdays 2:30 – 3:45 Modeling Glacial Cycles http://www.math.umn.edu/~mcgehee/teaching/Math5490-2014-2Fall/ Streaming video is available at http://www.ima.umn.edu/videos/ Click on the link: "Live Streaming from 305 Lind Hall". Participation: https://umconnect.umn.edu/mathclimate Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Hogg’s Model Atmospheric CO 2 & Temperature (Vostok data) surface temperature dT         c S t G C T 4 , dt atmospheric carbon dC  dT              V W W C C C max  ,0 .  0 1 max dt  dt  weathering CO2 outgassing volcanos    t 2      S t S S sin   i  insolation   i i   C     G C G A   ln greenhouse forcing C   0 Andrew McC. Hogg, "Glacial cycles and carbon dioxide: A conceptual model," Geophysical Research Pam Martin, University of Chicago, 2010 Letters 35 (2008). Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Hogg’s Model Hogg’s Model Hogg’s model shows how the carbon cycle can act as a feedback amplifying and modifying the insolation forcing, but the forcing is somewhat artificial, and the triggering mechanism is difficult to justify. What if the 100,000 year glacial cycle is not driven by eccentricity, but is a natural oscillation of the Earth’s climate? Saltzman and Maasch suggested just such a model. Math 5490 10/6/2014 Math 5490 10/6/2014 Richard McGehee, University of Minnesota 1

Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Salzman-Maasch Model Salzman-Maasch Model unforced Milankovitch forcing    X   X  Y  uM t global ice mass  Y   pZ  rY  sZ 2  Z Y 2 atmospheric CO 2    Z   q X  Z ocean circulation Barry Salzman and Kirk A. Maasch, "A Low-Order Dynamical Model of Global Climatic Variability Over the Full Pleistocene," Journal of Geophysical Research 95 (D2), 1955-1963 (1990) Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Salzman-Maasch Model Salzman-Maasch Model forced The Salzman-Maasch model shows how the carbon cycle and the ocean currents can interact to produce unforced oscillations with periods of about 100,000 years. The same model with slightly different parameters can exhibit stationary behavior. By forcing the model with Milankovitch cycles and by slowly varying the parameters over the last two million years, they can produce a bifurcation from small oscillations tracking the Milankovitch cycles to large oscillations with a dominant 100,000 year period. Seems like a nice idea (especially by mathematicians, since it looks like a “Hopf Bifurcation”,) but it is not widely accepted as the explanation, and it has some problems. Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Huybers’ Analysis of Deglaciations Salzman-Maasch Model Issue of Circular Reasoning Data sets (stacks of data from individual sediment cores) are usually The bifurcation explanation seems to have two serious problems (“cosmic coincidences”). “orbitally tuned”, i.e., the “age model” is adjusted so the cycles in the data line up with Milankovitch cycles. 1. Why does the intrinsic period of the glacial cycles Using tuned data sets to conclude that Milankovitch theory is valid is just happen to have the same period as the circular reasoning. eccentricity cycles? Huybers rederived the “age model” for a Pleistocene data set 2. Why does the phase of the glacial cycles agree without using orbital tuning. with the phase of the obliquity and eccentricity He concluded that the deglaciations are triggered by obliquity. cycles? Samantha Oestreicher, PhD Thesis, 2014. Peter Huybers, "Glacial variability over the last two million years: an extended depth-derived age model, continuous obliquity pacing, and the Pleistocene progression," Quaternary Science Reviews 26 , 37-55 (2007). Math 5490 10/6/2014 Math 5490 10/6/2014 Richard McGehee, University of Minnesota 2

Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Huybers’ Analysis of Deglaciations Huybers’ Analysis 18 O data are usually “orbitally tuned,” i.e. , the age model is partially determined by Milankovitch cycles. Huybers reworked the data using only geomagnetic markers, 18 O events, and depth. Vertical black lines: Geomagnetic events Red dots: deglaciations. Red dots: 18O events What are the horizontal red bars? Yellow bars: Obliquity cycle skipped Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Huybers’ Analysis Huybers’ Analysis Huyber’s age model agrees well with other age models, but contains no bias introduced by “orbital tuning”. The ages for geomagnetic events are uncertain. Consequently, the 18 O events are uncertain, and the entire age model is uncertain. Black: Huyber stack Red: Lisiecki and Raymo stack Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Huybers’ Model of Deglaciations Huybers’ Model V t : ice volume at time  V    V  T t if   t t t V 1 T : threshold variable t  t V T  0 if t t  : rate of increase of ice volume      T at b c   t t : normalized obliquity t Units and constants t : Kyr V : chosen so that η = 1. θ ’ : mean zero and variance one a = 0.05 b = 126 c = 20 Math 5490 10/6/2014 Math 5490 10/6/2014 Richard McGehee, University of Minnesota 3

Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Huybers’ Model Huybers’ 2011 Analysis of Deglaciations The deglaciations are triggered by the following forcing function. Huybers’ model produces the decline in temperature and the F   e        increase in period and amplitude of the glacial cycles, but it 1 2 1 2 sin( ) (1 ) t t t t depends heavily on an unspecified decline in the sensitivity of the triggering mechanism over last two million years. where e  eccentricity t   precession angle t Revised in 2011.   obliquity t   and are parameters. Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Huybers’ 2011 Analysis of Deglaciations Huybers’ 2011 Model      V V T V t if : ice volume at time V   t 1 t t t t  V T T  0 if : threshold variable t t t    T F 110 25 : rate of increase of ice volume t t F   e        1 2 1 2 sin( ) (1 ) t t t t black = climate data grey = F t Peter Huybers, Combined obliquity and precession pacing of late Pleistocene deglaciations, red = simulation N ATURE 480 (2011), 229-232 Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Abe-Ouchi et al Ice Sheet Model Questions The larger the ice sheet, the more unstable it becomes, and the more sensitive it is to insolation. Once it begins to retreat, 1. Did eccentricity play any role during the last million years? feedbacks cause a rapid pace. Is the apparent 100 kyr cycle an artifact (Huybers)? Is it an intrinsic cycle in the climate system that coincidentally has Ayako Abe-Ouchi, Fuyuki Saito, Kenji Kawamura, Maureen E. a period of 100,000 years (Maasch and Saltzman)? Raymo, Jun’ichi Okuno, Kunio Takahashi & Heinz Blatter, 2. Is the CO 2 feedback sufficient to explain the increasing amplitude “Insolation-driven 100,000-year glacial cycles and hysteresis of ice- and period of the glacial cycles during the last million years, i.e. , is sheet volume,” Nature 500 (2013), 190-193. doi:10.1038/nature12374 it the mechanism behind the Huybers model. 3. Where does the atmospheric CO 2 go during the glacial maxima? Animation available on Nature Web site: The ocean? The land? 4. What will be the effect of the anthropogenic CO 2 ? http://www.nature.com/nature/journal/v500/n7461/full/nature12374.html#videos Math 5490 10/6/2014 Math 5490 10/6/2014 Richard McGehee, University of Minnesota 4

Math 5490 10/6/2014 Glacial Cycles Glacial Cycles Atmospheric CO 2 & Temperature (Vostok data) Vostok Data 12 10 Current 8 conditions 6 are well 4 outside the δ T ( ° C) 2 range 0 recorded in ‐ 2 the ice core ‐ 4 ‐ 6 data. ‐ 8 ‐ 10 150 200 250 300 350 400 450 CO2 (ppm) Pam Martin, University of Chicago, 2010 Math 5490 10/6/2014 Math 5490 10/6/2014 Glacial Cycles Isotopes as Climate Proxies Vostok Data 12 10 8 6 Extrapolate 4 linear δ T ( ° C) 2 regression to 0 400 ppm ‐ 2 CO2. ‐ 4 ‐ 6 How do we know the past climates? ‐ 8 ‐ 10 150 200 250 300 350 400 450 CO2 (ppm) Math 5490 10/6/2014 Math 5490 10/6/2014 Isotopes as Proxies Isotopes as Proxies What is this? δ 18 O (‰) Ocean Sediment Cores Hansen, et al, Target atmospheric CO2: Where should humanity aim? Open Atmos. Sci. J . 2 (2008) http://eo.ucar.edu/staff/rrussell/climate/paleoclimate/sediment_proxy_records.html Math 5490 10/6/2014 Math 5490 10/6/2014 Richard McGehee, University of Minnesota 5