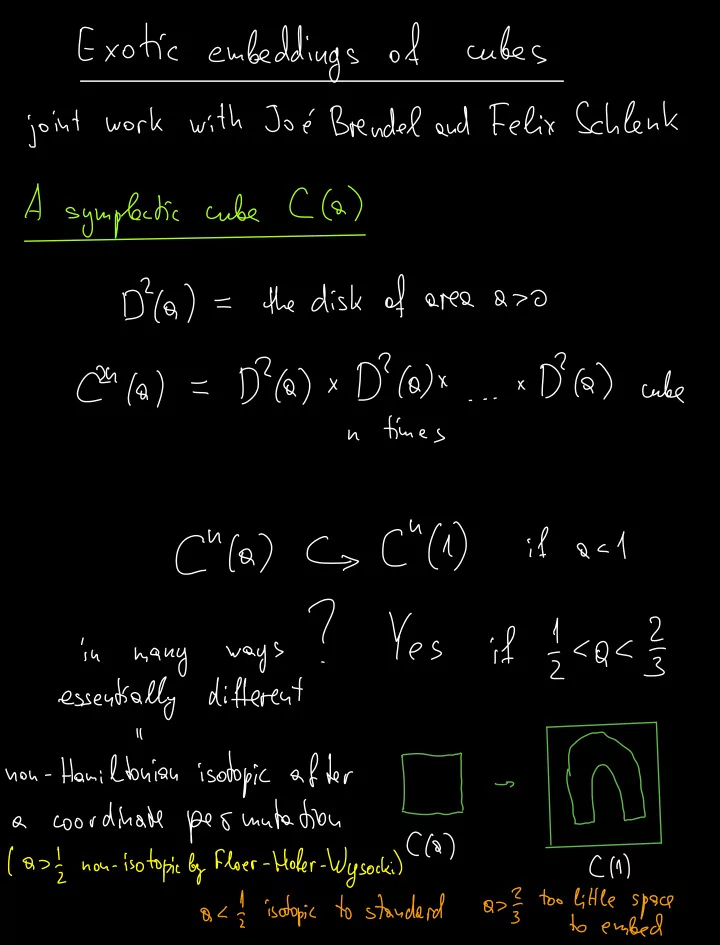

Exotic embeddings of cubes joint work with Joe Brendel and Felix Schlent A symplectic cube C a the disk of a a are D a D D D a a cube a a n times il act 1 a Yes il in cas ways many different essentially Il LD on Hamiltonian isotopic after coordinate permutation A non isotopic by fluer Hoher Wysocki a 1 too little space isotopic to standard as as to embed
There results further previous some are isotopic embeddings by ou non Dimitrogler Rizell Gutt Usher and Hind Theorem Brendel Schleck M 1 a et non isotopic embeddings Et when grows arbitrarily large a two least embeddings at cas un least three embeddings ca qt 1,1 3
A same theorem for version et the 1341 of B la na isotopie embeddings jpp psy when DU grows arbitrarily large f 1 a f Ça at least did one at least two 11,2 cas at least three 45,2 cas
Furlermore for similar theorem a embedding Del to monotone closed Dezzo surfaces P In il the particular degree then 9,8 6,5 is gnous arbitrarily large for a f end KE g a 1 P.is her QPxept KF8 a does not admit 2K Pialttiangular degenendons 1 KE g Q Clip blown up 3 times f6 l r Clip blown up 4 lines r K b Q q less well organized embeddings probably also with from degeneration to Pic 1 tone surfaces their number for other K growing arbitrarily large blown up 1,2 5,47 and 8 i times e
Markov type Markov's and equations tonic their and geometry Markov 1879 642 1N 3 abc d 0,6 ce GR such GB c of numerology called Markov's triple Hacking Prokhorov au earlier work of Manetti advancing 2010 projective tone surfaces with classified Piet and T only having singularities aha Wahl sinoothable tonic singularities Q Gorenstein snoothoble that and noticed they are vanishing local to global obstructions globally Pld f R lake isa Markov triple where Answery clin 11,421,145,2 more constructive proofs are also available log other now
why to car A is smoothing family a projective 3 foff X CA Xt X t tt smooth ff singulart Dat unit cl disk c D 0 Xt Xtz symplecto norphisa 7 characteristic foliation in ê µ Ë 0 iÏü t.net gag Ï ff 24
symplectomorphic Ifk x Xt Xi X Elk t p Smooth locus d singular part locus vanishing cycles Anything embedded to the smooth locus of to transported to Xt gets symplecticolly idea et using The Markov triples to geometry of of EP study symplectic back 2010 pm print to IPMU goes Gallus and Usnich of
Calkin and Usuich Markov triples have upgraded triangle t.eu CIR to Markov lathe ÇA c tric dans of Playa i corresponding to and introduced their mutations a 6,308 c 0,6 c Markov triangle étoki g sobe a geometric representation of d Markov's equation 10,07 10,0 C ad Zleugtha Each cone op Pp I is singularity a Zheights of Milnor number O V à normalized area length height
In la l a I i Pat 4 PU 1,17AM là pr 9211 Convex Hull ÆË Tap Using tonic fan mutations corresponding to Markov's G U have introduced mutations Ca b c Ca bisab c points d Ta functions on the lattice integer an Conjecture G U 2010 this function is function for counting Maslow 2 disk the some corresponding ton Lagrangian Ipl C a b c Vianna Pascalelf Touleonog by proved Dimitroglu Rizell Ekholm Tanking et al
Let from smoothing construct ça directly us Galvin M of Plait d unpublished Pca by is Symplecticolly given MY lattice dual triangle OqqcN.IR N a Ss R C Mor Re R to Tap ÏÏ i G U cf almost tonimutadia and dual matelas mutations of Casals Vianna in this consider polygon we bissectrices intersect integer they is not it in Note generally point one of Das the barycenter
ctntediteda The fiber j j.li over is monotone a Lagrangian tonus contained locus in the Smooth CNNRNR das d Plan t Yin
il then c 1 Similarly à Ebasis µ f2 Top I 1 Healy singular points the gives Etat hypotenuse removing Phi MD locus of the Smooth in 1PM 1,1 embeds to Eté of volume are area et K Sabc the hypotenuse taken with multiplicity ab the huit d a family d lires is is À he approximating l sharp Blab embeds to Edf embedding Eco A dË Ü3 embeds to BH d Elf or Casals Vianna A la.by is
Markov triple But also Ela cubes in are ab ab aÆÆ faxe Hab a a J C bissectrice bla BY SILLÉ BU c Markov triples Ca 6,1 for dilterait consecutive odd Fibonacci doubles la Hamiltonian isotopic are not Ca d Cada spifsizt L.me since already the restrictions to pifs not be Hamiltonian isotopic can The argument D survives stabilization by BY of other monotone nlds coulanty cubes taking products with
We Cia Bill finish case by consideration d the representation of the golden sedan following Kc r golden section ellipsoid F ç µ f r z s s the f f is integer center of inscribed circle even continuous Et le n'bed ÉË L bissectrice intersection point t
Cubes be traded sources can for other domains in about targets How the Del Pezzo surface et is but 9 possible degree highest smaller there DPs degree are and Markov type have their own they equations NARTIR je battu triangle a perimeter M X cap A gives area Kahler bric variety
A iw.K Mwettlxkizdkc w HYXOI.IQ Il 1 1 work A proportional formula Noether's 3 12 m K Et Milnor numbers et cornes l May have I KK 9 for singularities M méthylène A main.ua dc2 Markov equations type maêtnebkhei Vit TAI Kaneohe QUEL
ma à urgé abc tn c m.mn 12 na n m l must be integer Prokhorov Hacking 14 Marteau type equations But 4 of them only 04284F Gabe SAEKI E Coloc CE sale Q2 l 507f E Sale solutions with less then admit three I e 1 e un Singularities 442f42 CE fabe The other to do not 604267 E Gabe À 4172Eme 3 i 382 3è 9 be 604384 E Gabe 90464CE 3abe f4 f 8 à Gabe 8 à 284 E E 5alx.ba 3b72c2 Gabc 5045f Gabe
EH EH 2 phaetot audited Mit Papa Être ils complement is Elf 2
Recommend
More recommend