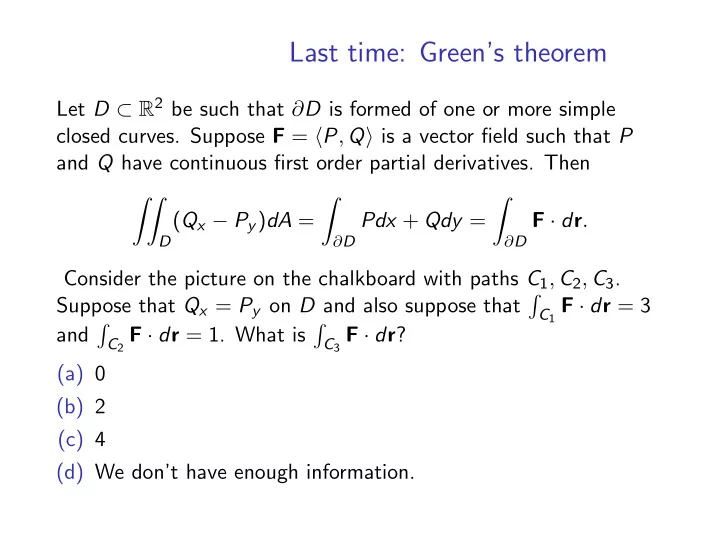
Last time: Greens theorem Let D R 2 be such that D is formed of one - PowerPoint PPT Presentation

Last time: Green’s theorem Let D ⊂ R 2 be such that ∂ D is formed of one or more simple closed curves. Suppose F = ⟨ P , Q ⟩ is a vector field such that P and Q have continuous first order partial derivatives. Then ∫︂∫︂ ∫︂ ∫︂ ( Q x − P y ) dA = Pdx + Qdy = F · d r . D ∂ D ∂ D Consider the picture on the chalkboard with paths C 1 , C 2 , C 3 . ∫︁ Suppose that Q x = P y on D and also suppose that C 1 F · d r = 3 and ∫︁ C 2 F · d r = 1. What is ∫︁ C 3 F · d r ? (a) 0 (b) 2 (c) 4 (d) We don’t have enough information.

Solution Fill in the space surrounded by the curves C 1 , C 2 , C 3 to get a region D with boundary ∂ D = ( − C 1 ) ∪ ( − C 2 ) ∪ C 3 . So we see that ∫︂ ∫︂ ∫︂ ∫︂ F · d r = − F · d r − F · d r + F · d r . ∂ D C 1 C 2 C 3 On the other hand, by Green’s theorem, ∫︂ ∫︂∫︂ F · d r = Q x − P y dA , ∂ D D but since P y = Q x this is zero. Combining, we see that ∫︂ ∫︂ ∫︂ ∫︂ 0 = − F · d r − F · d r + F · d r = − 3 − 1 + F · d r , C 1 C 2 C 3 C 3 ∫︁ so C 3 F · d r = 4.

Practice with curl Find curl ⟨ e x , z cos y , sin y ⟩ . How many of the three components are 0? (a) 0 (b) 1 (c) 2 (d) 3 (e) I don’t know. curl ⟨ e x , z cos y , sin y ⟩ = ⟨ cos y − cos y , 0 − 0 , 0 − 0 ⟩ = ⟨ 0 , 0 , 0 ⟩

Practice with curl Find curl ⟨ P ( x , y ) , Q ( x , y ) , 0 ⟩ . Solution curl ⟨ P ( x , y ) , Q ( x , y ) , 0 ⟩ = ⟨ ∂ 0 ∂ y − ∂ Q ∂ z , ∂ P ∂ z − ∂ 0 ∂ x , ∂ Q ∂ x − ∂ P ∂ y ⟩ = ⟨ 0 , 0 , Q x − P y ⟩ .

Practice with conservative vector fields and curl Is F ( x , y , z ) = ⟨ xz , xyz , − y 2 ⟩ conservative? Is G ( x , y , z ) = ⟨ e x , z cos y , sin y ⟩ conservative? (a) Yes and yes. (b) Yes and no. (c) No and yes. (d) No and no. (e) Not enough information. curl F ̸ = 0 , so F is not conservative. curl G = 0 on all of R 3 , so G is conservative.