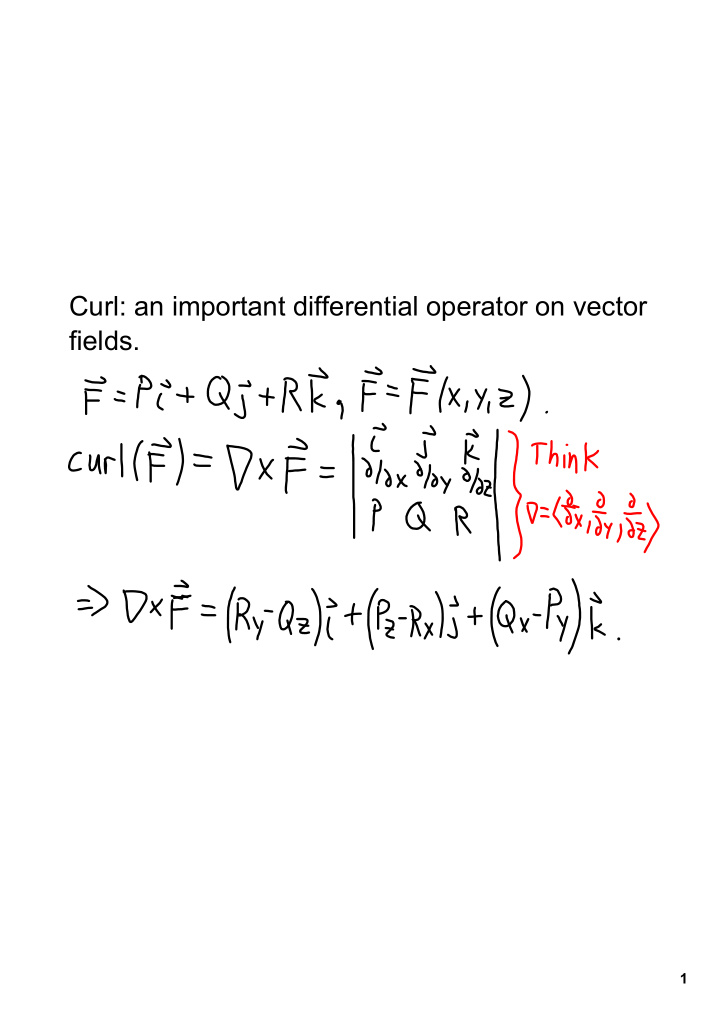



Curl: an important differential operator on vector fields. 1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
Green's Theorem in vector form 18
* Green's Theorem relates the integral of the tangential part of the field along C to the integral of the vertical part of the curl over * We will see this come up again with Stoke's Theorem later in the course. * We now come up with a similar identity involving the normal component of the field, integrated along the curve C . 19
20
21
22
23
24
Parametric Surfaces 25
26
27
Rotational surfaces 28
"Natural" parameterizations 29
Surface area: We showed previously when discussing surface area that 30
31
32
33
Practice! 34
35
36
37
38
39
40
41
Recommend
More recommend