Injectivity hyperfiniteness Connes 3 ingredients A (separably - PDF document

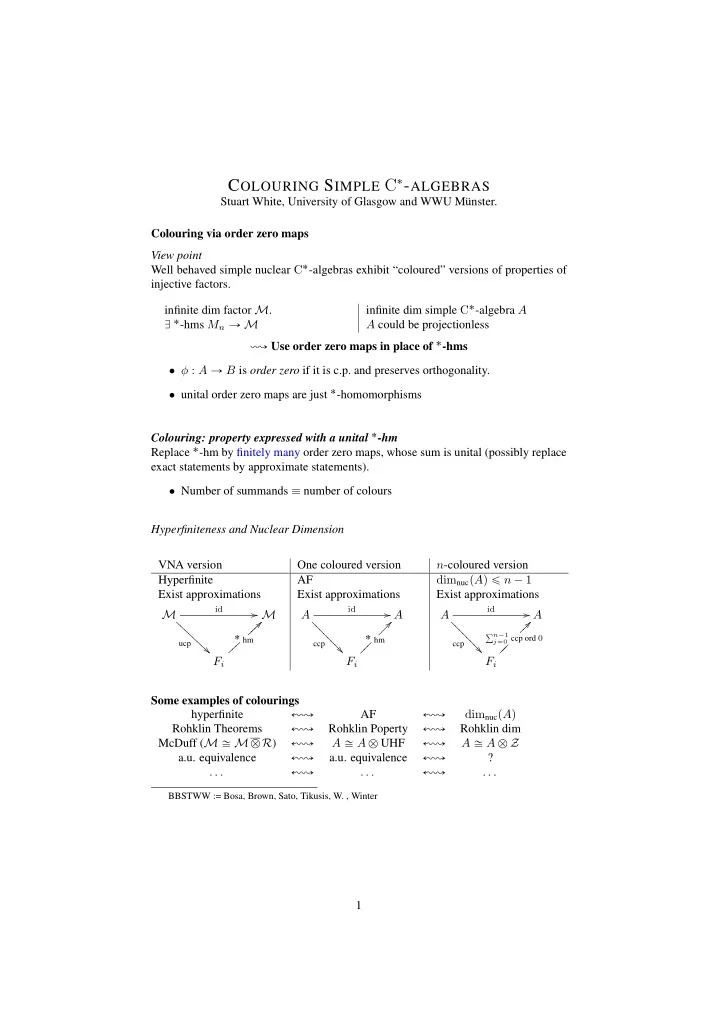
� � � � � � C OLOURING S IMPLE C ˚ - ALGEBRAS Stuart White, University of Glasgow and WWU Münster. Colouring via order zero maps View point Well behaved simple nuclear C ˚ -algebras exhibit “coloured” versions of properties of injective factors. infinite dim simple C ˚ -algebra A infinite dim factor M . D ˚ -hms M n Ñ M A could be projectionless ù Use order zero maps in place of ˚ -hms • φ : A Ñ B is order zero if it is c.p. and preserves orthogonality. • unital order zero maps are just ˚ -homomorphisms Colouring: property expressed with a unital ˚ -hm Replace ˚ -hm by finitely many order zero maps, whose sum is unital (possibly replace exact statements by approximate statements). • Number of summands ” number of colours Hyperfiniteness and Nuclear Dimension n -coloured version VNA version One coloured version dim nuc p A q ď n ´ 1 Hyperfinite AF Exist approximations Exist approximations Exist approximations id id id � M � A � A M A A ❆ ❄ ❄ ❆ ❄ ❄ ⑥ ⑧ ⑧ ❆ ⑥ ❄ ❄ ⑧ ⑧ ⑥ ❄ ❄ ⑧ ❆ ⑥ ⑧ ❄ ❄ ❆ ˚ hm ˚ hm ř n ´ 1 j “ 0 ccp ord 0 ❆ ❄ ❄ ucp ❆ ccp ❄ ccp ❄ ⑥ ⑧ ❆ ❄ ❄ ⑧ ⑥ ⑧ ⑧ ⑥ ⑧ ⑧ F i F i F i Some examples of colourings hyperfinite AF dim nuc p A q ú ú Rohklin Theorems Rohklin Poperty Rohklin dim ú ú McDuff ( M – M b R ) A – A b UHF A – A b Z ú ú a.u. equivalence a.u. equivalence ? ú ú . . . . . . . . . ú ú BBSTWW := Bosa, Brown, Sato, Tikusis, W. , Winter 1

� Injectivity ù ñ hyperfiniteness Connes’ 3 ingredients A (separably acting) injective II 1 factor M 1. is McDuff: M – M b R (get sequence of isomorphisms φ n : M b R – Ñ M such that φ n p x b 1 R q Ñ x ) 2. has approximately inner flip, ( D sequence p v n q of unitaries in M b M with v n p x b y q v ˚ n Ñ y b x ); Ñ R ω (can assume θ represented by θ n : M Ñ F n Ă 3. has an embedding θ : M ã R s.t. p id M b θ n qp v n q « w n , for a unitary w n ) p id M b 1 R q ω � p M b R q ω M 1 M b θ Then p x b 1 R ω q « p id M b θ n qp v n qp 1 M b θ n p x qqp id M b θ n qp v ˚ n q « w n p 1 M b θ n p x qq w ˚ n Thus x « φ n p w n p 1 M b θ n p x qq w ˚ n q P φ n p w n p 1 M b F n q w ˚ n q . C ˚ -versions of Connes’ argument Connes’ 3 ingredients A (separably acting) injective II 1 factor M 1. is McDuff: M – M b R ; 2. has approximately inner flip; Ñ R ω . 3. has an embedding θ : M ã Proposition 1 (Efros, Rosenberg (78)) . Let A be a separable unital C ˚ -algebra such that: 1. A absorbs the universal UHF algebra Q , ( A – A b Q ); 2. A has approximately inner flip ( ù ñ nuclearity, simplicity, at most one trace). 3. A is quasidiagonal (for A sep, unital, nuclear, QD ô A unital Ñ Q ω ); ã Then A is AF (and in fact A – Q ). 2

Coloured equivalence K -theoretic obstructions to approx inner flips e.g. AF with inner flip are UHF. Definition 1 (BBSTWW 15) . Let φ, ψ : A Ñ B be unital ˚ -hms. Say φ and ψ are n -coloured equivalent if D u 1 , ¨ ¨ ¨ , u n P B such that: • φ p x q “ ř n ψ p x q “ ř n i “ 1 u i ψ p x q u ˚ i “ 1 u ˚ i , i φ p x q u i • r u ˚ i u i , ψ p A qs “ r u i u ˚ i , φ p A qs “ 0 Theorem (Matui, Sato 13) Let A be simple, unital, separable, nuclear, UHF-stable with unique trace. Then A has a 2 -coloured approx inner flip. • If A also QD, then decomposition rank A at most 1 • Simple, unital, separable, unclear, QD, Z -stable C ˚ -algebras with unique trace have decomposition rank at most 3 . Theorem (BBSTWW, 15) Let A be unital, sep and nuclear. Let B be unital, simple, separable, Z -stable such that QT p B q “ T p B q and T p B q has compact boundary. Let φ, ψ : A Ñ B ω be unital ˚ -hms such that φ p a q , ψ p a q is full in B ω for each non-zero a P A ` .Then φ, ψ 2 -coloured equivalent ô @ τ P T p B ω q , τ ˝ φ “ τ ˝ ψ II 1 factor inj, sep predual M ω are unitarily equivalent iff τ M ω ˝ φ “ Coloured version of φ, ψ : Ñ B τ M ω . Coloured quasidiagonality Theorem (Voiculescu) Quasidiagonality a homotopy invariant. • The cone C 0 pp 0 , 1 s , A q on any C ˚ -algebra is QD. • Exist order zero maps A Ñ Q ω . Theorem (Sato, W., Winter 14) Let A be a nuclear C ˚ -algebra and τ A a trace on A . Then there exists an order zero map φ : A Ñ Q ω , which is a ˚ -hm modulo traces and τ A “ τ Q ω ˝ Φ . • Uses injectivity ù ñ hyperfiniteness in an essential way. • Can assemble two of these maps to get “ 2 -coloured quasidiagonality of τ A ”. • Z -stable, simple, separable, unital, nuclear C ˚ -algebras with unique trace have nuclear dimension ď 3 . 3

Combining coloured quasidiagonality and coloured equivalence, and counting the colours very carefully gives: Theorem (BBSTWW, ’15) Let A be simple, separable, unital, nuclear, Z -stable such that T p A q has compact boundary. 1. dim nuc p A q “ 1 (unless A is AF, when it is zero); 2. If all traces on A are quasidiagonal, then A has decomposition rank 1 (unless A is AF, when it is zero). When 2 become 1 Colours circumvent topological obstructions When there are no topological obstructions, do we really need colours? Conjecture (Blackadar, Kirchberg) Stably finite unital nuclear C ˚ -algebras are quasidiagonal. theorem (Tikuisis, W., Winter) A faithful trace τ A on separable, unital and nuclear C ˚ -algebra A in the UCT class is quasidigaonal: D ˚ -hm Φ : A Ñ Q ω such that τ A “ τ Q ω ˝ Φ . • Discrete amenable groups have quasidiagonal C ˚ -algebras (answering a ques- tion of Rosenberg). • Answers Blackadar-Kirchberg in UCT case with a faithful trace • Completes classification of simple, separable, unital, nuclear C ˚ -algebras of fi- nite nuclear dimension with the UCT (via Elliott, Gong, Lin, Niu). 4