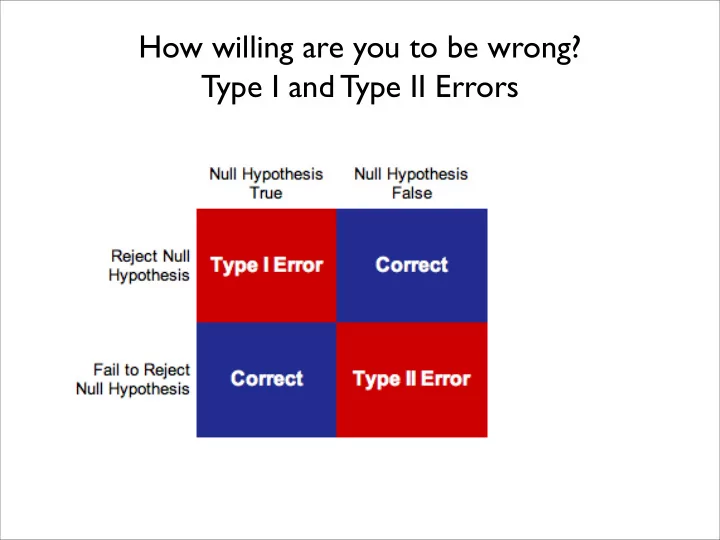
How willing are you to be wrong? Type I and Type II Errors Type 1, - PowerPoint PPT Presentation

How willing are you to be wrong? Type I and Type II Errors

Type 1, Type II Errors and Power Distribution of means for reference data Distribution of a.k.a. Ho means for my data 5% of the data (the upper tail) is shaded, we will allow the distributions to overlap here and still reject Ho.

Type 1 and Type II Errors • Our distributions overlap in the Type I Error region, our “rejection region”. “If our observed mean is > 1.16, we reject the null hypothesis.” • Thus we ALWAYS reject the null hypothesis in this case, and our power is 100%

Type I and Type II Errors Our distributions match almost perfectly Ho=true. Yet, we will still reject Ho 5% of the time. Type I Error Our sample mean is 1.34. We reject Ho because 1.34 exceeds our critical value and is within the 0.05 tail of our reference

Type I and Type II Errors • As the true means of the populations get closer... Type II Error Our sample mean is 0.87. We do NOT reject Ho because 0.87 does not exceed our critical value.

As the distance between means shrinks, the power goes down because there is more overlap. Here, 50% of the means drawn from our population are less than the critical value, 1.16. So we can only detect a difference for 50% of our possible means. How can we increase our power?

To increase power, increase ‘n’

Type I and Type II errors

Calculating Power One-tailed test (right tailed) U = µ 0 + z σ X n X U our upper critical value, X-value here µ 0 mean for H o z critical value of z given your choice of α σ but just in case, it’s the standard error of the mean you should know this one by now n

Calculating Power One-tailed test (right tailed) Now find the z-value and beta ( β ) z = X U − x X U our upper critical value, X-value here σ β x the observed sample mean n For a right-tailed test find β β =P(z<z-critical) Power=1- β = P(z>z-critical)

Example We have a sample of n=100 with a standard deviation of 3.6 and a mean of 17.9. We choose the following: α =0.05 Ho: μ =17.5 Ha: μ >17.5 What is the power of our z-test?

Calculating Power U = 17.5 + 1.65 3.6 U = µ 0 + z σ X X n 10 X U = 18.1 z = X U − x z = 18.1 − 17.9 σ β β 0.36 n z = 0.54 β Probability of getting a value to the RIGHT of 0.54 p=0.2946 = power (1- β )

Increasing power • Increase alpha ( α ) • Increase “n” • Decrease standard deviation • Use a 1-tailed test

JMP time