H = 2 J S S j over the neighbor atoms ij i j - PDF document

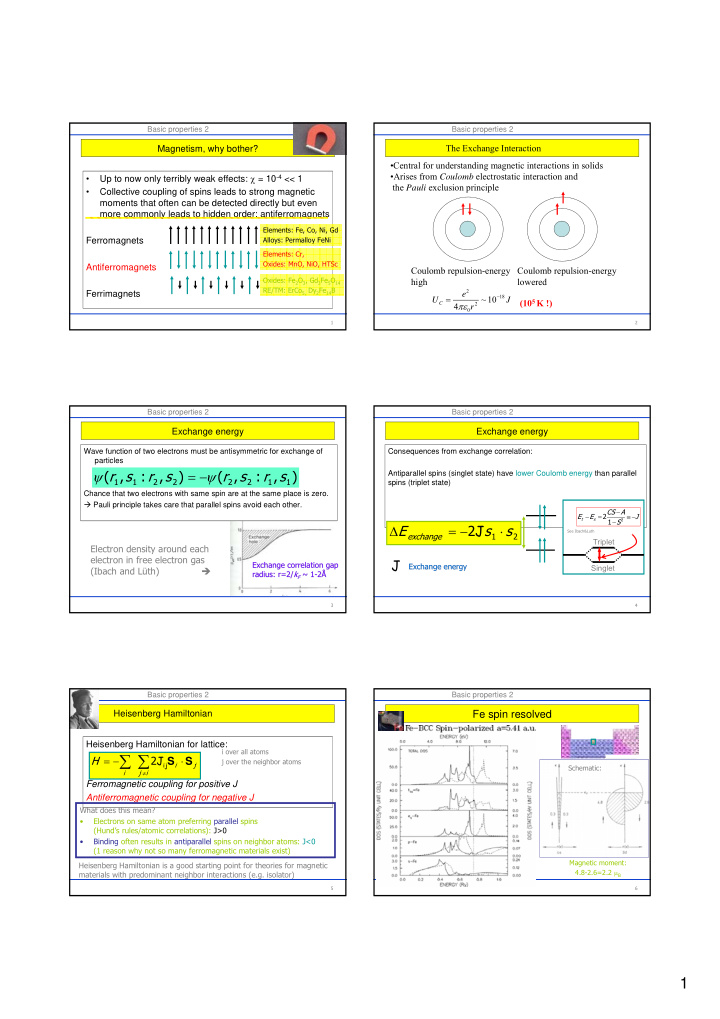
Basic properties 2 Basic properties 2 The Exchange Interaction Magnetism, why bother? •Central for understanding magnetic interactions in solids •Arises from Coulomb electrostatic interaction and Up to now only terribly weak effects: χ = 10 -4 << 1 • the Pauli exclusion principle • Collective coupling of spins leads to strong magnetic moments that often can be detected directly but even more commonly leads to hidden order: antiferromagnets Elements: Fe, Co, Ni, Gd Alloys: Permalloy FeNi Ferromagnets Elements: Cr, Oxides: MnO, NiO, HTSc Antiferromagnets Coulomb repulsion-energy Coulomb repulsion-energy Oxides: Fe 2 O 3 , Gd 3 Fe 5 O 14 high lowered RE/TM: ErCo 5, Dy 2 Fe 14 B 2 Ferrimagnets e = πε − 18 ~ 10 U C J (10 5 K !) 2 4 r 0 1 2 Basic properties 2 Basic properties 2 Exchange energy Exchange energy Wave function of two electrons must be antisymmetric for exchange of Consequences from exchange correlation: particles ψ ( r , s : r , s ) = − ψ ( r , s : r , s ) Antiparallel spins (singlet state) have lower Coulomb energy than parallel 1 1 2 2 2 2 1 1 spins (triplet state) Chance that two electrons with same spin are at the same place is zero. � Pauli principle takes care that parallel spins avoid each other. CS − A E − E = 2 ≡ − J t s 1 − S 2 J Δ E exchange = − 2 s ⋅ s See Ibach&Luth 1 2 Triplet Electron density around each electron in free electron gas J Exchange correlation gap Exchange correlation gap Exchange energy Exchange energy Singlet (Ibach and Lüth) � radius: r=2/ radius: r=2/ k k F F ~ 1 ~ 1- -2Å 2Å 3 4 Basic properties 2 Basic properties 2 Heisenberg Hamiltonian Fe spin resolved Heisenberg Hamiltonian for lattice: i over all atoms ∑ ∑ H = − 2 J ⋅ S S j over the neighbor atoms ij i j Schematic: i j ≠ i Ferromagnetic coupling for positive J Antiferromagnetic coupling for negative J What does this mean? • Electrons on same atom preferring parallel spins (Hund’s rules/atomic correlations): J>0 • Binding often results in antiparallel spins on neighbor atoms: J<0 (1 reason why not so many ferromagnetic materials exist) Magnetic moment: Heisenberg Hamiltonian is a good starting point for theories for magnetic 4.8-2.6=2.2 μ B materials with predominant neighbor interactions (e.g. isolator) 5 6 1

Basic properties 2 Basic properties 2 CS − A E − E = 2 ≡ − J t s 1 − S 2 Properties of ferromagnets Heisenberg Hamiltonian Triplet Not only whole or half integer moments Heisenberg model Hamiltonian Singlet ∑ ∑ J H = − 2 ⋅ S S ij i j i j ≠ i Ferromagnetic coupling for positive J Antiferromagnetic for negative J • Electrons on the same atom can have parallel spins (Hund’s rules/atomic correlations) • Bonding tends to lead to antiparallel moments on neighboring atoms (one reason why there are not many magnetic compounds) • But if J is positive neighboring atom moments couple parallel • Heisenberg Hamiltonian is good starting point for many theories on magnetism for systems with only pair wise interactions 7 8 Basic properties 2 Basic properties 2 Exchange interaction between Free Electrons I&L8.3 Exchange interaction between Free Electrons 1 ( ) ( )( ) i r ψ ( ) = e i + ⎛ i − − ⎞ k 1 r k r k r k k r r • Free electron wavefunction i i j j ⎜ i j i j ⎟ ψ ( − ) = e 1 − e r r V ⎜ ⎟ ij i j 2 V ⎝ ⎠ • Two free electron pair wavefunction • Probability of finding electron 1 in d r 1 and electron 2 in d r 2 : i 1 i k r 1 k r [ ( )( ) ] 2 ψ ( ) = e i i e j j ψ ( − ) d d = 1 − cos − − d d r r r r r k k r r r r ij V ij i j i j 2 i j i j i j V • space antisymmetric: � Two electrons with same spin cannot be at same position ⎛ i i i ⎞ 1 i k r k r k r k r j j i j j i ψ ( , ) = ⎜ e i i e − e e ⎟ � Ionic charge of a spin-up electron is not screened by other spin-up r r ⎜ ⎟ ij i j 2 V electrons. ⎝ ⎠ ( ) ( )( ) � This lowers the energy and leads to a 1 i + ⎛ i − − ⎞ k r k r k k r r ψ ( − ) = e i i j j ⎜ 1 − e i j i j ⎟ collective exchange interaction with positive sign r r ⎜ ⎟ ij i j 2 V ⎝ ⎠ 9 10 Basic properties 2 Basic properties 2 Band (Stoner) Model of ferromagnetism Exchange hole Heisenberg model does not completely explain Exchange interaction causes each spin to push away other spins � local charge density modified: Exchange hole ferromagnetism in metals. up electrons only: I n = − ↑ ( ) ( ) S E k E k ( ) ⎛ 2 ⎞ ↑ en sin k r − cos k r ( ) ⎜ ⎟ N ρ = 1 − 9 F F ex r ⎜ ( ) ⎟ 2 6 k r ⎝ ⎠ F I n radius radius = − ( ) ( ) ↓ S E k E k r=2/ k r=2/ k F F ~ 1 ~ 1- -2Å 2Å ↓ All electrons N ( ) ⎛ ⎞ 9 sin k r − cos k r 2 ( ) ⎜ ⎟ ρ = en 1 − F F ex r ⎜ ( ) ⎟ 6 I s is Stoner parameter and describes energy 2 k r ⎝ ⎠ F reduction due to electron spin correlation Use this density in Schrödinger equation: Hartree-Fock approximation. n , ↑ n is density of up, down spins ↓ Full theory Density Functional theory in Local Density Approximation (DF-LDA) 11 12 2

Basic properties 2 Basic properties 2 Band (Stoner) Model Band (Stoner) Model ↑ − Δ = with x I R n n Let R be small, use Taylor expansion: = N s ↓ R = μ 3 ⎛ Δ M R 2 ⎞ (spin excess) x B − Δ − + Δ ≈ − Δ − + N ( / 2 ) ( / 2 ) ' ( ) ' ' ' ( ) ⎜ ⎟ ... V g x x g x x g x x g x 3 ! ⎝ 2 ⎠ ~ = − Then ( ) ( ) / 2 ∂ ∂ 3 E k E k I R 1 ( ) 1 ( ) ∑ f k ∑ f k ↑ + ↑ ( ) = − − + S ~ I n n 3 ( ) ( ) ... R ~ I R ~ I R = − ↓ ~ ( ) ( ) s E k E k ∂ s ∂ 3 s = + ( ) 24 ( ) ( ) ( ) / 2 2 N N N E k E k E k E k I R ↓ k k S Spin excess given by Fermi statistics: ∂ ∂ ⎛ ⎞ ⎛ ⎞ ~ f V f V ∑ ∫ ∫ 1 → = − δ − ⎜ ⎟ ⎜ ⎟ ( ( )) ∑ ~ dk ~ dk E E = − ( ) ( ) ( ) R f k f k ∂ π 3 ∂ 3 ⎝ ⎠ ( 2 ) ⎝ ⎠ π F 2 E N E ↑ ↓ N N k k (at T=0) [ ( ) ] ~ V − 1 = − = − ( ) exp ( ) / 2 / m D E f(E) f E k I R E kT ↑ ↓ F , s F 2 D.O.S.: density of states at Fermi level E E F 13 14 Basic properties 2 Basic properties 2 Band (Stoner) Model ) and Δ Δ E for a 3d g(E F g(E F ) and E for a 3d ferromagnet ferromagnet ~ ( ) V Density of states = ( ) 1. DOS at Fermi level is greater in a 3d TM: 1. DOS at Fermi level is greater in a 3d TM: D E D E F 2 F N per atom per spin E 4s 4s E ~ ( ) = − ( 3 ) Third order Then R D E I R O F s terms E F E ~ F ( ) 3d 3d − = − ( 3 ) ( 1 ) R D E I O F s When is R> 0? ~ ( ) ~ > ( ) 1 − < D E F I 1 0 or D E F I s s Stoner Condition for Ferromagnetism For Fe, Co, Ni this condition is true bandwidth of 3d band smaller ( bandwidth of 3d band smaller (d d- -d d overlap smaller than overlap smaller than s s- -s s) ) band also contains more electrons (can take 10 in total, vs. 2 band also contains more electrons (can take 10 in total, vs. 2 for 4s band) for 4s band) Doesn’t work for rare earths, though 15 16 Basic properties 2 Basic properties 2 Spin resolved DOS: 3d Spin resolved DOS: 3d ferromagnet ferromagnet Band structure 2. Δ Δ E from alignment with (or against) B 2. E from alignment with (or against) B 0 0 is greater in a 3d TM: is greater in a 3d TM: plus: even a even a plus: small change in small change in Exchange interaction splits spin degenerated bands 4s 4s E E energy, Δ energy, Δ E, leads E, leads to a relatively to a relatively 3d 3d large no. of large no. of E E F E F F electrons electrons changing spin changing spin ↓ state state ↑ Δ Δ E E Ẽ ↑↓ ( k ) I ↓ ↑ N ↓ N ↑ N N 17 18 3