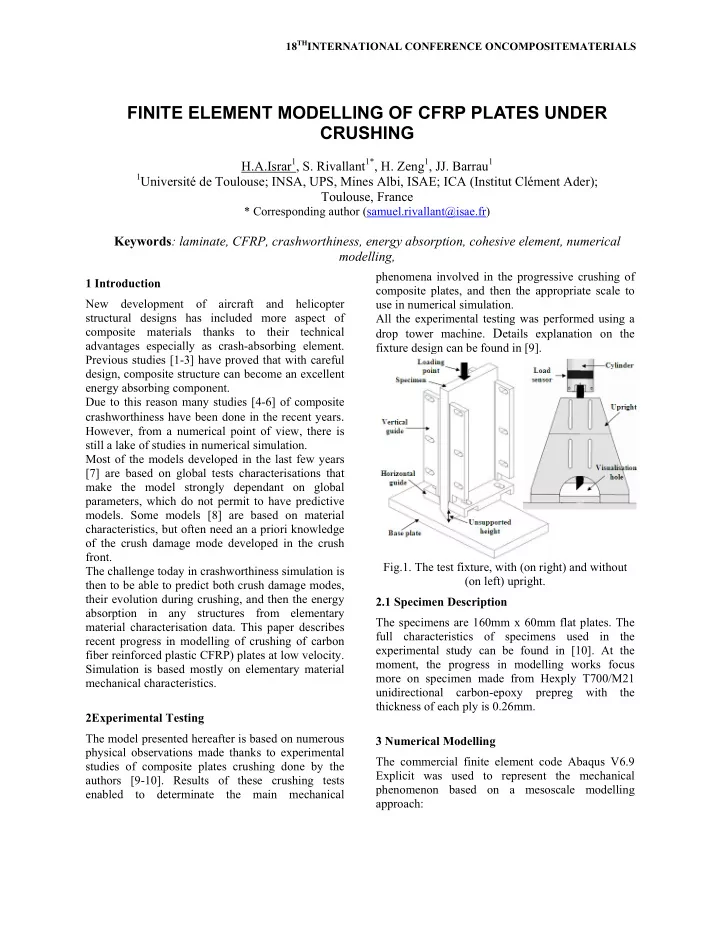

18 TH INTERNATIONAL CONFERENCE ONCOMPOSITEMATERIALS FINITE ELEMENT MODELLING OF CFRP PLATES UNDER CRUSHING H.A.Israr 1 , S. Rivallant 1* , H. Zeng 1 , JJ. Barrau 1 1 Université de Toulouse; INSA, UPS, Mines Albi, ISAE; ICA (Institut Clément Ader); Toulouse, France * Corresponding author (samuel.rivallant@isae.fr) Keywords : laminate, CFRP, crashworthiness, energy absorption, cohesive element, numerical modelling, phenomena involved in the progressive crushing of 1 Introduction composite plates, and then the appropriate scale to New development of aircraft and helicopter use in numerical simulation. structural designs has included more aspect of All the experimental testing was performed using a composite materials thanks to their technical drop tower machine. D etails explanation on the advantages especially as crash-absorbing element. fixture design can be found in [9]. Previous studies [1-3] have proved that with careful design, composite structure can become an excellent energy absorbing component. Due to this reason many studies [4-6] of composite crashworthiness have been done in the recent years . However, from a numerical point of view, there is still a lake of studies in numerical simulation. Most of the models developed in the last few years [7] are based on global tests characterisations that make the model strongly dependant on global parameters, which do not permit to have predictive models. Some models [8] are based on material characteristics, but often need an a priori knowledge of the crush damage mode developed in the crush front. Fig.1. The test fixture, with (on right) and without The challenge today in crashworthiness simulation is (on left) upright. then to be able to predict both crush damage modes, their evolution during crushing, and then the energy 2.1 Specimen Description absorption in any structures from elementary The specimens are 160mm x 60mm flat plates. The material characterisation data. This paper describes full characteristics of specimens used in the recent progress in modelling of crushing of carbon experimental study can be found in [10]. At the fiber reinforced plastic CFRP) plates at low velocity. moment, the progress in modelling works focus Simulation is based mostly on elementary material more on specimen made from Hexply T700/M21 mechanical characteristics. unidirectional carbon-epoxy prepreg with the thickness of each ply is 0.26mm. 2Experimental Testing The model presented hereafter is based on numerous 3 Numerical Modelling physical observations made thanks to experimental The commercial finite element code Abaqus V6.9 studies of composite plates crushing done by the Explicit was used to represent the mechanical authors [9-10]. Results of these crushing tests phenomenon based on a mesoscale modelling enabled to determinate the main mechanical approach:
- meshin ng of each ply y of the comp posite, - cohesiv ve elements b between plies s to take into accoun nt delaminat ion and spl laying with fractur re mechanics criteria - cohesiv ve elements inside plies to represent rupture e of plies into o fragment wi ithout losing mass a and volume - modell ling of fra agmentation thanks to rupture e criteria in n the ply (f for big size fragme ents) and sp ecific criteri a for small fragme ents localised d at the cru ushing front Fig g.3. Modellin ng: pure splay ying mode [11 1]. extrem mity - contact t laws to rule e debris accum mulation and 3.1 Mo odel Descript tion debris wedge appea aring. The lam minated plate e is modelled in three-dime ension. Th his model i s applied to o simulate th he crushing Only 1 100 mm leng gth of the pl late is mode elled to mixed-mode m phenomen non (splay ying and reduce the calculatio on time, whic ch is long eno ough to fr agmentation) ) (fig.2) of f laminated plate with have re ealistic bound dary conditio ons on the m metallic st acking seque ence [(0°/90°) )*4] sym and a a (0/45/90)n plate a and the guid des. Besides that, for the e same qu uasi-isotropic c laminate i in pure spla aying mode reason only one elem ment of 0.25m mm width is u used to (f fig.3). The nu umerical mod del for the c case of pure represe ent the 60mm m width of the plate b because sp playing mode e have been d done and com mpared with experim ments show th hat deformati ions along the e width th he experiment tal works and d gives good results [11]. are uni iform. The p plate lay-up i is modelled by 3D However, the H 2D continuu um shell ele ements used solid el lement C3D8 8 (8 nodes wi ith full integr ration). le ead to problem ms of instabil lity in the cas se of mixed- The dim mension of e each element t is 0.25mm in the m mode simulatio on. Thus, the current mod elling works thickne ess and also o 0.25mm in the othe er two fo ocus more on n mixed-mod de model usin ng 3D solid directio ons. The 8 nodes 3D cohesive el ements el lements and t triggering me echanism of 4 45° chamfer (COH3 3D8) with ze ro thickness have been u used to as s an improvem ment of the pr revious mode el in order to model the interface e bonding be etween the p plies. It re epresent bette er crush initi iation and fr ragmentation also ha as been place d inside the plies for the reason m mode. The sim mulation is b based on a d dynamic test mention ned earlier (fi fig.4). Nodes a and interface s at the w with a falling mass at the e initial spee d of 9 m/s. bottom of the plate d do not have a any imperfect tions to Fo orce/displacem ment curve and the high speed promot te an initial fa ailure as used d in the recen nt study ca ameras of the e experimenta al works are available to [12]. D Details of lam inated plate m model are pre esented make precise m correlation b between expe eriment and in the f fig.5. In orde er to represen nt the falling weight sim mulation. during the test, an a additional ma ass is added on the nodes a at the top of f the plate. Th he contact be ehavior betwee n the plate e elements, the e metallic pla ate and y z the gui ides is ruled by general c contact with f friction x coeffici ient μ =0.1. Coh hesive elemen nts: Cohesi ive elements: Fragme entation inte rface bonding g insid de the plies D Debris Splaying A Accumulatio on Fig.2. Mod elling: fragm entation and s splaying. Fig.4. Cohes sive element a arrangement.
The da amage propa agation is ru uled by a b bilinear softenin ng law. The l linear decrea se is calculat ted and V 0 =9 m/s so, the e energy abs sorbed is eq qual to the energy release rate at inte erface in eac ch mode. Co oupling betwee n three mode s is chosen lin near: Rigid Elem ment : G ∑ (2) Gu uides i G ic The sa ame law have e been applie ed on the co ohesive Laminate elemen nts inside the plies but wi ith higher va lues of energy and nominal traction stres ss in order to b be able to repre esent the ruptu ure of the plie es. The co ohesive elem ments propert ties were inc creased Rigid Element : from t the baseline e values to o more acc curately Metal llic Plate represe ent the right c crushing morp phology as ob btained y in expe eriments. z E 1 E 2 G 12 υ 12 x Solid d (GPa) (GPa) (GPa) elemen nt 125 9 5 0.33 Fig g.5. Laminate e plate model. . Interfac ce: Y t S c G I G II mJ/mm 2 ) mJ/mm 2 ) Inter- - (MPa) (MPa) (m (m 3. .2 Delaminat tion Law lamina ar 350 150 2 1.2 Th he delaminati ion law is bas sed on tractio n-separation Y t S c G I G II an nd consist of f three states s: a damage initiation, a Interfac ce: mJ/mm 2 ) mJ/mm 2 ) (MPa) (MPa) (m (m Inside e da amage evolut tion and a co mpletely sep aration state plies s 1750 150 120 1.2 w with the follow wing behaviou ur law: Table 1. Mechanica al characterist tic of T700/M M21 for δ δ ⎧ ⎫ ⎡ ⎤ ⎡ ⎤ t K 0 0 0 n nn n ⎪ ⎪ − simulation. 1 D D ⎢ ⎥ ⎢ ⎥ = × × δ δ (1) t 0 K K 0 ⎨ ⎬ ⎢ ⎥ ⎢ ⎥ s ss s T ⎪ ⎪ ⎢ ⎥ ⎢ ⎥ 0 δ δ t 0 0 0 K ⎩ ⎭ ⎣ ⎦ ⎣ ⎦ t tt t 4 Resu lt Discussion ns Where (t n , t s a W and t t ) are str resses in the normal and Both th he numericall ly simulation n force/displac cement th he two local shear direct tions, [K] is the rigidity curve a and the crush hing physical l phenomeno n have m matrix, ( δ n, δ s s and δ t) are e the separati ions in each being compared w with the ex xperimental r results. di irection. Genera ally, results o of numerical modelling s show a good c orrelation bet tween behavi iour during te est and simulat tion. The ill lustrations of f crash initia ation obtained d from both e experimental and num merical mode el are present ted in fig.7 at different inte ervals of time s. The de formation seq quence of sim mulation mod del and experim ment was very y similar to e each other (in itiation occurre ed at the sam me displaceme ent followed by the same d elamination l ocation of the e related plies s). Fig g.6. Evolution n of damage. It show ws this sim mulation mod del is capa ble of capturin ng the initia ation of the crushing pla ate that D amage initia ates when on ne of the th hree stresses consist both splayin ng and fragm mentation mo odes at (f fig.6) reaches its maximal value. Maxim mal value is the sam me time. th he rupture value calculated c from the ch haracterisation n test of comp posite ply.
Recommend
More recommend