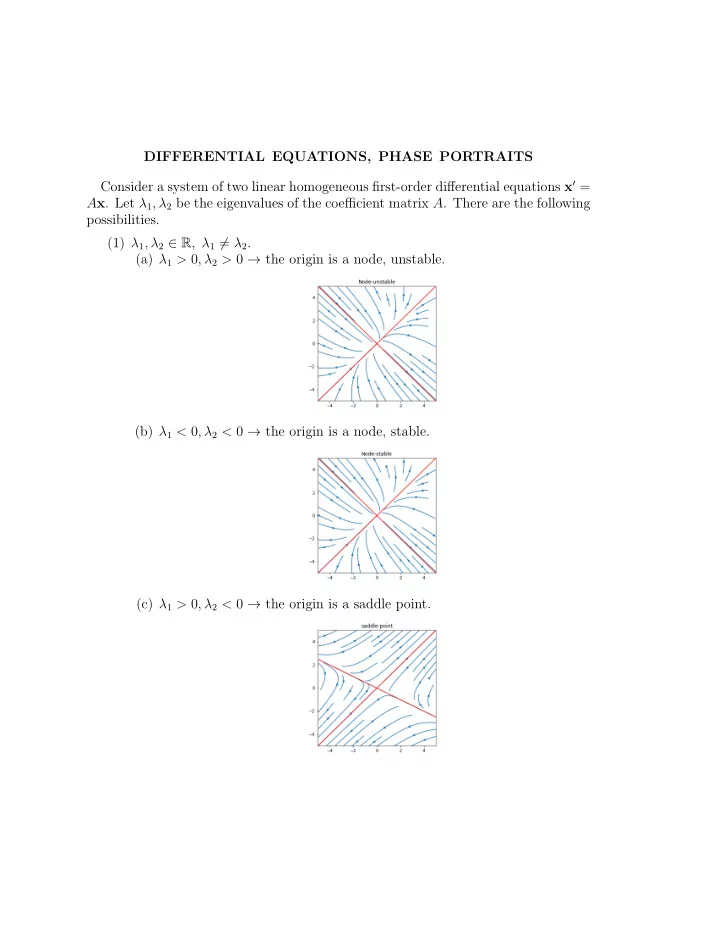
SLIDE 1 DIFFERENTIAL EQUATIONS, PHASE PORTRAITS Consider a system of two linear homogeneous first-order differential equations x′ =
- Ax. Let λ1, λ2 be the eigenvalues of the coefficient matrix A. There are the following
possibilities. (1) λ1, λ2 ∈ R, λ1 = λ2. (a) λ1 > 0, λ2 > 0 → the origin is a node, unstable. (b) λ1 < 0, λ2 < 0 → the origin is a node, stable. (c) λ1 > 0, λ2 < 0 → the origin is a saddle point.
SLIDE 2
2 DIFFERENTIAL EQUATIONS, PHASE PORTRAITS
(d) λ1 > 0, λ2 = 0 → the eigenspace corresponding to λ = 0 (in green) is an unstable line. (e) λ1 < 0, λ2 = 0 → the eigenspace corresponding to λ = 0 (in green) is a stable line. (2) λ1, λ2 ∈ R, λ1 = λ2, two eigenvectors. (a) λ1 = λ2 > 0 → the origin is a star, unstable. (b) λ1 = λ2 < 0 → the origin is a star, stable.
SLIDE 3
DIFFERENTIAL EQUATIONS, PHASE PORTRAITS 3
(3) λ1, λ2 ∈ R, λ1 = λ2, one eigenvector. (a) λ1 = λ2 > 0 → the origin is a improper node, unstable. (b) λ1 = λ2 < 0 → the origin is a improper node, stable. (4) λ1, λ2 ∈ C, λ1 = λ + iµ, λ2 = λ − iµ (a) λ > 0 → the origin is a spiral point unstable.
SLIDE 4
4 DIFFERENTIAL EQUATIONS, PHASE PORTRAITS
(b) λ < 0 → the origin is a spiral point stable. (c) λ = 0 → the origin is a center.
