Detection of Co-orbital Exoplanets A. Leleu 1 , P. Robutel 1 , A.C.M. - PDF document

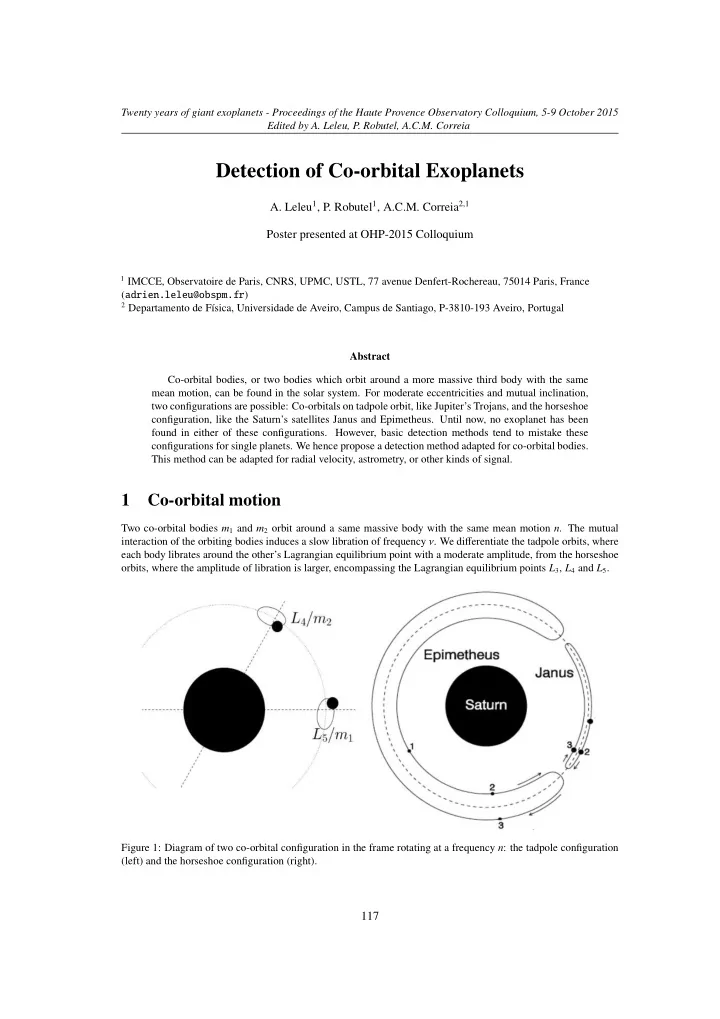
Twenty years of giant exoplanets - Proceedings of the Haute Provence Observatory Colloquium, 5-9 October 2015 Edited by A. Leleu, P. Robutel, A.C.M. Correia Detection of Co-orbital Exoplanets A. Leleu 1 , P. Robutel 1 , A.C.M. Correia 2 , 1 Poster presented at OHP-2015 Colloquium 1 IMCCE, Observatoire de Paris, CNRS, UPMC, USTL, 77 avenue Denfert-Rochereau, 75014 Paris, France ( adrien.leleu@obspm.fr ) 2 Departamento de F´ ısica, Universidade de Aveiro, Campus de Santiago, P-3810-193 Aveiro, Portugal Abstract Co-orbital bodies, or two bodies which orbit around a more massive third body with the same mean motion, can be found in the solar system. For moderate eccentricities and mutual inclination, two configurations are possible: Co-orbitals on tadpole orbit, like Jupiter’s Trojans, and the horseshoe configuration, like the Saturn’s satellites Janus and Epimetheus. Until now, no exoplanet has been found in either of these configurations. However, basic detection methods tend to mistake these configurations for single planets. We hence propose a detection method adapted for co-orbital bodies. This method can be adapted for radial velocity, astrometry, or other kinds of signal. 1 Co-orbital motion Two co-orbital bodies m 1 and m 2 orbit around a same massive body with the same mean motion n . The mutual interaction of the orbiting bodies induces a slow libration of frequency ν . We di ff erentiate the tadpole orbits, where each body librates around the other’s Lagrangian equilibrium point with a moderate amplitude, from the horseshoe orbits, where the amplitude of libration is larger, encompassing the Lagrangian equilibrium points L 3 , L 4 and L 5 . Figure 1: Diagram of two co-orbital configuration in the frame rotating at a frequency n : the tadpole configuration (left) and the horseshoe configuration (right). 117

Twenty years of giant exoplanets - Proceedings of the Haute Provence Observatory Colloquium, 5-9 October 2015 Edited by A. Leleu, P. Robutel, A.C.M. Correia 2 Detection Method As hinted by Laughlin & Chambers (2002), the reflex motion of a star induced by co-orbital exoplanets has the shape of a modulated signal (Fig. 2, top). The short periods are due to the mean motion n of the barycentre of the two planets, while the amplitude modulation is due to the variation of the distance between the planet’s barycentre and the star. The amplitude of the modulation depends on the amplitude of the libration and the mass repartition between the two co-orbitals. In the Fast Fourier Transform (FFT) of the raw data (Fig. 2, bottom left) one can identify a single peak K 0 cos nt that can be mistaken for a Keplerian orbit. The demodulation method consist in subtracting this peak to the signal, then multiply the signal by cos nt . It yields a signal whose FFT can be seen on Fig. 2, bottom right. On this spectrum, a peak at P ν is easily identifiable. 6.6 6.58 6.56 6.54 RV[km/s] 6.52 6.5 6.48 6.46 6.44 6.42 6.4 0 100 200 300 400 500 T[day] 1 0.5 0.9 0.45 0.8 0.4 0.7 0.35 0.6 0.3 Power Power 0.5 0.25 0.4 0.2 0.3 0.15 0.2 0.1 0.1 0.05 0 0 1 10 100 1000 10000 1 10 100 1000 10000 Period[d] Period[d] Figure 2: Top: In black: radial velocity induced by co-orbitals in tadpole configuration, P n ≈ 11 . 5 d and P ν ≈ 110 d . In red: realistic dataset with a simulated precision of 1 m / s . The full dataset is 4750 days long. Bottom left: FFT of the raw data. Bottom right: FFT of the data after demodulation. With the frequencies n and ν , and the amplitudes and phases of the peaks in P n and P ν , an analytical model (Leleu et al. 2015) allows us to determine the orbital parameters a j , λ j and the masses m j sin I of the co-orbitals. In the case of a tadpole configuration, sin I can be determined in order to get the m j . 118

Twenty years of giant exoplanets - Proceedings of the Haute Provence Observatory Colloquium, 5-9 October 2015 Edited by A. Leleu, P. Robutel, A.C.M. Correia Figure 3: Detectability of co-orbitals in a given dataset: Let us consider a dataset in which we found a peak of the form K 0 cos nt that we believed to be a single planet m p ≈ 10 − 3 m star , the black lines gives the minimum time span T and accuracy ǫ needed to detect co-orbitals with the given mass distribution. For example, the shaded part represent the detectable configurations for K 0 = 40 ǫ and T = 15 P n , considering m 1 ≤ m 2 , we could detect co-orbitals for m 1 down to ≈ m 2 / 10. The higher m 1 + m 2 , the faster the libration, hence reducing the time span needed to get a libration period in the dataset. On the other hand, the amplitude of the modulation, hence the detectability, increases as the amplitude of libration gets larger, and when m 1 and m 2 tend to be equals. Fig. 3 gives the detectable co-orbital configurations from a given dataset. 3 Conclusion We provide a method to extract the co-orbital signature from a dataset using a demodulation technique, then to determine the orbital parameters and the mass of the co-orbitals with an analytical model. The critical parameters for the measurement is obviously the accuracy and the time span, while co-orbitals are easier to detect if more massive, with a large amplitude of libration. The associated article, Leleu et al. (2015), gives more details on the signal, the demodulation method, the extraction from the signal of the orbital parameters and masses of the co-orbitals and provides additional realistic examples. Acknowledgments: Thank you to the organizers of this great conference. References Laughlin, G. & Chambers, J. E. 2002, Astron. J., 124, 592 Leleu, A., Robutel, P., & Correia, A. C. M. 2015, Astron. Astrophys., 581, A128 119