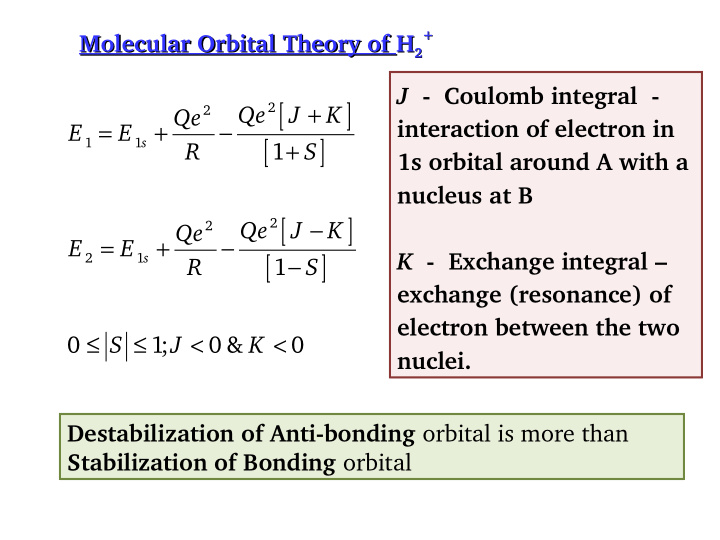
Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 J - - PowerPoint PPT Presentation

+ + Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 J - Coulomb integral - [ ] + 2 2 Qe J K Qe = + − interaction of electron in E E [ ] + 1 1 s R 1 S 1s orbital around A with a nucleus at B [ ] − 2 2 Qe J K Qe = + − E E [ ] − K - Exchange integral – 2 1 s R 1 S exchange (resonance) of electron between the two ≤ ≤ < < 0 1 ; 0 & 0 S J K nuclei. Destabilization of Anti-bonding orbital is more than Stabilization of Bonding orbital

+ + Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 E 2 1 E 1

Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 ignore µ ( h h h h 2 2 2 2 ) = − ∇ − ∇ − ∇ − ∇ 2 2 2 2 H H 2 A B e 1 e 2 2 m 2 m 2 m 2 m A B e e 2 2 2 2 2 2 e e e e e e − − − + + Q Q Q Q Q Q r r r r r R 1 A 1 B 2 A 2 B 12 µ ( h h 2 2 2 2 2 2 2 2 e e e e e e ) = − ∇ − ∇ − − − + + 2 2 H H Q Q Q Q Q Q 2 e 1 e 2 2 m 2 m r r r r r R e e 1 A 1 B 2 A 2 B 12     µ ( h h 2 2 2 2 2 2 2 2 e e e e e e ) = − ∇ + − ∇ + − + + 2 2     H H Q Q Q Q Q Q 2 e 1 e 2  2 m r   2 m r  r r r R e 1 A e 2 B 1 B 2 A 12 µ ( µ ( µ ( 2 2 2 2 e e e e ) ) ) = + − − + + H H H H H H Q Q Q Q Cannot be Solved 2 1 e 2 e r r r R 1 B 2 A 12

Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 ( ) 1 ψ = ψ = φ + φ [ ] bonding 1 1 s 1 s + A B 2 2 S Place the second electron in the bonding orbital to get H 2 ψ = ψ ψ × ( H ) 2 1 2 bonding       ( ) ( ) 1 1 1 [ ]     = φ + φ × φ + φ α β − β α 1 1 2 2 (1) (2) (1) (2)   [ ] [ ]  1 s 1 s   1 s 1 s   + +  A B A B 2 2 2 2 2 S S    

Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 ψ ( H ) bonding 2   ] ( ) ( ) 1 1 [ ]     = φ + φ × φ + φ α β − β α 1 1 2 2 (1) (2) (1) (2)   [     + 1 s 1 s 1 s 1 s   2 1 S A B A B 2 Spatial Part 1   ψ = φ φ + φ φ + φ φ + φ φ 1 2 1 2 1 2 1 2   [ ] + bonding 1 s 1 s 1 s 1 s 1 s 1 s 1 s 1 s 2 1 S A A B B A B B A 1 [ ] × + × + × + × 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) [ ] + A A B B A B B A 2 1 S

Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 ψ ( H ) − anti bonding 2   ] ( ) ( ) 1 1 [ ]     = φ − φ × φ − φ α β − β α 1 1 2 2 (1) (2) (1) (2)   [     − 1 s 1 s 1 s 1 s   2 1 S A B A B 2 Spatial Part 1   ψ = φ φ + φ φ − φ φ − φ φ 1 2 1 2 1 2 1 2   [ ] − − anti bonding 1 s 1 s 1 s 1 s 1 s 1 s 1 s 1 s 2 1 S A A B B A B B A 1 [ ] × + × − × − × 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) [ ] − A A B B A B B A 2 1 S

Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 1   ψ = φ φ + φ φ − φ φ − φ φ 1 2 1 2 1 2 1 2 [ ]   − − anti bonding 1 s 1 s 1 s 1 s 1 s 1 s 1 s 1 s 2 1 S A A B B A B B A 1 [ ] × + × − × − × 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) [ ] − A A B B A B B A 2 1 S 1   ψ = φ φ + φ φ + φ φ + φ φ 1 2 1 2 1 2 1 2 [ ]   + bonding 1 s 1 s 1 s 1 s 1 s 1 s 1 s 1 s 2 1 S A A B B A B B A 1 [ ] × + × + × + × 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) 1 s (1) 1 s (2) [ ] + A A B B A B B A 2 1 S

Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 Effective nuclear charge changes the absolute energy Levels and the size of orbitals! Matching of energies of AOs important for LCAO-MO If energies are not close to each other, they would Not interact to form MOs.

+ , H + + + Diatoms of First Row: H H 2 , H 2 ,He 2 , He 2 Diatoms of First Row: 2 ,He 2 , He 2 2

Molecular Orbital Theory of H H 2 Molecular Orbital Theory of 2 Effective nuclear charge changes the absolute energy levels and the size of orbitals! Matching of energies of AOs important for LCAO-MO, if the energies of two Aos are not close they will not interact to form MOs.

Matching of AO energies for MO Matching of AO energies for MO Both symmetry and energy Matching is required for MO. Valence electrons are most important Due to large difference in energy of 1s (H) and 1s (F), LCAO-MO for both 1S is not feasible in HF. Rather, 2p z (F) and 1s (H) form a sigma bond.

Bonding in First-Row Homo-Diatomic Molecules Bonding in First-Row Homo-Diatomic Molecules 2p 2p 2s 2s 1s 1s The orbital energies of the two approaching atoms are identical before they start interacting to form a BOND

Bonding in First-Row Homo-Diatomic Molecules Bonding in First-Row Homo-Diatomic Molecules 3 σ * 1 π * 2p 2p 1 π 3 σ 2 σ * 2s 2s 2 σ 1 σ * 1s 1s 1 σ The interaction between the energy and symmetry matched orbitals leads to various types of BONDs

MO Energies of Dinitrogen MO Energies of Dinitrogen Experiments tell us this picture is incorrect!

Bonding in First-Row Homo-Diatomic Molecules Bonding in First-Row Homo-Diatomic Molecules 2p 2p 2s 2s 1s 1s The 2s and 2p orbitals are degenerate in Hydrogen. However in the many electron atoms these two sets of orbitals are no longer degenerate.

Bonding in First-Row Homo-Diatomic Molecules Bonding in First-Row Homo-Diatomic Molecules 2p 2p 2s 2s 1s 1s The difference in the energies of the 2s and 2p orbitals increases along the period. Its is minimum for Li and maximum for Ne

MO Energies of Dinitrogen MO Energies of Dinitrogen Mixing of 2s and 2p orbital occur because of small energy gap between them 2s and 2p electrons feels not so different nuclear charge. Note how the MO of 2s →σ have p-type looks, while π -levels are clean

s-p Mixing: Hybridization of MO s-p Mixing: Hybridization of MO Mixing of 2s and 2p orbital occur because of small energy gap between them 2s and 2p electrons feels not so different nuclear charge

s-p Mixing: Hybridization of MO s-p Mixing: Hybridization of MO Incorrect! B 2 is paramagnetic. This can only happen if the two electrons with parallel spin are placed in the degenerate π -orbitals and if π orbitals are energetically lower than the σ orbital

MO diagram of F 2 : No s-p Mixing MO diagram of F 2 : No s-p Mixing No Mixing of s and p orbital because of higher energy Gap between 2s and 2p levels in Oxygen and Fluorine! 2s and 2p electrons feels very different nuclear charge

MO Energy Level Diagram for Homo-Diatomics MO Energy Level Diagram for Homo-Diatomics Upto N 2 Beyond N 2

Bond-Order and Other Properties Bond-Order and Other Properties N 2 : (1 σ g ) 2 (1 σ * u ) 2 (2 σ g ) 2 (2 σ * u ) 2 (1 π ux ) 2 (1 π uy ) 2 (3 σ g ) 2 BO = 3 All spins paired: diamagnetic O 2 : (1 σ g ) 2 (1 σ * u ) 2 (2 σ g ) 2 (2 σ * u ) 2 (3 σ g ) 2 (1 π ux ) 2 (1 π uy ) 2 (1 π ux ) 1 (1 π * uy ) 1 BO = 2 2 spins unpaired: paramagnetic

MO Contours and Electron Density MO Contours and Electron Density

Hetero-Diatomics: HF Hetero-Diatomics: HF Due to higher electronegativity of F than H, the electron distribution is lopsided

Hetero-Diatomics: CO Hetero-Diatomics: CO

Hybridization Hybridization Linear combination of atomic orbitals within an atom leading to more effective bonding 2p z 2p x 2p y 2p x 2p y α 2s- β 2p z α 2s+ β 2p z 2s The coefficients α and β depend on field strength Hybridization is close to VBT approach. Use of experimental information All hybridized orbitals are equivalent and are ortho-normal to each other