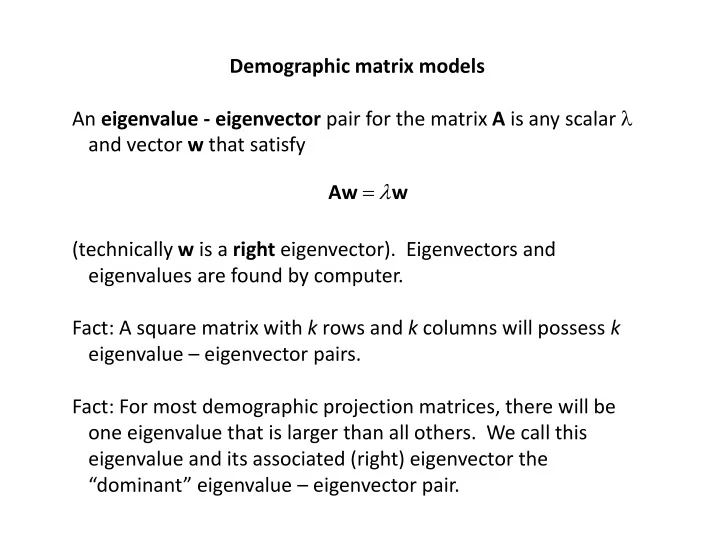
Demographic matrix models An eigenvalue eigenvector pair for the - PowerPoint PPT Presentation

Demographic matrix models An eigenvalue ‐ eigenvector pair for the matrix A is any scalar  and vector w that satisfy   Aw w (technically w is a right eigenvector). Eigenvectors and ( h i ll i i h i ) Ei d eigenvalues are found by computer. Fact: A square matrix with k rows and k columns will possess k eigenvalue – eigenvector pairs. Fact: For most demographic projection matrices, there will be one eigenvalue that is larger than all others. We call this eigenvalue and its associated (right) eigenvector the i l d it i t d ( i ht) i t th “dominant” eigenvalue – eigenvector pair.

Demographic matrix models Fact: The dominant eigenvalue gives the long ‐ run finite rate of increase (  ), and the dominant (right) eigenvalue gives the stable age distribution stable age distribution. Fact: An eigenvalue – left eigenvector pair for the matrix A is any scalar  and vector v that satisfy  l d h i f   T T v A v Fact: The eigenvalues associated with the right eigenvectors are the same as the eigenvalues associated with the left eigenvector eigenvector. Fact: The left eigenvector associated with the dominant eigenvalue  gives the reproductive values.  i i l th d ti l

Teasel, Dipsacus sylvestris small rosette small rosette large rosette flowering stalk photos courtesy Oregon State U