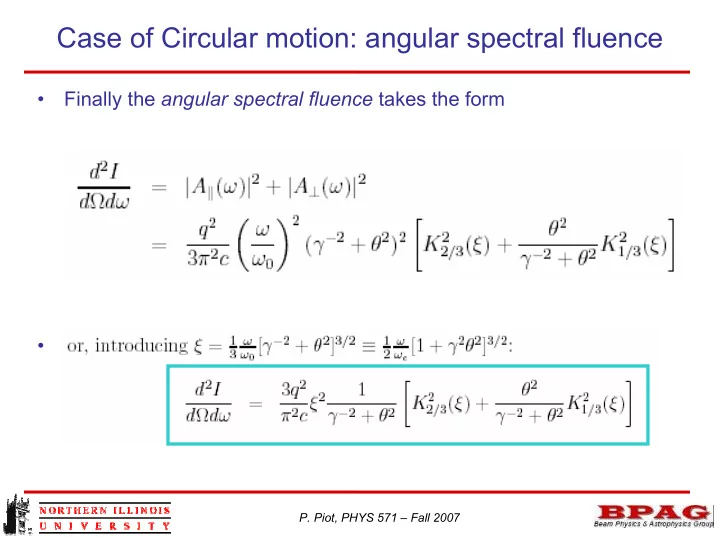

Case of Circular motion: angular spectral fluence • Finally the angular spectral fluence takes the form • …. P. Piot, PHYS 571 – Fall 2007
Angle-integrated spectrum I • Last Lesson we noted – High frequency radiation occupies angles θ < γ -1 (<< γ -1 for ω << ω c ) – Low frequency ( ω << ω c ) we have where the critical angle was defined as P. Piot, PHYS 571 – Fall 2007
Angle-integrated spectrum II • But so • And Broad spectrum γ -independent • • Can do a similar asymptotic expansion for the high frequency region of the angle-integrated spectrum – let as an exercise… P. Piot, PHYS 571 – Fall 2007
Angle-integrated spectrum III • Derived by Schwinger to be 1.33 ( ω/ω c ) 1/3 P. Piot, PHYS 571 – Fall 2007
Angular distribution (frequency integrated) I • Need to evaluate • Change of variable gives: • Where the identity P. Piot, PHYS 571 – Fall 2007
Angular distribution II • So finally we have • Let’s do a consistency check and consider the total radiated energy !!!! • then σ σ π π P. Piot, PHYS 571 – Fall 2007
Total power • So finally we have with • In agreeement with the Pcirc we derived at the beginning of chapter 4: P. Piot, PHYS 571 – Fall 2007
Case of periodic circular motion I • Up to now we considered the steady case circular motion (no transient) and computed instantaneous spectra. • If the motion is periodic P. Piot, PHYS 571 – Fall 2007
Case of periodic circular motion II • And we can show (following the steps we did for the instantaneous case) that Same general form as for instantaneous motion a factor sqrt(2 π ) come from the difference in normalization between Fourier transforms and Fourier series… The spectrum is now discrete at ω = n ω 0 • P. Piot, PHYS 571 – Fall 2007
Multiparticle Coherence I • In real life a bunch consists of many particle so one may wonder how does this affect all the results previously derived • It depends on the frequency (wavelength) of observation! Electric field radiated by two particle at “small” (right) and “long” wavelength (compared to the particle spacing) P. Piot, PHYS 571 – Fall 2007
Multiparticle Coherence II • Let’s compute the total field generated by an ensemble of N electrons. ∑ − ωδ = i t E ( P ) E ( P ) e k N k k • Let’s assume the single particle field have the same value at the observation P. Then spectral angular fluence is 2 2 2 d W d W ∑ − ωδ ∝ ≡ i t 2 | E ( P ) | e k N ω Ω ω Ω d d d d k N 1 • Let’s evaluate the multiplicative factor P. Piot, PHYS 571 – Fall 2007
Multiparticle Coherence III • We have. 2 ∑ ∑ ∑ ∑∑ − ωδ − ωδ i t i t − ωδ + ωδ + ωδ = = + i t i t i t e e e N e e j j k k ≠ j j k j k j • Introducing the line charge density Λ (t) we can write 2 ~ ∑ − ωδ = + − Λ ω i t e N N ( N 1 ) ( ) j ~ ≈ + Λ ω 2 N N ( ) Fourier transform of Typically N>>1 the line charge density P. Piot, PHYS 571 – Fall 2007
Multiparticle coherence IV BBF measurement (easy!) can provide information on the bunch longitudinal charge distribution 10 13 100000 90000 10 12 80000 10 11 Population 70000 BFF (a.u.) 10 10 60000 10 9 50000 40000 10 8 30000 10 7 20000 10 6 10000 10 5 0 10 -5 10 -4 10 -3 10 -2 10 -1 10 0 10 1 10 2 -5.0 -2.5 0.0 2.5 5.0 100000 10 13 90000 10 12 80000 10 11 Population 70000 BFF (a.u.) 10 10 60000 10 9 50000 40000 10 8 30000 10 7 20000 10 6 10000 10 5 0 10 -5 10 -4 10 -3 10 -2 10 -1 10 0 10 1 10 2 -5.0 -2.5 0.0 2.5 5.0 10 13 100000 90000 10 12 80000 10 11 Population 70000 BFF (a.u.) 10 10 60000 10 9 50000 40000 10 8 30000 10 7 20000 10 6 10000 10 5 0 10 -5 10 -4 10 -3 10 -2 10 -1 10 0 10 1 10 2 -5.0 -2.5 0.0 2.5 5.0 z/ / z z P. Piot, PHYS 571 – Fall 2007
Multiparticle coherence V Example of real measurement… 4 2 (A) (B) Autocorrelation (a.u.) Interferogram (a.u.) µ 3 1 110 m 2 0 1 −1 −1000 0 1000 −1000 0 1000 Mirror Position (microns) MIrror Position (microns) 60 2 (C) (D) 50 Bunch Population (a.u.) Power Spectrum (a.u.) 1.5 Low Frequency Extrapolation 40 1 30 0.5 Deduced Spectrum 20 0 10 0 −0.5 0 100 −0.1 −0.05 0 0.05 0.1 Wavenumber (1/cm) s (mm) P. Piot, PHYS 571 – Fall 2007
Multi-particle coherence: example of CSR Coherent Synchrotron Radiation Beam pipe CSR enhancement induced frequency cut-off SR P. Piot, PHYS 571 – Fall 2007
Recommend
More recommend