c
play

c + = Diffusion Diffusion 2 6.82 10 -6 v c D c 10 - PowerPoint PPT Presentation

Feb 25, 2024 •417 likes •515 views

! Diffusivity ! Diffusivity ! Diffusion Equation ! Diffusion Equation ! Diffusion to a Wall ! Diffusion to a Wall ! Deposition Velocity, Diffusion ! Deposition Velocity, Diffusion Boundary Layer, Diffusion Force Boundary Layer, Diffusion

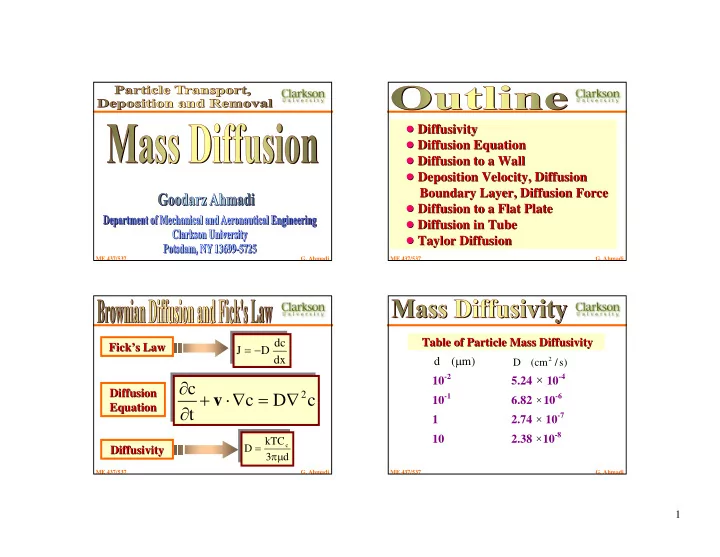
  1. ! Diffusivity ! Diffusivity ! Diffusion Equation ! Diffusion Equation ! Diffusion to a Wall ! Diffusion to a Wall ! Deposition Velocity, Diffusion ! Deposition Velocity, Diffusion Boundary Layer, Diffusion Force Boundary Layer, Diffusion Force ! ! Diffusion to a Flat Plate Diffusion to a Flat Plate ! Diffusion in Tube ! Diffusion in Tube ! Taylor Diffusion ! Taylor Diffusion ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi Table of Particle Mass Diffusivity dc Table of Particle Mass Diffusivity Fick’s Law Law = − Fick’s J D µ dx d ( m ) 2 D ( cm / s ) 5.24 × 10 -4 10 -2 ∂ c + ⋅ ∇ = ∇ Diffusion Diffusion 2 6.82 × 10 -6 v c D c 10 -1 ∂ Equation Equation t 2.74 × 10 -7 1 2.38 × 10 -8 10 kTC = c D Diffusivity Diffusivity πµ 3 d ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 1

  2. s 2 = Mean Square Mean Square Effect of Effect of 2 Dt Displacement Displacement Particle Mass Particle Mass = − τ − − τ Brownian Motion Brownian Motion 2 t / 2 kT s 2 Dt [ 1 ( 1 e ) / t ] θ = 2 t of Rotation of Rotation πµ 3 d Particle Mean 2 = Particle Mean Particle Fluctuation Particle Fluctuation 1 3 u 2 = m u kT Energy Energy 3 kT / m Free Path Free Path 2 2 λ α ≈ τ π 8 kT / m Concentration in − Concentration in mg ( x x ) = − 0 C C exp{ } gravitational field gravitational field 0 kT ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi y ∂ ∂ 2 c c ∂ ∂ ∂ η ∂ − ∂ η c c c y c = = = = − D = C ( y , 0 ) C ∂ ∂ ∂ ∂ η ∂ ∂ η ∂ η 2 t 0 t t 2 t y 2 t 4 Dt Similarity Equation Similarity Equation Similarity Variable Similarity Variable = C ( 0 , t ) 0 2 2 + dc d c dc y = − η + η = η = ln( ) ln A 2 0 η η η 2 d d d 4 Dt ∂ ∂ ∂ η ∂ ∂ ∂ 2 2 c c c 1 c c 1 dc = ∫ η = = = = − η 2 − η 2 η + Ae c A e d B 1 ∂ ∂ η ∂ ∂ η ∂ ∂ η η 2 2 1 y 4 Dt d y y 4 Dt 0 ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 2

  3. η 1.0 tD=0.0025 tD=0.062 η → ∞ = C ( ) C tD=0.25 = 0 0.8 C ( y , t ) C erf ( y / 4 Dt ) tD=1 0 0.6 C/Co tD=4 0.4 η = = 0.2 C ( 0 ) 0 0.0 ξ 2 ∫ 0 0.5 1 1.5 2 2.5 3 ξ = − ξ 2 ξ erf ( ) e d = ∞ = x π y erf ( 0 ) 0 erf ( ) 1 0 Variation of concentration profile with time. Variation of concentration profile with time. ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi Number Deposited Number Deposited J D D D Diffusion Diffusion = = = = = dN Jdt C dt u π 0 in Time dt in Time dt t Velocity π δ Velocity D C t 0 c Diffusion Diffusion δ = π Dt Boundary Boundary c 4 Dt = Layer Layer Total Deposited in Total Deposited in N C π 0 Time Interval (0,t) Time Interval (0,t) Diffusion Diffusion = πµ F 3 du / C Force Force d D c ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 3

  4. U C C U C 4 DL in = − out out 1 Concentration Ratio Concentration Ratio π 2 R C uR in Detailed Analysis Detailed Analysis 4 DL 4 Dt t = = = L / u N C N C π π in C 0 u = − φ + φ + φ out 2 / 3 4 / 3 1 2 . 56 1 . 2 0 . 177 C in π 2 R L − = − C C N DL φ = out in π 2 R L 2 uR ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi C o U o U o C o ∂ ∂ ∂ 2 u u u + = ν u v Momentum ∂ ∂ 2 x y dy y δ ∂ ∂ u v Mass + = 0 ∂ ∂ x y δ c x = = = y = u v c 0 0 ∂ ∂ ∂ 2 c c c + = Concentration u v D ∂ ∂ ∂ 2 → ∞ x y y = = y u U , c c 0 0 ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 4

  5. = = ∞ = u f ( 0 ) f ' ( 0 ) 0 f ' ( ) 1 = η U Boundary Boundary f ' ( ) η = 0 y U ν Conditions Conditions x 0 = ∞ = c ( 0 ) 0 c ( ) c 0 ψ = ν η = η U o x f ( ) c c ( ) ν Blasius Blasius x = γ = δ = 5 f ' ' ( 0 ) 0 . 332 Solution Solution U 0 Momentum/Mass Concentration γ 2 + + = Near the Near the η ff ' ' 2 f ' ' ' 0 1 + = ν f ~ ... c " S fc ' 0 = S c Plate Plate c 2 2 D Blasius Equation ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 1.0 η ∫ − γ 3 γ C exp( s z ) dz 0 1 c γ = 0.8 = 0 C 1 ∞ 12 ∫ − γ 0.6 3 exp( s z ) dz C/Co 1 c 0 0.4 0.2 γ η s 3 c ∫ = − γ 0.0 1 c 3 [exp( s z )] dz 0 0.5 1 1.5 2 2.5 3 1 c c 0 . 89 z 0 0 ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 5

  6. γ δ c ⎡ ∂ ⎤ 3 ( s ) c U U = = = 1 c 0 0 J D ⎢ ⎥ Dc 0 . 34 Dc 3 s ∂ 0 0 c ⎣ ⎦ y 0 . 89 vx vx u = y 0 c z r R y δ Dc 3 vx 0 . 6 Diffusion Diffusion δ = ≈ ≈ 0 c J U Boundary Layer 3 s 3 s Boundary Layer 0 c c 2 Laminar Laminar r = − = − u u ( 1 ) y R r Flow Flow 0 2 = ∫ L R Total Total U L = = I Jdx 0 . 68 Dc s R o 3 R Diffusion Diffusion 0 c eL eL ν 0 2 y + u ~ u ... 0 R ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi ∂ ∂ η 2 − 2 u c c Diffusion 2 Diffusion Concentration Concentration ∫ = η η 3 0 c exp{ } d y D Equation Equation 0 1 1 ∂ ∂ 9 Profile 2 Profile R x y = 0 c ∞ − 2 ∫ η η 3 exp{ } d 1 1 9 u y Similarity Similarity Boundary Condition Boundary Condition η = 0 0 Wall Flux Wall Flux 3 Variable Variable 3 c = DR x y = 0 0 Dc u 0 0 3 ⎡ ∂ ⎤ c 3 x DR u = = J D ⎢ ⎥ J = → ∞ 2 = 0 ∂ ∞ 0 . 67 c D y 2 3 ⎣ y ⎦ c = 2 ∫ − η η + η = 3 0 c y 0 exp( ) d DRx c ' ' c ' 0 1 1 9 0 0 3 ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 6

  7. ∂ ∂ ∂ ∂ ∂ 2 2 c c c 1 c c + = + + Total Flux L 2 Total Flux u L u ( r ) D ( ) ∫ = π = π 0 I 2 R Jdx 2 . 01 c DR 3 ∂ ∂ ∂ ∂ ∂ 2 2 t x r r r x 0 DR 0 2 r Diffusion Diffusion = − 3 2 2 Neglecting Axial Neglecting Axial Dc R x 1 R x u ( r ) 2 U ( 1 ) δ = = = 0 Boundary Boundary 2 3 R Diffusion Diffusion c 1 / 3 1 / 3 J 0 . 67 s R 0 . 67 s R layer layer c eR c eR ∂ ∂ ∂ ∂ 2 2 c 2 r c c 1 c + − = + u R U ( 1 ) D ( ) = 0 R ∂ ∂ ∂ ∂ 2 2 ν t R x r r r e R ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi [ ] = ⎡ ∂ ⎤ R R ∂ c ≈ ∂ ∂ c c 1 1 2 c ∫ ∫ ∫ c c = = = π = = Moving Moving = = o r 0 c cdA 2 rcdr crdr 0 ⎢ ⎥ const For For 0 π 2 2 ∂ A ⎣ ⎦ Frame Frame ∂ ∂ R R ∂ r x x t A 0 0 R Concentration Concentration ∂ ∂ ∂ 2 2 2 r c c 1 c Diffusion Diffusion − = + ∂ U ( 1 ) D ( ) In Moving In Moving 2 2 4 R U c 1 r 1 r ∂ ∂ ∂ = + − + − 2 2 Frame Frame R x r r r c c ( ) ∂ 2 4 4 D x 3 R 2 R ∂ 2 2 4 ∂ UR c r 1 r R 2 2 R U c = + − Total Total ∫ Solution Solution = π − = − π c c ( ) 2 Q 2 c ( u U ) rdr ( R ) ∂ o ∂ 2 4 c 4 D x R 2 R Flux Flux 48 D x 0 ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 7

  8. ∂ 2 2 Q R U c Flux Per Flux Per = = − J ( ) t = N π ∂ 2 = 2 δ Unit Area Unit Area R 48 D x 0 c ( x ) π R 2 2 R U Effective (Taylor) Effective (Taylor) eff = D Diffusivity Diffusivity 48 D − ∂ c ≠ ∂ ∂ ∂ 2 2 c c 1 N 1 ( x Ut ) = − = = − const . J D c exp{ } ∂ ∂ ∂ ∂ eff 2 x t x x π 2 π 2 R 4 D t D t eff eff L 2 UR >> e >> = = P R S Range of Validity P 14 Range of Validity e e c D R ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi ! Mass diffusion decreases with size ! Mass diffusion decreases with size 2.0 1.8 1.6 ! Diffusion Boundary Layer is ! Diffusion Boundary Layer is 1.4 1.2 C/Co generally smaller that momentum generally smaller that momentum 1.0 0.8 boundary layer boundary layer 0.6 0.4 0.2 ! Convective diffusion in a tube ! Convective diffusion in a tube 0.0 0 1 2 3 4 ! ! Taylor diffusion in a tube Taylor diffusion in a tube x Variations of concentration along the tube at different times. Variations of concentration along the tube at different times. ME 437/537 G. Ahmadi ME 437/537 G. Ahmadi 8

Recommend

More recommend