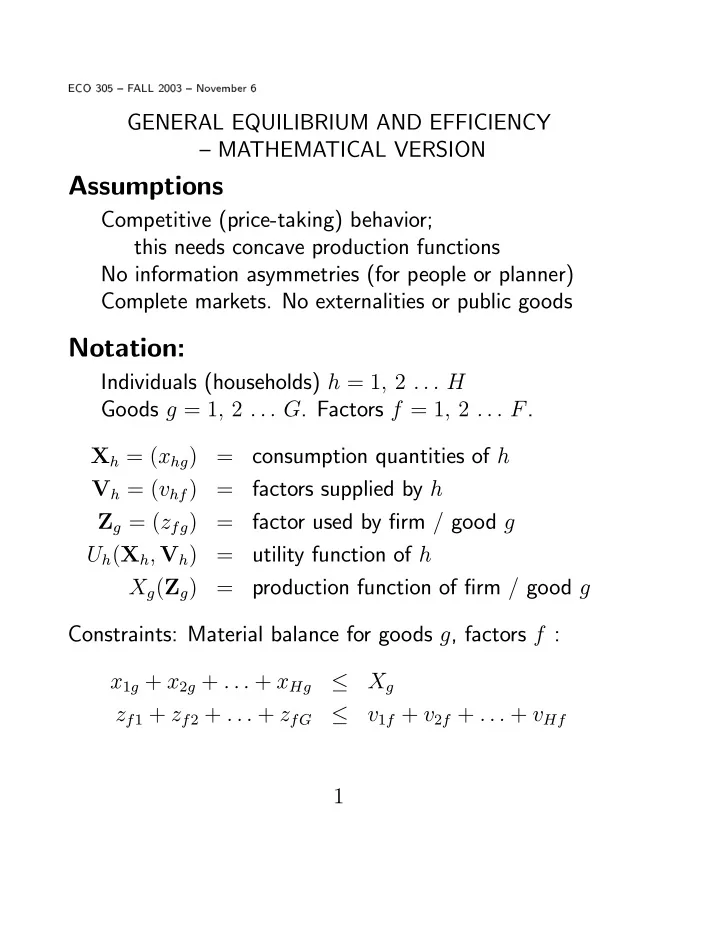

ECO 305 — FALL 2003 — November 6 GENERAL EQUILIBRIUM AND EFFICIENCY — MATHEMATICAL VERSION Assumptions Competitive (price-taking) behavior; this needs concave production functions No information asymmetries (for people or planner) Complete markets. No externalities or public goods Notation: Individuals (households) h = 1 , 2 . . . H Goods g = 1 , 2 . . . G . Factors f = 1 , 2 . . . F . X h = ( x hg ) = consumption quantities of h V h = ( v hf ) = factors supplied by h Z g = ( z fg ) = factor used by firm / good g U h ( X h , V h ) = utility function of h X g ( Z g ) = production function of firm / good g Constraints: Material balance for goods g , factors f : x 1 g + x 2 g + . . . + x Hg X g ≤ z f 1 + z f 2 + . . . + z fG v 1 f + v 2 f + . . . + v Hf ≤ 1
Optimization Social objective (Pareto efficiency + interpersonal weights) W = W ( U 1 , U 2 . . . U H ) � � G H F H G � � � � � L = W + α g X g − x hg + β f v hf − z fg g =1 g =1 h =1 f =1 h =1 FONCs for interior optimum: ∂W ∂U h − α g = 0 ∂U h ∂x hg ∂W ∂U h + β f = 0 ∂U h ∂v hf ∂X g α g − β f = 0 ∂z fg Relate to Edgeworth Box geometry of Nov. 4 handout: ∂U h /∂x h 1 α 1 Exchange (Fig. 1) = for all h ∂U h /∂x h 2 α 2 Factor alloc (Fig. 3) ∂X g /∂z 1 g β 1 = for all g ∂X g /∂z 2 g β 2 Combined (Fig. 4) − ∂U h /∂v hf β f = ∂X g = for all h ∂U h /∂x hg α g ∂z fg 2
Equilibrium p = ( p g ) , w = ( w f ) vectors of prices of goods factors FIRMS F � Profit function π g ( p g , w ) = max p g X g ( z 1 g , . . . z Fg ) − w f z fg f =1 X g = ∂π g /∂p g , z fg = − ∂π g /∂w f HOUSEHOLDS p · X h ≤ w · V h + I h x hg = x hg ( p , w , I h ) , v hf = v hf ( p , w , I h ) G � I h = θ hg π g ( p g , w ) g =1 EQUILIBRIUM H G � � = X g ( p g , w ) x hg p , w , θ hg π g ( p g , w ) h =1 g =1 G H G � � � z fg ( p g , w ) = v hf p , w , θ hg π g ( p g , w ) g =1 h =1 g =1 3
Existence System has ( F + G − 1) unknowns and equations: (1) All quantitites homogeneous degree zero in ( p , w ) Only relative prices matter Can use freedom to set any one p g or w f equal to 1 Then all prices measured in units of this good or factor It is called numeraire — can be composite bundle (2) Walras’ Law - at all ( p , w ) (equil. or not) total value of excess demands is ≡ 0 � � G H F G H � � � � � p g x hg − X g + w f z fg − v hf g =1 g =1 h =1 f =1 h =1 H G F G F � � � � � p g X g − = p g x hg − w f v hf − w f z fg h =1 g =1 f =1 g =1 f =1 H G � � = I h − π g ≡ 0 h =1 g =1 Existence of solution proved by fixed point theorem Uniqueness, dynamic stability not guaranteed 4
Equivalence In equilibrium, consumers’ and firms’ FONCs are ∂U h ∂U h − λ h p g = 0 , + λ h w f = 0 ∂x hg ∂v hf ∂X g p g = w f ∂z fg Same as Lagrange conditions of social optimum if ∂W/∂U h = 1 /λ h with α g = p g , β f = w f So equilibrium is an optimum with particular social weights It is “Pareto efficient” Conversely, social optimum with planner’s weights can be implemented as equilibrium if lump sums can be transfered between people to make λ h = 1 / ( ∂W/∂U h ) 5
Uses of general equilibrium analysis (1) Emphasizes linkages throughout economy (2) Clarifies conditions needed for optimality of markets perfect competition, no externalities or public goods complete markets, no information asymmetries No guarantee of ethically good distribution of income (3) Extensions to allow externalities, public goods can find optimal policy intervention (4) Clarifies limitations of planning benevolent dictator with complete information (5) Broad interpretation (a) equilibrium over time: p t − 1 /p t = 1 + r borrowing and lending, investment, R-and-D (b) uncertainty: claims to $ in different “scenarios” trading risk, insurance, finance Other extensions/modifications more drastic (a) Oligopoly — strategic behavior, game theory (b) Asymmetric information — signaling, screening incentive schemes, contracts and organizations 6
Recommend
More recommend