


Announcements 1 st Midterm and Homeworks #1 and #2 will be returned this • week in workshops Discussion of 1 st exam results Monday, Oct 13 th , after Prof. y, , • McFarland is back from Japan 2 nd Midterm Exam is 23 October 8:00-9:20am Hubbell 2 Midterm Exam is 23 October, 8:00 9:20am, Hubbell • HW#5 due Wednesday Oct 15 th 11:30 • Preflights #10 and #11 will be posted later this week • – due Monday and Wednesday, 7am, respectively – everything with workshops also back on the normal schedule this week
Questions “AWESOME job using the chalk board to do examples. • Please keep doing this! keeps people awake and makes us follow along sicne we write it down” – I will keep trying to do this. It’s a little uncomfortable for me b because of the awkwardness of the boards. f h k d f h b d – “can you please not make HW sets due Tuesdays? The ChemE students in the class all have 2 other major problem sets due Tuesdays and this would just push us over the t d T d d thi ld j t h th edge” – I will try to keep them on Wednesday, but I need better participation in the Wednesday preflights participation in the Wednesday preflights – If you think of other ways I can achieve that, please let me know. I’m open to other ideas. – “You have to love baseball!!! Didn't your party school U You have to love baseball!!! Didn t your party school U. – Chicago teach you anything about loving the sport?” – Nope. The Brewers’ spectacular collapse doesn’t help either. – Personal note from Prof. McFarland: thank you so much for Personal note from Prof McFarland: thank you so much for not mentioning football in the comments this week. Oy!
Leftover: Lecture 8, Concept 2 + Q • Two parallel plate capacitors are identical κ κ (same A , same d ) except that C 1 has half of (same A , same d ) except that C 1 has half of C C 1 E 1 =? E =? the space between the plates filled with a material of dielectric constant κ as shown. - Q – If both capacitors are given the same + Q + Q amount of charge Q , what is the relation E 2 =? C 2 between E 1 , the electric field in the air of - Q C 1 , and E 2 , the electric field in the air of C 2 (1) E 1 < E 2 (2) E 1 = E 2 (3) E 1 > E 2 • The key here is to realize that the electric field in the air in C 1 must be • The key here is to realize that the electric field in the air in C must be equal to the electric field in the dielectric in C 1 !! • WHY? • Plates are conductors; therefore they are equipotential surfaces. • For this to happen, the charge density on each plane must be non- F thi t h th h d it h l t b uniform to create equal electric fields!! • Another way (microscopic view): polarized dielectric attracts charge from conductor, thus moving charge from air region of C 1 • Consequently, E 1 < E 2 . • So V 1 < V 2 , and thus C 1 > C 2 . (A reasonable guess!)
Today… • Current and How Current Works Current and How Current Works • Devices – Batteries – Resistors • Ohm’s Law • Power in Resistors ( • Power in Resistors (probably next lecture) b bl t l t ) Text Reference: Chapter 25:1-8 Examples: 25. 1,3,5,6,10
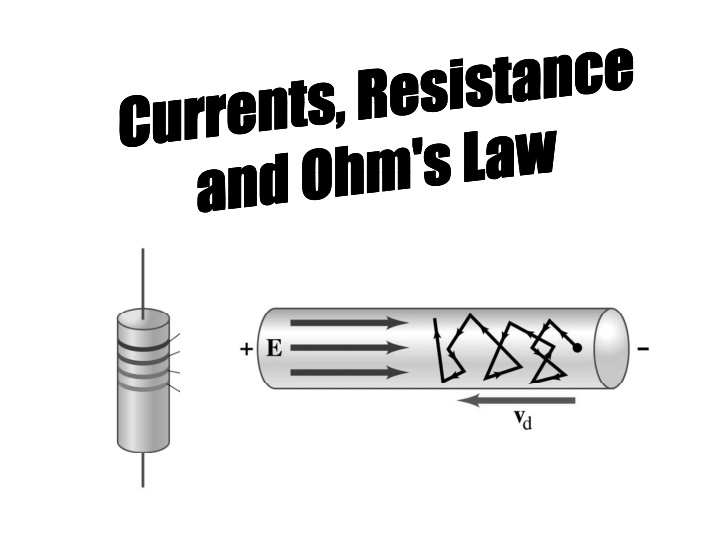
Current is charge in motion • Charge, e.g. electrons, exists in conductors C with a density, n e ( n e approx 10 23 cm -3 ) • “Somehow” put that charge in motion: Somehow put that charge in motion: • – effective picture – some net charge flows with an average velocity per charge carrier, v d – more on how this happens in a minute! • Current density, j , is given by j = q e n e v d C t d it j i i b j – unit of j is C/m 2 sec or A/m 2 (A = Ampere) and 1A = 1C/s – current, I , is j times cross sectional area, I = j π r 2 – for 10 Amp in 1mm x 1mm area, j =10 +7 A/m 2 , and v d is about 10 -3 m/s
Circuit Elements • Capacitors: Purpose is to store charge (energy). We have calculated the capacitance of a system • We had to modify Gauss' Law to account for bulk • matter effects (dielectrics) … C = κ C 0 We calculated effective capacitance of series or p • parallel combinations of capacitors • Batteries (“Voltage sources”): + + - Purpose is to provide a constant OR potential difference between 2 points. Electrical ↔ chemical energy conversion • V - + For now, we will think of them as an ideal • source of voltage Later: Non-ideal batteries will be dealt with • in terms of an "internal resistance".
Circuit Elements (Cont’d) • Resistors: R i Purpose is to limit current drawn in a circuit. p dQ = Note: UNIT: Ampere = A = C/s I dt dt Resistance can be calculated from knowledge of the • geometry of the resistor AND the “resistivity” of the material out of which it is made. The effective resistance of series and parallel • combinations of resistors will be calculated using Kirchhoff's Laws (which are really just statements about potential difference and current conservation). “Conductors” as we have considered them before, • have resistance!
Defining Resistance R R Resistance Resistance • I I Resistance is defined to be the ratio of the applied voltage to the current passing through. the current passing through. V V R ≡ UNIT: OHM = Ω I • Is this a good definition? i.e., does the resistance belong only to the resistor? Recall the case of capacitance: ( C = Q / V ) depended on the geometry, not on Q or V individually g y, y Q Does R depend on V or I ? It seems as though it should, at first glance...
PF09 Question #2 C Consider two circuits, each with a load resistor R i id i i h i h l d i R V R driven by a battery with voltage V i . V 1 =5 Volts, V 2 =20 Volts, R 1 =10 Ω , R 2 =50 Ω. , , 2 1 2 Compare the currents through the two resistors. 100% a) I 1 > I 2 b) I 1 = I 2 c) I 1 < I 2 80% 95% 60% 40% 40% 20% 0% 3% 3%
What you said … V V R R What I would have said What I would have said … I=V/R. I 1 =5V/10 Ω , I 2 =20V/50 Ω Ri h Right: Ohm's law says that I=V/R. I1= 5/10 > I2= 20/50 For question 2 I = V/R and for question 4 P = IV. Equation roulette :) [ok, you win this time… care to try your luck again?] Wrong: Current is the flow of electric charge. Resistors reduce the flow of charge, and the battery provides the charge. Although there is less charge provided by V1, the ratio of Voltage/Resistance for V1/R1 is larger than V2/R2. So despite the fact that more charge is flowing from V2, R2 is reducing the charge much more. So I1>I2. [Careful not to confuse charge flow with voltage. Voltage is just a potential difference… tells you how much higher energy of charge leaving battery is than charge returning. #2 has smaller CURRENT at higher VOLTAGE] The current for circuit 1 is higher because the battery is working against a smaller resistance [Right answer, but… current is also proportional to V. More V means more force on charge carriers]
R Resistance and Currents I I Let’s analyze the flow of charge y g • through a resistor… – If there is a potential difference inside V the resistor, then there is also…? – A constant electric field Constant electric field implies • what force on charge carriers? g – Constant, and therefore a constant acceleration! If acceleration of charge carriers occurs, then how does g , • current vary with time? – Well, it should increase linearly with time, like velocity does under constant acceleration, right? This isn’t what we observe… • – We observe that current is proportional to voltage and doesn’t change over long period of time – Let’s understand this…
Resistance and Currents (cont’d) • Charges are put in motion, but scatter in a very short time from things that get in the way – it’s crowded inside that metal – defects, vibrational motion of atoms (phonons), etc 1 Typical scattering time τ = 10 -14 sec • charges accelerated for this time and then randomly scattered charges accelerated for this time and then randomly scattered • average velocity attained in this time is v = a τ = eE τ / m • current density, j = en e v , so current is proportional to E which is • proportional to Voltage proportional to Voltage OHM’s LAW j = ( e 2 n e τ / m ) E ( e 2 n e τ / m = σ , called conductivity) •
Lecture 9, Concept 1 • Which analogy would you use to explain flow of charge in a resistor in response to an potential difference? Why? (1) S (1) Snow falling outside your window f lli t id i d (2) A car rolling down a hill towards a wall (3) Molecules of a scent traveling through the air traveling to your nose
Lecture 9, Concept 1 • Which analogy would you use to explain flow of charge in a resistor in response to an potential difference? Why? (1) S (1) Snow falling outside your window f lli t id i d (2) A car rolling down a hill towards a wall (3) Molecules of a scent traveling through the air traveling to your nose • The snow is falling at an apparently constant velocity despite the constant acceleration due to gravity because of microscopic collisions with air molecules in its path collisions with air molecules in its path • The car rolls down the hill in response to a constant acceleration, and increases in speed until a catastrophic collision at the bottom. • The scent molecules diffuse through the air, but they diffuse g , y uniformly.
Recommend
More recommend