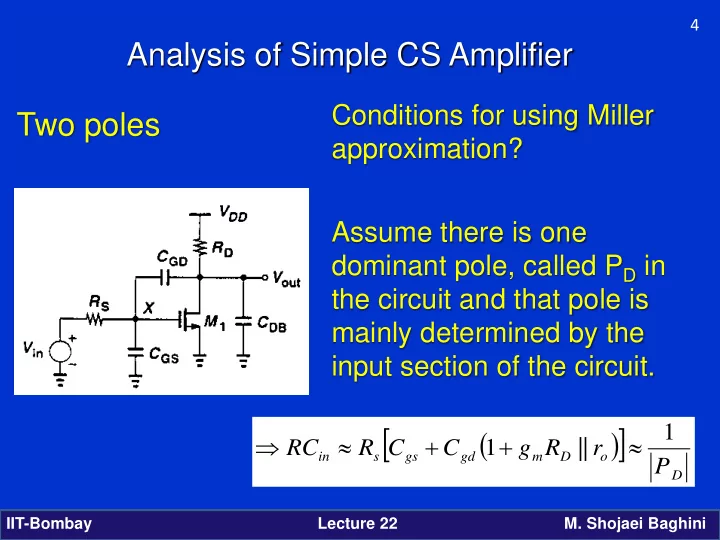

4 Analysis of Simple CS Amplifier Conditions for using Miller Two poles approximation? Assume there is one dominant pole, called P D in the circuit and that pole is mainly determined by the input section of the circuit. [ ] 1 ( ) ⇒ ≈ + + ≈ 1 || RC R C C g R r in s gs gd m D o P D IIT-Bombay Lecture 22 M. Shojaei Baghini
5 Analysis of Simple CS Amplifier (cont’d) Miller approximation: 1 1 ≈ ≈ [ ] P ( ) + + D 1 || RC R C C g R r in s gs gd m D o Assume node X is almost short to AC ground at frequencies close to the second pole (|P 2 |): 1 1 ⇒ ≈ ≈ [ ] P 2 + || RC R r C C out D o db gd [ ] [ ] ( ) + << + + : || 1 || Assumption R r C C R C C g R r D o db gd s gs gd m D o >> , 1 A v IIT-Bombay Lecture 22 M. Shojaei Baghini
6 Analysis of Simple CS Amplifier (cont’d) Even if the assumption is valid it doesn’t mean the transfer function is an all-pole transfer function. NOTE: Miller approximation removes the zero. How to calculate the zero? IIT-Bombay Lecture 22 M. Shojaei Baghini
7 Analysis of Simple CS Amplifier with Miller Approximation - Example mA = Ω = µ = 5 , 200 , 1 . 1 R k I A g D D m V = = Ω 50 r R k o s = = = 10 , 16 , 112 C fF C fF C fF gd db gs ( ) ≈ × = || 1 . 1 5 5 . 5 g R r m D o + = + × = 6 . 5 112 6 . 5 10 177 C C fF gs gd = ⇔ 113 ( / ) 18 P Mrad s MHz × = 50 177 8 . 85 D k fF ns = ⇔ 7 . 7 ( / ) 1 . 2 P Grad s GHz × = 2 5 26 0 . 13 k fF ns = ⇔ . . 68 . 75 ( / ) 10 . 9 R H Z Grad s GHz << ( 0 . 13 8 . 85 ) ns ns IIT-Bombay Lecture 22 M. Shojaei Baghini
8 Analysis of Simple CS Amplifier – Exact Transfer Function ( ) − ( ) v s C s g R = out GD m D ( ) v s 3 3 1 [ ] 1 ( ) ( ) ∑ ∑ + + + + + + in 2 1 R R C C s R g R C R C R C C s S D i j S m D GD S GS D GD DB 2 = = 1 1 j i ≠ = = = , , , i j C C C C C C 1 2 3 GS DB GD [ ] ( ) ( ) + + + + = 1 R g R C R C R C C s S m D GD S GS D GD DB [ ] s ( ) + + + + C R R g R R R C R C GD S D m S D S GS D DB IIT-Bombay Lecture 22 M. Shojaei Baghini
9 Analysis of Simple CS Amplifier – Exact Transfer Function (cont’d) = = = , , C C C C C C 1 2 3 GS DB GD ≠ ( ) i j Denominator: 3 3 1 [ ] ( ) ∑ ∑ + + + + + + = 2 1 R R C C s R R g R R C R C R C s S D i j S D m S D GD S GS D DB 2 = = j 1 i 1 2 1 1 s s s + + = + + + 1 1 1 s p p P P P P 1 2 1 2 1 2 1 1 << ⇒ >> If P P 1 2 P P 1 2 1 1 ⇒ ≈ = P ( ) 1 + + + + 3 R R g R R C R C R C ∑ τ S D m S D GD S GS D DB i = i 1 IIT-Bombay Lecture 22 M. Shojaei Baghini
10 Analysis of Simple CS Amplifier – Example of large R S C GS 1 ≈ 1 p [ ] ( ) Large R S : + + 1 R g R C C S m D GD GS [ ] ( ) + + 1 g R C C ≈ m D GD GS 2 p ( ) + + R C C C C C C D GD GS DB GS GD DB Large C GS 1 ≈ 1 P R C S GS 1 ≈ 2 P ( ) + R C C D GD DB IIT-Bombay Lecture 22 M. Shojaei Baghini
11 Analysis of Source Follower R L R L Effect of load resistance and g mb is shown by R L . + 1 C s GS 1 + 1 ( ) g g R v s = m m L out ( ) + ( ) v s 3 3 1 R R R R C C ∑ = ∑ × + + + + in 2 S L S L GS L 1 C C s R C s − + + i j S GD + 1 1 2 1 g R g R R g = 1 1 j i m L m L L m ≠ = = = , , , If R L →∞ ? i j C C C C C C 1 2 3 GS L GD IIT-Bombay Lecture 22 M. Shojaei Baghini
Recommend
More recommend