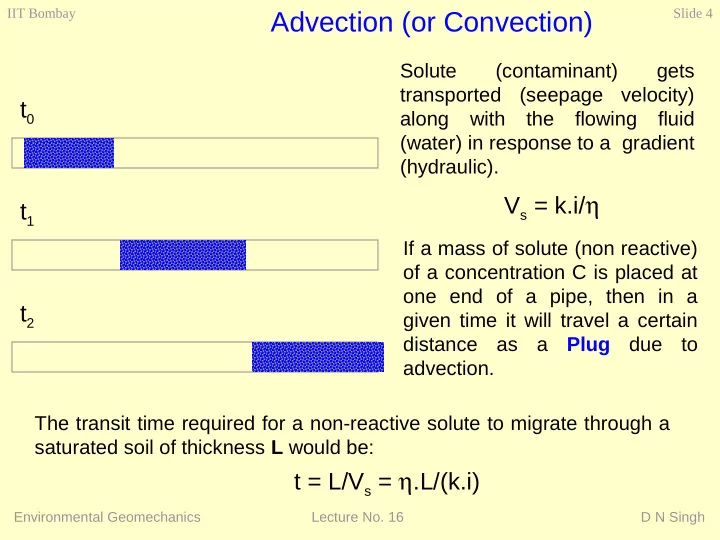

IIT Bombay Slide 4 Advection (or Convection) Solute (contaminant) gets transported (seepage velocity) t 0 along with the flowing fluid (water) in response to a gradient (hydraulic). V s = k.i/ η t 1 If a mass of solute (non reactive) of a concentration C is placed at one end of a pipe, then in a t 2 given time it will travel a certain distance as a Plug due to advection. The transit time required for a non-reactive solute to migrate through a saturated soil of thickness L would be: t = L/V s = η .L/(k.i) Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay Slide 5 Representative values for effective porosity η Description Soils GC, GP, GM,GS 0.20 SW,SP,SM,SC - ML, MH 0.15 CL,OL, CH, OH, PT 0.01 Rocks Non fractured rocks 0.15 Fractured rocks 0.0001 The advective mass flux, J, (or the mass flowing through a unit cross sectional area in a unit of time) is: J =v.C=k.i.C C = concentration of the solute (i.e., the mass of solute per unit volume of the mixture). Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay Slide 6 Diffusion Contaminant at Contaminant • Solutes (contaminants) migrate due to concentration concentration = 0 their chemical activity in the absence of C 0 at t 0 at t 0 bulk fluid flow. • From higher concentration to lower concentration area. • Difference in contaminant concentration is the concentration gradient. Sample • Diffusion ceases when concentration gradient becomes negligible. 1.0 • Relative contaminant concentration C t /C 0 < 1.0 C t / C 0 0.5 =C t /C 0 • Time after introduction of contaminant 0 = t t o t Environmental Geomechanics Lecture No. 16 D N Singh
Diffusion IIT Bombay Slide 7 • Add small amount of dye in a fluid • Pulse gets spread out Add continuous dye-- a sharp front Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay Slide 8 Types of Diffusion • Steady State Diffusion J D =-D. η .( ∆ C/ ∆ x) • Diffusion flux constant with time • Fick’s First law applicable D = diffusion coefficient [L 2 /T] η = porosity ∆ C/ ∆ x = concentration gradient (i.e., change in concentration with distance) • Non Steady-state Diffusion ( ) ( ) ∂ ∂ ∂ C x , t C x , t • Concentration gradient non-uniform = D • Follows Fick’s second law ∂ ∂ ∂ t x x Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay Slide 9 Chemical Energy Field • To study the mechanism(s) of contaminant transport – the intact and fractured rock samples (Gurumoorthy 2002) diffusion characteristics of the saturated and unsaturated soils (Rakesh 2005) • Investigations using the Cl - , I +2 , Cs +1 and Sr +2 in their active as well as inactive forms • Development of Diffusion Cell Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay CONTAMINANT TRANSPORT MODELING THROUGH THE ROCK MASS Slide 10 Diffusion cells C 0 C t C t Intact Rock mass (IRM) C o C t Fractured Rock mass (FRM) Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay CONTAMINANT TRANSPORT MODELING THROUGH THE ROCK MASS Slide 11 Diffusion characteristics Diffusion cells N ( ) ( ) ∂ ∂ 2 C x, t C x, t 33 50 75 100 = D α 40 i C 0 C t ∂ 2 ∂ C t x t Fractured rock mass Intact rock mass 30 -4 ) 0 (x10 Intact Rock mass (IRM) 20 t /C C C D a α aL 10 = × − t i t C LV 6V 0 C o 0 0 2000 4000 6000 8000 10000 0 2000 4000 6000 8000 10000 Time (s) s.L.V 6 10 D i = Diffusion time (s) Intact rock mass a C t Fractured rock mass 5 10 y=1.8 4 10 Fractured Rock mass t m =t p .N -2 (FRM) 3 10 y=1.97 2 10 7 min. 50 days 6 m thick FRM 1 10 1 10 100 75 min. 520 days 0.3 m thick IRM N (D i ) m =(D i ) p Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay Slide 12 Modeling Diffusion in soils using impedance spectroscopy (IS) 60 A C U B 60 B ′ A ′ 30 70 Diffusion cell Impedance value of the soil is measured by using LCR meter Diffusion of contaminant can be monitored by determining the change in the impedance of the soil Environmental Geomechanics Lecture No. 16 D N Singh
IIT Bombay Slide 13 • Break-through curve • The slope of the break-through curve diffusion coefficient, D • Archie’s law ( D = ξ . η m ) porosity of the geomaterials Environmental Geomechanics Lecture No. 16 D N Singh
Recommend
More recommend