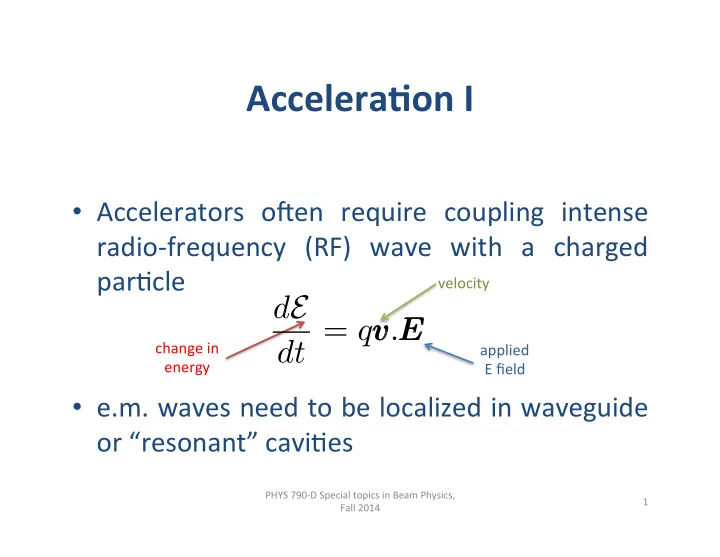
Accelera'on I Accelerators o+en require coupling - PowerPoint PPT Presentation

Accelera'on ¡I ¡ ¡ • Accelerators ¡ o+en ¡ require ¡ coupling ¡ intense ¡ radio-­‑frequency ¡ (RF) ¡ wave ¡ with ¡ a ¡ charged ¡ par=cle ¡ velocity ¡ d E v E dt = qv v.E E change ¡in ¡ ¡ applied ¡ ¡ energy ¡ E ¡field ¡ • e.m. ¡waves ¡need ¡to ¡be ¡localized ¡in ¡waveguide ¡ or ¡“resonant” ¡cavi=es ¡ ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 1 ¡ Fall ¡2014 ¡

radiofrequency ¡(RF) ¡accelera'on ¡ • consider ¡a ¡par=cle ¡ ¡ undergoing ¡accele-­‑ ¡ ra=o ¡ • take ¡resonant ¡pillbox ¡ ¡ cavity ¡opera=ng ¡on ¡TM 010 ¡mode ¡ ¡ E z ( r = 0 , z, t ) = E 0 sin( ω t ) ¡ here ¡ ¡ ¡ ¡ ¡ ¡is ¡the ¡resonant ¡frequency ¡of ¡the ¡mode ¡ ¡ ω PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 2 ¡ Fall ¡2014 ¡

TM ¡modes ¡ • re ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 3 ¡ Fall ¡2014 ¡

TM 010 ¡mode ¡(taking ¡ r<<R ) ¡ • In ¡the ¡paraxial ¡approxima=on: ¡ J 0 ( x ) ' 1 � x 2 J 1 ( x ) ' x 4 2 • so ¡ ¡ E z ( r, φ , z, t ) = E 0 cos( ω t ) E r ( r, φ , z, t ) = 0 PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 4 ¡ Fall ¡2014 ¡

radiofrequency ¡(RF) ¡accelera'on ¡ • prac=cally ¡the ¡field ¡ emission ¡ is ¡wriZen ¡as ¡ ¡ ¡ E z = E 0 sin( ω t + ϕ ) ¡ with ¡ ¡ ¡ ¡ ¡being ¡an ¡ ¡ ϕ arbitrary ¡phase ¡ delay ¡ • we ¡assume ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡when ¡ ¡ ϕ = 0 the ¡par=cle ¡is ¡in ¡the ¡center ¡ master ¡clock ¡ of ¡the ¡cavity. ¡ ¡ ¡ ¡ ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 5 ¡ Fall ¡2014 ¡

Energy ¡gain ¡in ¡a ¡pillbox ¡cavity ¡1 ¡ • carry ¡the ¡=me ¡integral ¡ Z t + δ E = qE 0 β c t − cos( ω t + ϕ ) dt ✓ ω z Z + L/ 2 ◆ = qE 0 cos β ( z ) c + ϕ dz − L/ 2 • we ¡assume ¡the ¡velocity ¡remains ¡constant ¡ during ¡accelera=on. ¡ ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 6 ¡ Fall ¡2014 ¡

Energy ¡gain ¡in ¡a ¡pillbox ¡cavity ¡2 ¡ accelera'ng ¡phase ¡ ¡ • finally: ¡ (or ¡off-­‑crest ¡phase) ¡ δ E = qE 0 L T cos ϕ “DC” ¡ ⇣ ⌘ ω L sin T β c T = ω L β c transit-­‑'me ¡factor ¡ ω L PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 7 ¡ β c Fall ¡2014 ¡

Radial ¡effects ¡ ¡ • Note ¡we ¡assumed ¡pencil ¡beam ¡ • in ¡prac=ce: ¡ – beam ¡has ¡a ¡transverse ¡extent ¡ – beam ¡has ¡a ¡longitudinal ¡(temporal) ¡extent ¡ • radial ¡extend ¡leads ¡to ¡varia=on ¡in ¡accelera=on ¡ across ¡the ¡beam ¡transverse ¡direc=on: ¡ 1 − r 2 ✓ ◆ δ E ( r ) = δ E 4 can ¡be ¡quan=fied ¡by ¡compu=ng ¡an ¡variance ¡in ¡energy… ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 8 ¡ Fall ¡2014 ¡

Effect ¡of ¡non-­‑zero ¡temporal ¡extent ¡ ¡ • temporal ¡extend ¡implies ¡that ¡the ¡bunch ¡ effec=vely ¡sample ¡a ¡range ¡of ¡accelera=ng ¡ ¡ phase ¡ ¡ • so ¡that ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡where ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡is ¡the ¡phase ¡ φ → φ + δφ δφ offset ¡of ¡the ¡considered ¡par=cle ¡within ¡the ¡ bunch ¡(and ¡ ¡ ¡ ¡ ¡is ¡the ¡reference ¡or ¡synchronous ¡ ϕ phase) ¡ ¡ δ E ( δφ ) = qE 0 L T cos( ϕ + δϕ ) so ¡the ¡accelera=on ¡along ¡a ¡bunch ¡is ¡not ¡monoenerge=c… ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 9 ¡ Fall ¡2014 ¡

Limita'on ¡of ¡the ¡pillbox ¡model ¡ • cavi=es ¡have ¡holes ¡for ¡beam ¡to ¡pass ¡through ¡ the ¡cavity, ¡ ideal ¡pillbox ¡ • the ¡axial ¡field ¡does ¡ ¡ not ¡have ¡a ¡hard-­‑edge ¡ ¡ profile ¡and ¡ ¡ ¡ ¡ f ( z ) ¡ E ( z, t ) = E 0 f ( z ) cos( ω t + φ ) ¡ ¡ ¡ ¡ ¡where ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡is ¡the ¡ ¡ f ( z ) ¡ ¡ ¡ ¡spa=al ¡field ¡profile ¡ 2 z/L PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 10 ¡ Fall ¡2014 ¡

How ¡to ¡find ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡? ¡ ¡ f ( z ) • Can ¡apply ¡boundaries ¡condi=ons, ¡ • in ¡essence ¡the ¡aperture ¡lead ¡to ¡diffrac=on ¡of ¡the ¡ field ¡and ¡its ¡leakage ¡outside ¡of ¡the ¡cavity ¡ • As ¡already ¡discussed ¡this ¡leads ¡to ¡a ¡decrease ¡in ¡Q ¡ • In ¡prac=ce ¡the ¡fields ¡ are ¡computed ¡using ¡ ¡ frequency-­‑domain ¡ simula=on ¡“eigen-­‑ ¡ solver” ¡ ¡ ¡ ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 11 ¡ Fall ¡2014 ¡

Transverse ¡fields ¡ ¡ • with ¡the ¡knowledge ¡of ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡ ¡the ¡transverse ¡ f ( z ) field ¡can ¡be ¡es=mated ¡ • assume ¡azimuthally ¡symmetric ¡cavity ¡then ¡ E = 1 d ( rE r ) + 1 dE φ d φ + dE z r E r r .E r dr r dz • so ¡that ¡ f ( z ) E r = − r dE z = − E 0 r d 2 2 dz dz • and ¡likewise ¡for ¡B ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 12 ¡ Fall ¡2014 ¡

Coupled ¡cavi'es ¡ • Mo=va=on: ¡ ¡ – larger ¡accelera=on ¡length, ¡ – distributed ¡power ¡to ¡several ¡resonators ¡(reduce ¡ number ¡of ¡couplers ¡& ¡auxiliary ¡system ¡needed) ¡ • “aZach” ¡several ¡resonators ¡together, ¡the ¡ coupling ¡occurs ¡via ¡cell-­‑to-­‑cell ¡(the ¡coupling ¡ hole ¡has ¡to ¡be ¡large ¡enough) ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 13 ¡ Fall ¡2014 ¡

Modes ¡in ¡a ¡mul'-­‑cell ¡cavity ¡ L • example ¡of ¡a ¡2-­‑cell ¡ ¡ cavity ¡ • can ¡be ¡treated ¡as ¡ ¡ 2 ¡coupled ¡oscillators ¡ D with ¡coupling ¡ ¡ ¡ ¡ ¡ ¡then ¡ ¡ k two ¡eigenfrequencies: ¡ ¡ ω 0 TM 010 , 0 ω 1 = 0 ¡mode; ¡oscillator ¡are ¡in ¡phases ¡ √ 1 + k ω 0 TM 010 , π ω 2 = π ¡mode; ¡oscillator ¡are ¡in ¡phases ¡ √ 1 − k PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 14 ¡ Fall ¡2014 ¡

Analogy ¡with ¡mechanical ¡oscillators ¡ • example ¡ from ¡S. ¡Belomestnykh ¡SRF2005 ¡Ithaca ¡NY ¡ PHYS ¡790-­‑D ¡Special ¡topics ¡in ¡Beam ¡Physics, ¡ 15 ¡ Fall ¡2014 ¡