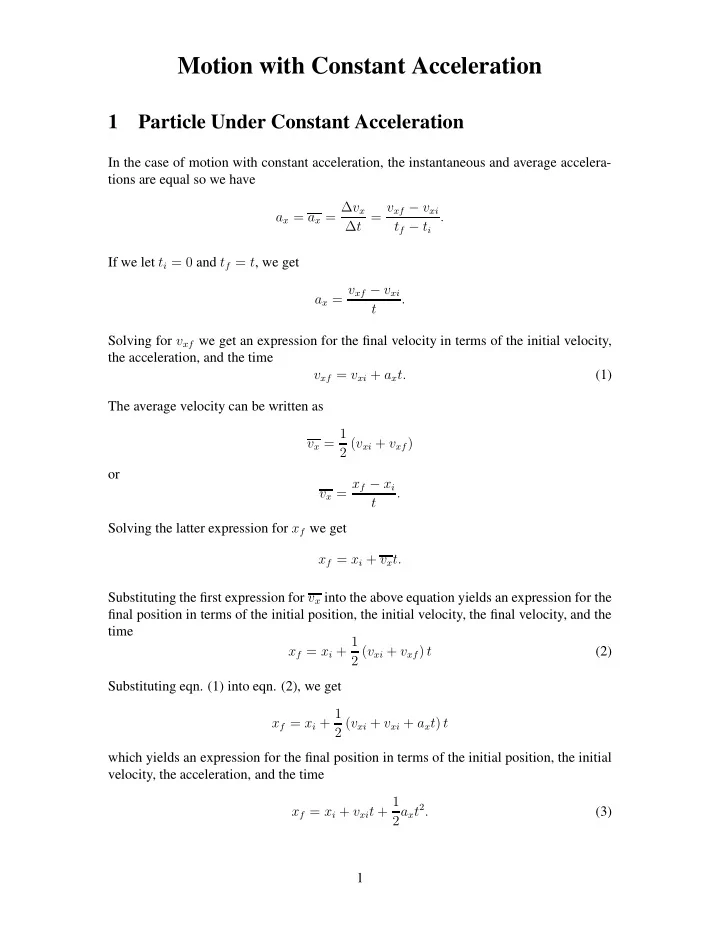
Motion with Constant Acceleration 1 Particle Under Constant - PDF document

Motion with Constant Acceleration 1 Particle Under Constant Acceleration In the case of motion with constant acceleration, the instantaneous and average accelera- tions are equal so we have a x = a x = ∆ v x ∆ t = v xf − v xi . t f − t i If we let t i = 0 and t f = t , we get a x = v xf − v xi . t Solving for v xf we get an expression for the final velocity in terms of the initial velocity, the acceleration, and the time v xf = v xi + a x t. (1) The average velocity can be written as v x = 1 2 ( v xi + v xf ) or v x = x f − x i . t Solving the latter expression for x f we get x f = x i + v x t. Substituting the first expression for v x into the above equation yields an expression for the final position in terms of the initial position, the initial velocity, the final velocity, and the time x f = x i + 1 2 ( v xi + v xf ) t (2) Substituting eqn. (1) into eqn. (2), we get x f = x i + 1 2 ( v xi + v xi + a x t ) t which yields an expression for the final position in terms of the initial position, the initial velocity, the acceleration, and the time x f = x i + v xi t + 1 2 a x t 2 . (3) 1

Solving eqn. (1) for t and substituting into (2), we get x f = x i + 1 � v xf − v xi � 2 ( v xi + v xf ) a which can be solved for the final velocity squared in terms of the initial velocity squared, the acceleration, and the displacement v 2 xf = v 2 xi + 2 a x ( x f − x i ) . (4) Graphs of position, velocity, and acceleration as a function of time for motion with con- stant acceleration are shown in Figure 1. 1.1 Constant Acceleration Example You brake your Porsche from 75 km/h to 45 km/h over a distance of 88 m. (a) What is the acceleration, assumed to be constant? v xi = 75 km/h v xf = 45 km/h x f − x i = 88 m a x =? v 2 xf = v 2 xi + 2 a x ( x f − x i ) 2 ( x x − x i ) = (45 km/h ) 2 − (75 km/h ) 2 a x = v 2 xf − v 2 xi = − 2 . 0 × 10 4 km/h 2 2 (0 . 088 km ) � � 1 h � 2 a x = − 2 . 0 × 10 4 km � 1000 m = − 1 . 5 m/s 2 h 2 1 km 3600 s (b) What is the elapsed time? x f − x i = 1 2 ( v xi + v xf ) t t = 2 ( x f − x i ) 2 (0 . 088 km ) 75 km/h + 45 km/h = 1 . 5 × 10 − 3 h = 5 . 3 s = v xi + v xf (c) If you continue to slow down with the acceleration calculated in (a), how much time will elapse in bringing the car to rest from 75 km/h? a x = − 2 . 0 × 10 4 km/h 2 v xi = 75 km/h v xf = 0 t =? v xf = v xi + a x t t = v xf − v xi 0 − 75 km/h − 2 . 0 × 10 4 km/h 2 = 3 . 8 × 10 − 3 h = 14 s = a x 2

Figure 1: Graphs of position, velocity, and acceleration versus time for motion with con- stant acceleration. 3

2 Freely Falling Objects Free fall near the surface of the earth is the most common example of motion with (nearly) constant acceleration. Free-fall acceleration near the surface of the earth is g = 9.80 m/s 2 . In solving free-fall problems, we will use the equations derived earlier for motion with constant acceleration with the following substitutions x → y and a x → a y = − g = 9 . 8 m/s 2 . 2.1 Free Fall Example A worker drops a wrench down an elevator shaft of a tall building. (a) Where is the wrench 1.5 seconds later? y i = 0 v yi = 0 t = 1 . 5 s y f =? y f = y i + v yi t + 1 2 a y t 2 y f = 0 + 0 + 1 (1 . 5 s ) 2 = − 11 m � − 9 . 8 m/s 2 � 2 (b) How fast is the wrench falling after 1.5 s? � − 9 . 8 m/s 2 � v yf = v yi + a y t = 0 + (1 . 5 s ) = − 15 m/s 3 Homework Set 2 - Due Monday, Sept. 13 • Read Sections 2.6-2.7 • Answer Question 9 & 11 • Do Problems 19, 26, 33 & 39 4