= a a m ; a m m = 1,2,3 Consider : Is it possible that all three - PowerPoint PPT Presentation

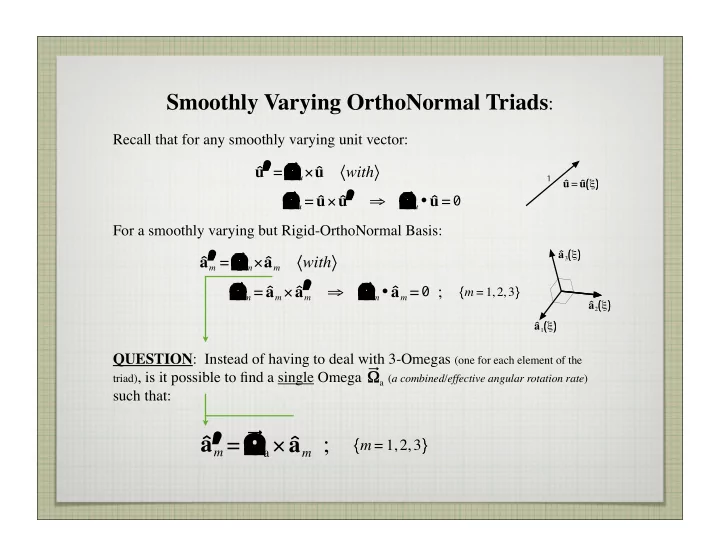
Smoothly Varying OrthoNormal Triads : Recall that for any smoothly varying unit vector: u ′ = Ω u × u with 1 u = u � Ω u = u × u ′ Ω u • u = 0 ⇒ For a smoothly varying but Rigid-OrthoNormal Basis: a 3 � ′ = Ω m × a m a m with ′ Ω m • a m = 0 ; Ω m = a m × a m ⇒ m = 1,2,3 a 2 � a 1 � QUESTION : Instead of having to deal with 3-Omegas (one for each element of the triad) , is it possible to find a single Omega ( a combined/effective angular rotation rate ) Ω a such that: ′ = Ω a × a m ; a m m = 1,2,3

Consider : Is it possible that all three Omegas are EQUAL , i.e. the same vector? Let us assume that they are, so that: Ω a = Ω 1 = Ω 2 = Ω 3 Unfortunately, this supposition leads to the conclusion: Ω a = Ω a • a 1 a 1 + Ω a • a 2 a 2 + Ω a • a 3 a 3 Ω a = Ω 1 • a 1 a 1 + Ω 2 • a 2 a 2 + Ω 3 • a 3 a 3 0 0 0 Ω a = zero a 1 + zero a 2 + zero a 3 = ZERO Hence, it follows that the three Omegas are ALL EQUAL, ONLY if they are ALL ZERO! Not a very interesting case. Therefore: No simple answer to our original question!

Taking a different tack, lets now assume (for the sake of argument) that such a vector exists and see where that leads. Using the parallel / perpendicular decomposition, a vector with the desired Ω a property would necessarily satisfy: Ω a = a m • Ω a a m + a m ×Ω a × a m ; m = 1,2,3 Ω a = Ω a • a m a m + a m × Ω a × a m ; m = 1,2,3 ′ ; Ω a = Ω a • a m a m + a m × a m m = 1,2,3 Ω a = Ω a • a m a m + Ω m ; m = 1,2,3 Ω a = Ω a • a 1 a 1 + Ω 1 ; m = 1 Ω a = Ω a • a 2 a 2 + Ω 2 ; m = 2 + Ω a = Ω a • a 3 a 3 + Ω 3 ; m = 3 3 ⋅ Ω a = Ω a • a 1 a 1 + Ω a • a 2 a 2 + Ω a • a 3 a 3 + Ω 1 + Ω 2 + Ω 3 3 ⋅ Ω a = Ω a + Ω 1 + Ω 2 + Ω 3 2 ⋅ Ω a = Ω 1 + Ω 2 + Ω 3 Ω a = 1 2 Ω 1 + Ω 2 + Ω 3 Conclude: This is the ONLY vector which COULD have the desired property!

Since Ω a = 1 2 Ω 1 + Ω 2 + Ω 3 is the ONLY vector which could possibly have the desired property, we now adopt it as a TRIAL solution, and then proceed to see if it works. But first, …some more useful info about our original 3-Omegas. 1 ; whenever m = n a m • a n = const. = 0 ; whenever m ≠ n d ′ = 0 d ξ a m • a n = a m ′ • a n + a m • a n 0 = a m • a n ′ + a n • a m ′ 0 = a m • Ω n × a n + a n • Ω m × a m 0 = Ω n • a n × a m + Ω m • a m × a n ; {TSP identity} 0 = − Ω n • a m × a n + Ω m • a m × a n 0 = Ω m − Ω n • a m × a n For the choice of “free-indices” with m = n , this identity is trivially satisfied. More interesting conclusions result from choices for which m ≠ n .

Ω m − Ω n • a m × a n = 0 m =2 & n =3 : Ω 2 − Ω 3 • a 2 × a 3 = 0 Ω 2 − Ω 3 • a 1 = 0 ε 1 = Ω 2 • a 1 = Ω 3 • a 1 ⇒ m =3 & n =1 : Ω 3 − Ω 1 • a 3 × a 1 = 0 Ω 3 − Ω 1 • a 2 = 0 ε 2 = Ω 3 • a 2 = Ω 1 • a 2 ⇒ m =1 & n =2 : Ω 1 − Ω 2 • a 1 × a 2 = 0 Ω 1 − Ω 2 • a 3 = 0 ε 3 = Ω 1 • a 3 = Ω 2 • a 3 ⇒ Thus, the 3-Omegas SHARE certain vector components. We will now combine this new observation with the previously derived perpendicularity conditions: Ω 1 • a 1 = Ω 2 • a 2 = Ω 3 • a 3 = 0

Ω m = Ω m • a 1 a 1 + Ω m • a 2 a 2 + Ω m • a 3 a 3 ; m = 1,2,3 Ω 1 = Ω 1 • a 1 a 1 + Ω 1 • a 2 a 2 + Ω 1 • a 3 a 3 Ω 2 = Ω 2 • a 1 a 1 + Ω 2 • a 2 a 2 + Ω 2 • a 3 a 3 Ω 3 = Ω 3 • a 1 a 1 + Ω 3 • a 2 a 2 + Ω 3 • a 3 a 3 Ω 1 = 0 a 1 + ε 2 a 2 + ε 3 a 3 = ε 2 a 2 + ε 3 a 3 Ω 2 = ε 1 a 1 + 0 a 2 + ε 3 a 3 = ε 1 a 1 + ε 3 a 3 + Ω 3 = ε 1 a 1 + ε 2 a 2 + 0 a 3 = ε 1 a 1 + ε 2 a 2 Ω 1 + Ω 2 + Ω 3 = 2 ε 1 a 1 + 2 ε 2 a 2 + 2 ε 3 a 3 Ω 1 + ε 1 a 1 Ω a = 1 2 Ω 1 + Ω 2 + Ω 3 = ε 1 a 1 + ε 2 a 2 + ε 3 a 3 = Ω 2 + ε 2 a 2 Ω 3 + ε 3 a 3 Ω a = Ω m + ε m a m ; m = 1,2,3

From here, it is easily shown that our “candidate” vector does indeed have the desired property, namely that: Ω a × a m = Ω m + ε m a m × a m = Ω m × a m + ε m a m × a m ′ + ε m 0 = a m Ω a × a m = a m ′ ′ = Ω a × a m ; a m m = 1,2,3 Therefore: Trial Vector has the desired property!

At any instant during the smooth evolution of a Rigid OrthoNormal triad, there exists a unique combined - effective Angular Rotation Rate vector such that: Ω a ′ = Ω a × a m ; a m m = 1,2,3 This vector can be determined from any of the following relations: 1 2 Ω 1 + Ω 2 + Ω 3 = 3 2 Ω AVG Ω 1 + ε 1 a 1 Ω a = Ω 2 + ε 2 a 2 Ω 3 + ε 3 a 3 ε 1 a 1 + ε 2 a 2 + ε 3 a 3 expressed in terms of the individual omegas: Ω 1 = a 1 × a 1 ′ Ω 2 = a 2 × a 2 ′ Ω m = a m × a m ′ ; m = 1, 2, 3 Ω 3 = a 3 × a 3 ′ ε 1 = Ω 2 • a 1 = Ω 3 • a 1 = a 2 ′ • a 3 = − a 3 ′ • a 2 ε 2 = Ω 3 • a 2 = Ω 1 • a 2 = a 3 ′ • a 1 = − a 1 ′ • a 3 which have the various scalar components: ε 3 = Ω 1 • a 3 = Ω 2 • a 3 = a 1 ′ • a 2 = − a 2 ′ • a 1

Why? What’s the Big deal? a 3 � A ( ξ ) = A 1 ( ξ ) a 1 ( ξ ) + A 2 ( ξ ) a 2 ( ξ ) + A 3 ( ξ ) a 3 ( ξ ) a 2 � a 1 � ; A m ( ξ ) = A ( ξ ) • a m ( ξ ) ; m = 1,2,3 d ξ A = d d d ξ A 1 a 1 + A 2 a 2 + A 3 a 3 = d d ξ A 1 a 1 + d d ξ A 2 a 2 + d d ξ A 3 a 3 ′ a 1 + A 1 a 1 ′ + A 2 ′ a 2 + A 2 a 2 ′ + A 3 ′ a 3 + A 3 a 3 = A 1 ′ ′ a 1 +A 2 ′ a 2 +A 3 ′ a 3 + A 1 a 1 ′ = Ω a × a m ; = A 1 ′ + A 2 a 2 ′ + A 3 a 3 ′ a m m = 1,2,3 ′ a 1 +A 2 ′ a 2 +A 3 ′ a 3 + A 1 Ω a × a 1 + A 2 Ω a × a 2 + A 3 Ω a × a 3 = A 1 ′ a 1 +A 2 ′ a 2 +A 3 ′ a 3 + Ω a × A 1 a 1 + Ω a × A 2 a 2 + Ω a × A 3 a 3 = A 1 ′ a 1 +A 2 ′ a 2 +A 3 ′ a 3 + Ω a × A 1 a 1 + A 2 a 2 + A 3 a 3 = A 1 A ′ = A 1 ′ a 1 +A 2 ′ a 2 +A 3 ′ a 3 + Ω a × A Scalar component Omega derivatives correction term