5 ksi
play

' = 5 ksi f c ( )( ) 15 . = 15 . ' = = E 33000 w f - PDF document

Feb 01, 2023 •459 likes •943 views

DESIGN EXAMPLE 1 This example illustrates the design of an interior and exterior beam of a precast prestressed concrete beam bridge using fully prestressed beams with harped bonded strands in accordance with the AASHTO LRFD Bridge Design

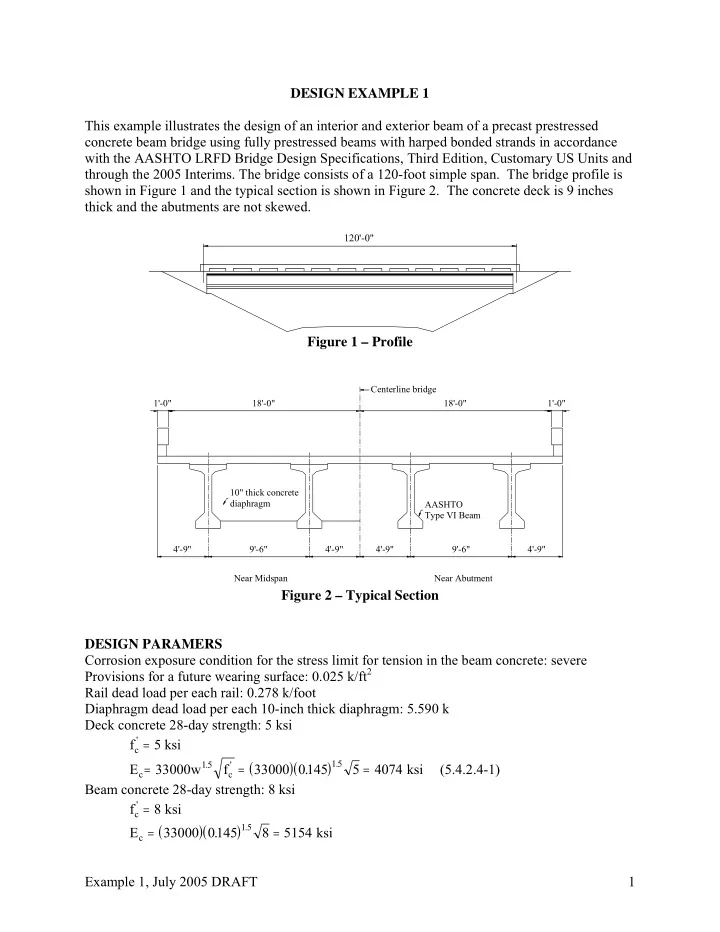
  1. DESIGN EXAMPLE 1 This example illustrates the design of an interior and exterior beam of a precast prestressed concrete beam bridge using fully prestressed beams with harped bonded strands in accordance with the AASHTO LRFD Bridge Design Specifications, Third Edition, Customary US Units and through the 2005 Interims. The bridge consists of a 120-foot simple span. The bridge profile is shown in Figure 1 and the typical section is shown in Figure 2. The concrete deck is 9 inches thick and the abutments are not skewed. 120'-0" Figure 1 – Profile Centerline bridge 1'-0" 18'-0" 18'-0" 1'-0" 10" thick concrete diaphragm AASHTO Type VI Beam 4'-9" 9'-6" 4'-9" 4'-9" 9'-6" 4'-9" Near Midspan Near Abutment Figure 2 – Typical Section DESIGN PARAMERS Corrosion exposure condition for the stress limit for tension in the beam concrete: severe Provisions for a future wearing surface: 0.025 k/ft 2 Rail dead load per each rail: 0.278 k/foot Diaphragm dead load per each 10-inch thick diaphragm: 5.590 k Deck concrete 28-day strength: 5 ksi ' = 5 ksi f c ( )( ) 15 . = 15 . ' = = E 33000 w f 33000 0145 . 5 4074 ksi (5.4.2.4-1) c c Beam concrete 28-day strength: 8 ksi ' = 8 ksi f c ( )( ) 15 . E c = = 33000 0145 . 8 5154 ksi Example 1, July 2005 DRAFT 1

  2. Beam concrete strength at release: 7 ksi ' = 7 ksi f ci ( )( ) 15 . E ci = = 33000 0145 . 7 4821 ksi Non-prestressed reinforcement: Grade 60 f y = 60 ksi s = 29 000 E , ksi (5.4.3.2) Prestressing steel: 0.6-inch diameter, 270-ksi low relaxation strand f pu = 270 ksi ( )( ) py = = = f 090 . f pu 0 90 270 . 243 k si (Table 5.4.4.1-1) E ps = 28 500 , ks i (5.4.4.2) SECTION PROPERTIES The non-composite and composite section properties are summarized in Table 1. Although the haunch between the top of the girder and the bottom of the deck slab is not included in the composite section properties, it is included in the dead loads. In order to calculate the composite section properties, first calculate the effective flange width. Effective Flange Width (4.6.2.6.1) Calculate the effective flange width for the interior beam first. For the interior beam, the effective flange width may be taken as the least of: a) One-quarter of the effective span length: 120 feet for simple spans (0.25)(120)(12) = 360 in b) Twelve time the average thickness of the slab, 9 inches, plus the greater of: The web thickness: 8 inches One-half of the top flange of the girder: 42 inches (0.5)(42) = 21 in The greater of these two values is 21 inches and: (12)(9) + 21 = 129 in c) The average spacing of adjacent beams: 9.5 feet (9.5)(12) = 114 in The least of these is 114 inches and therefore, the effective flange width is 114 inches. For the exterior beam, the effective flange width may be taken as one-half the effective flange width of the adjacent interior beam, 114 inches, plus the least of: a) One-eight of the effective span length: 120 feet (0.125)(120)(12) = 180 in b) Six times the average thickness of the slab, 9 inches, plus the greater of: One-half of the web thickness: 8 inches (0.5)(8) = 4 in One-quarter of the top flange of the girder: 42 inches (0.25)(42) = 10.5 in The greater of these two values is 10.5 inches and: (6)(9) + 10.5 = 64.5 in Example 1, July 2005 DRAFT 2

  3. c) The width of the overhang: 4.75 feet (4.75)(12) = 57 in The least of these is 57 inches and the effective flange width is: (0.5)(114) + 57 = 114 in Composite Section Properties area of non-composite beam or deck (in 2 ) A = d = distance between the centers of gravity of the beam or deck and the composite section (in) moment of inertia of non-composite beam or deck (in 4 ) I o = I comp = moment of inertia of composite section (in 4 ) section modulus of non-composite section, extreme bottom beam fiber (in 3 ) S b = section modulus of composite section, extreme bottom beam fiber (in 3 ) S bc = section modulus of composite section, extreme top deck slab fiber (in 3 ) S slab top = section modulus of non-composite section, extreme top beam fiber (in 3 ) S t = section modulus of composite section, extreme top beam fiber (in 3 ) S tc = y b = distance from the center of gravity of the non-composite section to the bottom of the beam (in) y bc = distance from the center of gravity of the composite section to the bottom of the beam (in) y slab top = distance from the center of gravity of the composite section to the top of the deck slab (in) y t = distance from the center of gravity of the non-composite section to the top of the beam (in) y tc = distance from the center of gravity of the composite section to the top of the beam (in) w = weight of the non-composite beam (k/ft) For the composite section, the modular ratio, n, to account for different concrete strengths in the beam and deck slab is: E 4074 deck = = = n 07906 . E 5154 beam The transformed deck area is: ( )( )( ) A = = 2 114 0 7906 9 . 81112 . in The moment of inertia of the transformed deck area is: ( )( )( ) 3 114 0 7906 9 . I o = = in 3 5475 12 Ad 2 I o + Ad 2 A y b Ay b d I o Deck 811.12 76.50 62051.00 22.96 427499 5475 432974 Beam 1085.00 36.38 39472.30 17.16 319590 733320 1052910 Total 1896.12 101523.30 1485884 ∑ Ay 10152330 . I 1485884 b = = = = c = = in 3 y 5354 . in S 27751 ∑ bc bc 189612 . y 5354 . A bc Example 1, July 2005 DRAFT 3

  4. I 1485884 in 3 = − = − = = c = = y h y 72 5354 . 1846 . in S 80503 tc beam bc tc y 1846 . tc I 1485884 in 3 = + = + = = c = = y y t 1846 . 9 27 46 . in S 68443 ( )( ) slab top tc slab slab top y n 27 46 07906 . . slab top (114)(0.7906) = 90.12" C. G. Slab 9" 22.96" 18.46" C. G. Composite Section 17.16" 76.50" 72" C. G. Beam 53.54" 36.38" Figure 3 – Composite Section, Interior and Exterior Beams Table 1 – Section Properties Non-composite Section Composite Section Property Type VI Beams Property Interior Beam Exterior Beam A (in 2 ) I comp (in 4 ) 1085 1485884 1485884 I (in 4 ) 733320 y bc (in) 53.54 53.54 y b (in) 36.38 y tc (in) 18.46 18.46 y t (in) 35.62 y slab top (in) 27.46 27.46 S b (in 3 ) S bc (in 3 ) 20157 27751 27751 S t (in 3 ) S tc (in 3 ) 20587 80503 80503 S slab top (in 3 ) w (k/ft) 1.130 68443 68443 DEAD LOADS The rail and future wearing surface allowance loads are distributed equally to all beams. Interior Beam The dead loads, DC, acting on the non-composite section are: Beam: 1.130 k/foot Slab: (9.5)(0.75)(0.150) = 1.069 k/ft Haunch: 0.025 k/foot Diaphragms: 5.590 k at midspan Example 1, July 2005 DRAFT 4

  5. The dead load, DC, acting on the composite section is: Rail: ( )( ) 0278 2 . = 0139 . k / ft 4 The dead load, DW, acting on the composite section is: Future wearing surface allowance (FWS): ( )( ) 0025 36 . = 0225 . k / ft 4 Exterior Beam The dead loads, DC, acting on the non-composite section are: Beam: 1.130 k/foot Slab: (9.5)(0.75)(0.150) = 1.069 k/ft Haunch: 0.025 k/foot Diaphragms: 2.795 k at midspan The dead load, DC, acting on the composite section is: Rail: 0.139 k/foot The dead load, DW, acting on the composite section is: FWS: 0.225 k/foot DISTRIBUTION OF LIVE LOAD Use the approximate formulas found in Article 4.6.2.2 for cross section k, a concrete slab on concrete beams. K g = longitudinal stiffness parameter (in 4 ) L = span length (ft) N b = number of girders S = girder spacing (ft) t s = deck slab thickness (in) Interior Beam LONGITUDINAL STIFFNESS PARAMETER (4.6.2.2.1) e g = the distance between the centers of gravity of the basic beam and the deck (in) t 9 s = + = + = e y 3562 . 4012 . in g t 2 2 The modular ratio for calculating the longitudinal stiffness parameter is: E 5154 B = = = n 12651 . E 4074 D The longitudinal stiffness parameter is: ( ) [ ] ( ) ( )( ) 2 2 4 = + = + = K n I Ae 12651 733320 . 1085 4012 . 3137123 in g g Example 1, July 2005 DRAFT 5

Recommend

More recommend