2 K s 10 s 100 4 3 2 s 20 s 100 s 500 s - PowerPoint PPT Presentation

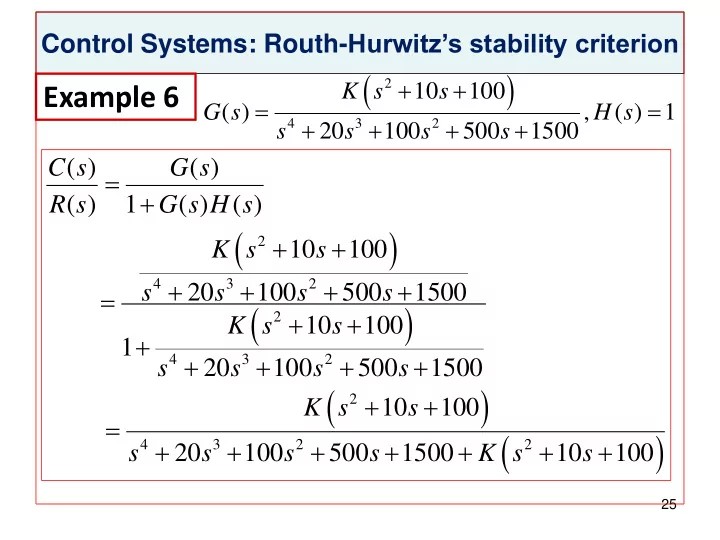
Control Systems: Routh- Hurwitz’s stability criterion     2 K s 10 s 100 Example 6   G s ( ) , H s ( ) 1     4 3 2 20 100 500 1500 s s s s C s ( ) G s ( )   R s ( ) 1 G s H s ( ) ( )     2 K s 10 s 100     4 3 2 s 20 s 100 s 500 s 1500      2 K s 10 s 100  1     4 3 2 s 20 s 100 s 500 s 1500     2 K s 10 s 100           4 3 2 2 20 100 500 1500 10 100 s s s s K s s 25

Control Systems: Routh- Hurwitz’s stability criterion Example 6 Method I using determinants         4 3 2 B s ( ) s 20 s s (100 K ) s (500 10 ) 1500 100 K K  a a 0 20 500 10 K 0 3 1      a a a 1 100 K 1500 100 K 3 4 2 0  0 a a 0 20 500 10 K 3 1 The value of ∆ 1 =20 >0 and the value of ∆ 2 =1500+10K >0 ,     100( K 7.8)( K 192.2) 7.8 < K < 192.2 conditions for 3 stability 26

Control Systems: Routh- Hurwitz’s stability criterion Example 6 Method II using array         4 3 2 B s ( ) s 20 s s (100 K ) s (500 10 ) 1500 100 K K n s a a a 4 2 0  1 n 0 s a a 3 1  a a a a a a     n 2 3 2 4 1 3 o s b b 0 3 0 a a 3 3 b a b a   n 3 3 1 0 3 s b 3 27

Control Systems: Routh- Hurwitz’s stability criterion Example 6 Method II using array   n s 1 100 K 1500 100 K   n 1 s 20 500 10 K 0  1500 10 K   n 2 s 1500 100 K 0 20   100( K 7.8)( K 192.2)  n 3 s  1500 10 K conditions for 7.8 < K < 192.2 stability 28

Control Systems: Routh- Hurwitz’s stability criterion Example 7 K   ( ) , ( ) 1 G s H s    4 3 2 s s s s C s ( ) G s ( )   ( ) 1 ( ) ( ) R s G s H s K    4 3 2 s s s s       4 3 2 B s ( ) s s s s K K  1    4 3 2 s s s s K      4 3 2 s s s s K 29

Control Systems: Routh- Hurwitz’s stability criterion Example 7 Method I using determinants 1 1 0   1 1 K 3 ∆ 1 =1 >0 and the value of ∆ 2 =0. ∆ 3 =-K 0 1 1 The system is unstable for all values of K 30

Control Systems: Routh- Hurwitz’s stability criterion Example 7 Method II using array      4 3 2 B s ( ) s s s s K n s a a a 4 2 0  n 1 s a a 0 3 1  a a a a a a    n 2 3 2 4 1 3 o s b b 0 3 0 a a 3 3 b a b a   n 3 3 1 0 3 s b 3 31

Control Systems: Routh- Hurwitz’s stability criterion Example 7 Method II using array 4 s 1 1 K 3 s 1 1 0  2 The system is unstable for all values 0 s K of K K  1 s 1 0 0  0 s K 0 0 32

ME 779 Control Systems Topic #14 Controller Basics Reference textbook : Control Systems, Dhanesh N. Manik, Cengage Publishing, 2012 1

Control Systems: Controller Basics Learning Objectives • Proportional controllers • Proportional and derivative controllers • Proportional and integral controllers • Proportional, integral and derivative controllers 2

Control Systems: Controller Basics Closed-loop (feedback) systems Block diagram 3

Control Systems: Controller Basics Proportional controller for inertia load Proportional controller Proportional control system for inertia load 4

Control Systems: Controller Basics Proportional controller for inertia load K p K K Closed-loop response C s ( ) 2 Js    p p    2 2 2 K R s ( ) Js K J s ( )  p p n 1 2 Js K   p n J    c t ( ) 1 cos t Step response n 5

Control Systems: Controller Basics Proportional controller for inertia load Step response 6

Control Systems: Controller Basics Proportional plus derivative controller for inertia load Proportional plus derivative controller K  d T d K p Proportional and derivative control for inertial load 7

Control Systems: Controller Basics Proportional plus derivative controller for inertia load  K (1 T s )   p d ( ) (1 ) G s K T s  K (1 T s ) ( ) C s 2 c p d Js   p d    (1 ) 2 K T s R s ( ) Js K (1 T s )  p d p d 1 2 K K Js T   p   d p  K (1 T s ) 2 J n  p d J   K T K   p d p 2   J s s   J J        K          p  t  ( ) 1 cos sin c t e t t n  d d 2     J   2  1    n Step response       K T 1       p d t 2   e sin 1 t n  n   J  2    1 n 8

Control Systems: Controller Basics Proportional plus derivative controller for inertia load  K (1 T s ) p d  C s ( ) 2 Js cs   K (1 T s ) ( ) R s  p d 1  2 Js cs  K (1 T s )  p d    2 Js s c ( K T ) K      p d p c K T 1   p d   2   JK    p K (1 T s ) C s ( )  p d     2 2 R s ( ) J s ( 2 s ) n n 9

Control Systems: Controller Basics Proportional plus derivative controller for inertia load        K          p t   c t ( ) 1 e cos t sin t n  d d 2     Step response J  2   1    n       K T 1       p d t 2   e sin 1 t n  n   J  2   1  n Derivative control adds K    p c ( ) 1 damping to the system J  2 n 10

Control Systems: Controller Basics Comparison of proportional and integral controllers Integral Proportional K K  i  G p G  2  1 s s ( 1) s 1  E s ( ) 1 s 1  E s ( ) 1 1 s s ( 1)      1 2      K 2 R s ( ) 1 G s ( ) s 2 R s ( ) 1 G s ( ) s s 1  i 1 2 1  s s ( 1) 11

Control Systems: Controller Basics Comparison of proportional and integral controllers Integral Proportional   E s ( ) 1 1 s s ( 1) E s ( ) 1 s 1      2 1    K 2 R s ( ) 1 G s ( ) s s 1    i R s ( ) 1 G s ( ) s 2 1 2  1 s s ( 1)   K ( s 1) U s ( ) K s ( 1) U ( ) s   p 2 1 i    2 R s ( ) s s 1 R s ( ) ( s 2) C s ( ) 1 1 C s 1 ( ) 1 1     2        2 2 R s ( ) s s K s s 1 R s ( ) s 1 K s 2 i p 12

Control Systems: Controller Basics Comparison of proportional and integral controllers Step response Integral Proportional     3 1 3 e     1 0.5 t   2 t e t ( ) e cos t sin t     e t ( ) 1 2 2 2   3 1 2     e  1   2 t   1 3 3 u t ( ) 1     0.5 t   u t ( ) 1 e sin t cos t 1 2 2   2 2   3   e    1   2 t 1 3 3       c t ( ) 1 0.5 t   c t ( ) 1 e sin t cos t 1 2   2 2 2   3 13

Control Systems: Controller Basics Comparison of proportional and integral controllers Steady-state error for step input 14

Control Systems: Controller Basics Comparison of proportional and integral controllers Plant input for step input 15

Control Systems: Controller Basics Comparison of proportional and integral controllers Closed-loop response for step input 16

Control Systems: Controller Basics PI (proportional-integral) controllers 17

Control Systems: Controller Basics PI (proportional-integral) controllers     sK K K 1    p i i     G K   p     1 ( 1) s s s s  E s ( ) 1 s s ( 1)       2 R s ( ) 1 G s ( ) s s (1 K ) K 1 p i   ( s 1)( sK K ) U ( ) s   p i 1    2 R s ( ) s s (1 K ) K p i 18

Control Systems: Controller Basics PI (proportional-integral) controllers  ( sK K ) C s ( ) 1   p i     2 ( ) (1 ) ( 1) R s s s K K s p i u t  e   t ( ) 1 e t ( ) Step response   e  t   c t ( ) 1 19

Control Systems: Controller Basics PI (proportional-integral) controllers Steady-state error for step input 20

Control Systems: Controller Basics PI (proportional-integral) controllers Plant input for step input 21

Control Systems: Controller Basics PI (proportional-integral) controllers Closed-loop response for step input Integral control reduces steady- state error 22