1
play

1 Vertical shifts Types of Transformations Moves the graph up or - PDF document

Sep 07, 2022 •428 likes •491 views

Transformation of Functions Recognize graphs of common functions Use vertical shifts to graph functions Symmetry & Use horizontal shifts to graph functions Use horizontal shifts to graph functions Transformations Use

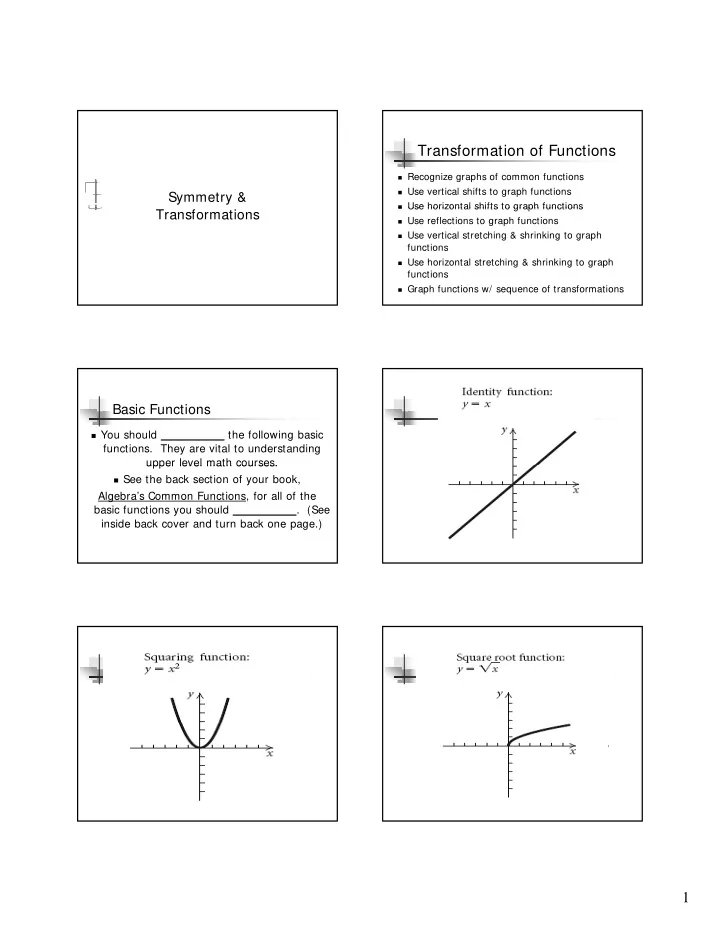
  1. Transformation of Functions � Recognize graphs of common functions � Use vertical shifts to graph functions Symmetry & � Use horizontal shifts to graph functions � Use horizontal shifts to graph functions Transformations � Use reflections to graph functions � Use vertical stretching & shrinking to graph functions � Use horizontal stretching & shrinking to graph functions � Graph functions w/ sequence of transformations Basic Functions � You should __________ the following basic functions. They are vital to understanding upper level math courses. upper level math courses. � See the back section of your book, Algebra’s Common Functions, for all of the basic functions you should __________. (See inside back cover and turn back one page.) 1

  2. � Vertical shifts Types of Transformations � Moves the graph up or down � Impacts only the “y” � Vertical translation : shifts basic graph up or values of the function down; ____ if y = f(x) + b or _____ if y = f(x) – b � No changes are made � Horizontal translation : shifts basic graph left or to the “x” values right; ______ if y f(x d) or ______ if y right; if y = f(x - d) or if y = f(x + f(x + � Horizontal shifts d) � Moves the graph left � Reflection : crosses an axis; or right across x axis if y = -f(x) & across y axis if y = f(-x) � Impacts only the “x” � Vertical stretching and shrinking : y = af(x) values of the function _______ if |a| > 1; _______ if 0< |a|< 1 � No changes are made to the “y” values � Horizontal stretching and shrinking : y = f(cx) stretch if 0< |c|< 1; shrink if |c|> 1 2

  3. Recognizing the shift from the equation, look at examples of Combining a vertical & horizontal shifting the function f(x)= x 2 . shift � Example of function � Vertical shift of 3 units up that is shifted down = = + 2 2 4 units and right 6 f f ( ( x ) ) x , , h ( ( x ) ) x 3 units from the f h original function. � Horizontal shift of 3 units left (HINT: x’s go the __________ direction that you might think.) = = − − ( ) , ( ) 6 4 f x x g x x = = + 2 2 ( ) , ( ) ( 3 ) f x x g x x Tests of Symmetry Types of Symmetry Symmetry with respect to the � f(x) = f(-x) symmetric to _________ � y-axis (x, y) & (____ , y) are even function reflections across the y-axis reflections across the y axis � - f(x) = f(-x) � Origin (x, y) & _________ are symmetric to _________ _________ function reflections across the origin � x-axis (x, y) & (x, ____) are � f(x) = - f(x) symmetric to _________ reflections across the x-axis neither even nor odd Example – Determine any/all Example – Determine any/all = + symmetry of 6x + 7y = 0 . 2 symmetry of f x ( ) x 1 a) y-axis d) y-axis b) Origin b) Origin e) origin e) origin c) x-axis f) x-axis � Is the function even, odd, or neither? � Is the function even, odd, or neither? 3

  4. Horizontal stretch & shrink VERTICAL STRETCH (SHRINK) f(x) = |x 2 – 4| f(x) = |x 2 – 4| � We’re MULTIPLYING by � y’s do what we think an integer (not 1 or 0). they should: If you � x’s do the opposite of see 3(f(x)), all y’s what we think they what we think they are MULTIPLIED by b should. (If you see 3x in the equation where it 3 (it’s now 3 times used to be an x, you as high or low!) DIVIDE all x’s by 3, thus 2 − = it’s compressed or shrunk ( ) 3 4 h x x horizontally.) 2 − = ( ) ( 3 ) 4 g x x Sequence of transformations Graph of Example � Follow the _________ of operations. � Select two points (or more) from the original function and = x 3 ( ) f x _________ that point one step at a time. = 3 3 − f ( x ) x = + − = + ( ) 3 ( 2 ) 1 3 ( 2 ) 1 g x f x x + − = + − 3 3 ( 2 ) 1 3 ( 2 ) 1 f x x Your turn. Describe these transformations Transformations with with the Absolute Value Function. = 2 ( ) f x x = ( ) | | f x x the Squaring Function Function Transformation Function Transformation � g(x) = x 2 + 4 ________________________ � g(x) = -|x| ________________________ � h(x) = x 2 – 5 _______________________ � h(x) = |2x| ________________________ � j(x) = (x – 3) 2 ________________________ � j(x) = 3|x| ________________________ � k(x) = (x + 1) 2 � k(x) = |x + 4| ________________________ ________________________ � q(x) = |x - 5| ________________________ � q(x) = -x 2 ________________________ � r(x) = |x| - 1 ________________________ � r(x) = 2x 2 ________________________ � s(x) = -½ |x| ________________________ � s(x) = ¼ x 2 ________________________ � t(x) = |x| + 2 ________________________ � t(x) = (5x) 2 ________________________ 4

  5. Summary of Transformations � See instructor webpage. 5

Recommend

More recommend