x = y * y* Q x r x j ij i = + j i j * x* - PowerPoint PPT Presentation

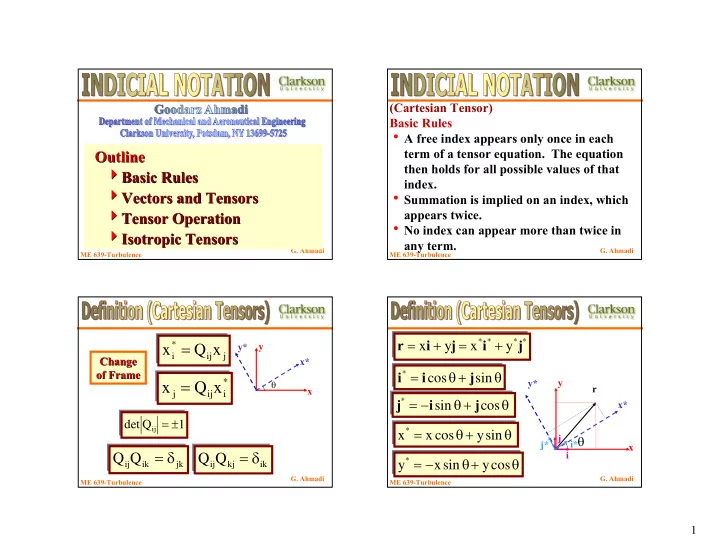
(Cartesian Tensor) Basic Rules h A free index appears only once in each term of a tensor equation. The equation Outline Outline then holds for all possible values of that 4 Basic Rules 4 Basic Rules index. 4 Vectors and Tensors 4 Vectors and Tensors h Summation is implied on an index, which appears twice. 4 Tensor Operation 4 Tensor Operation h No index can appear more than twice in 4 Isotropic Tensors 4 Isotropic Tensors any term. G. Ahmadi G. Ahmadi ME 639-Turbulence ME 639-Turbulence = + = + x = r i j * i * * j * * x y x y y* y Q x i ij j Change Change x* = θ + θ of Frame of Frame i * i j cos sin x = y * y* θ Q x r x j ij i = − θ + θ j i j * x* sin cos = ± det Q 1 = θ + θ ij x * x cos y sin j θ i* j* x = δ = δ i Q ij Q Q ij Q = − θ + θ y * x sin y cos ik jk kj ik G. Ahmadi G. Ahmadi ME 639-Turbulence ME 639-Turbulence 1

T * = Transformation in Scalar Transformation in θ θ Scalar ⎡ ⎤ T cos sin [ ] = Two Dimension Two Dimension Q ⎢ ⎥ − θ θ ⎣ ⎦ sin cos = ⋅ v Q v * Vector Vector Vector ⎡ ⎤ [ ] 1 0 δ = Kronecker Kronecker ⎢ ⎥ Second Order Second Order = ⋅ ⋅ Delta Delta ij τ Q t Q * T ⎣ ⎦ 0 1 Tensor Tensor G. Ahmadi G. Ahmadi ME 639-Turbulence ME 639-Turbulence v = * ε Alternating Alternating Vector Q v Vector i ij j ijk Symbol Symbol Second Second t = * Q Q t ε = Order Tensor Order Tensor 1 , for i , j , k even permutatio n ij ik jl kl ijk ε = − 1 , for i , j , k odd permutatio n ijk Third Order Third Order λ = λ * ε = Q Q Q 0 , when two indices are equal Tensor Tensor ijk ijk im jn kl mnl G. Ahmadi G. Ahmadi ME 639-Turbulence ME 639-Turbulence 2

∂ ϕ ∂ U ∇ ϕ = = ϕ ∇ × = ε = ε U ( ) Curl k Curl ( ) U ∂ i , i ∂ i ijk ijk k , j x x Gradient Gradient i j ∂ v ∇ = = j v ( ) v = ε ∂ A ij j , i Determinant Determinant det A A A x ijk 1 i 2 j 3 k i Divergence Divergence ∇ v ⋅ = ε ε = δ δ − δ δ v Identity Identity i , i ijk imn jm kn jn km ∂ τ ∂ ϕ 2 ∇ ϕ = = ϕ ∇ τ ⋅ = = τ 2 ij Laplacian Laplacian ( ) ∂ ∂ , ii ∂ j ij , i x x x i i i G. Ahmadi G. Ahmadi ME 639-Turbulence ME 639-Turbulence All Scalars Rank Zero: Rank Zero: All Scalars All Scalars Rank Four: Rank Four: None Rank One: Rank One: None None αδ δ + β δ δ + δ δ αδ ( ) ij kl ik jl il jk Rank Two: Rank Two: ij + γ δ δ − δ δ ( ) ik jl il jk αε Rank Three: Rank Three: ijk G. Ahmadi G. Ahmadi ME 639-Turbulence ME 639-Turbulence 3