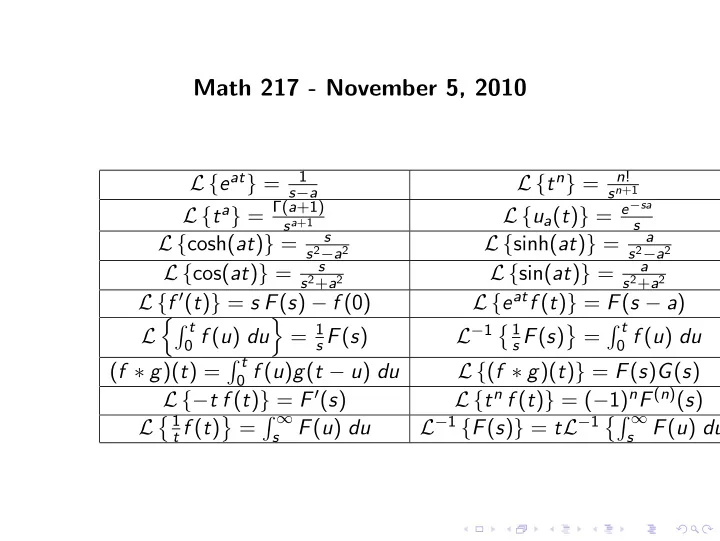
Math 217 - November 5, 2010 1 n ! L { e at } = L { t n } = s n +1 s - - PowerPoint PPT Presentation

Math 217 - November 5, 2010

L {eat} =

1 s−a

L {tn} =

n! sn+1

L {ta} = Γ(a+1)

sa+1

L {ua(t)} = e−sa

s

L {cosh(at)} =

s s2−a2

L {sinh(at)} =

a s2−a2

L {cos(at)} =

s s2+a2

L {sin(at)} =

a s2+a2

L {f ′(t)} = s F(s) − f (0) L {eatf (t)} = F(s − a) L t

0 f (u) du

• = 1

s F(s)

L−1 1

s F(s)

• =

t

0 f (u) du

(f ∗ g)(t) = t

0 f (u)g(t − u) du

L {(f ∗ g)(t)} = F(s)G(s) L {−t f (t)} = F ′(s) L {tn f (t)} = (−1)nF (n)(s) L 1

t f (t)

• =

∞

s

F(u) du L−1 {F(s)} = tL−1 ∞

s

F(u) du

• 1. Set up the partial fractions for the following.

(If you run out of things to be doing, work on the solutions).

(a) 1 s3(s − 3) = (b) 3s2 + 5 (s2 + 4s + 5)(s − 2) =

• 2. Find the inverse Laplace transforms for the previous problems. (At

least get the ones you know how to do!)

• 1. Set up the partial fractions for the following.

(If you run out of things to be doing, work on the solutions).

(a) 1 s3(s − 3) = − 1 27 s − 1 9 s2 − 1 3 s3 + 1 27 (s − 3) (b) 3s2 + 5 (s2 + 4s + 5)(s − 2) = 2 s s2 + 4 s + 5 + 1 s − 2

• 2. Find the inverse Laplace transforms for the previous problems. (At

least get the ones you know how to do!)

Lecture Problems

• 3. Find the inverse transforms using: L

t

0 f (u) du

• = 1

s F(s).

(a) L−1

• 1

s − 3

• =

(b) L−1

• 1

s(s − 3)

• =

(c) L−1

• 1

s2(s − 3)

• =

(d) L−1

• 1

s3(s − 3)

• =

Lecture Problems

• 3. Find the inverse transforms using: L

t

0 f (u) du

• = 1

s F(s).

(a) L−1

• 1

s − 3

• = e3t

(b) L−1

• 1

s(s − 3)

• =

t e3u du = 1 3(e3t − 1) (c) L−1

• 1

s2(s − 3)

• =

t 1 3(e3u − 1) du = e3 t 9 − t 3 − 1 9 (d) L−1

• 1

s3(s − 3)

• = e3 t

27 − t2 6 − t 9 − 1 27

• 4. Find the inverse transforms using: L {eatf (t)} = F(s − a).

(a) L−1

• 1

(s − 3)2 + 9

• =

(b) L−1

• s

(s − 3)2 + 9

• =

(c) L−1

• s + 1

s2 + 4s + 5

• =

(d) L−1

• 2 − 3s

4s2 − 24s + 37

• =

• 4. Find the inverse transforms using: L {eatf (t)} = F(s − a).

(a) L−1

• 1

(s − 3)2 + 9

• = 1

3e3t sin 3t (b) L−1

• s

(s − 3)2 + 9

• = e3 t (sin (3 t) + cos (3 t))

(c) L−1

• s + 1

s2 + 4s + 5

• = e−2 t (cos (t) − sin (t))

(d) L−1

• 2 − 3s

4s2 − 24s + 37

• = e3 t

−14 sin t

2

• − 3 cos

t

2

• 4

• 5. Homework problems (not to turn in, but you definitely should do

these!). Solve the differential equations using Laplace

(a) y ′′ + 2y ′ + 5y = 0, y(0) = 1, y ′(0) = 2. (b) y ′′ + 8y ′ + 25y = 0, y(0) = −1, y ′(0) = 1.

• 5. Homework problems (not to turn in, but you definitely should do

these!). Solve the differential equations using Laplace

(a) y ′′ + 2y ′ + 5y = 0, y(0) = 1, y ′(0) = 2. Solution: y = e−t

3 sin(2 t) 2

+ cos (2 t)

• (b) y ′′ + 8y ′ + 25y = 0, y(0) = −1, y ′(0) = 1.

Solution: y = e−4 t (− sin (3 t) − cos (3 t))