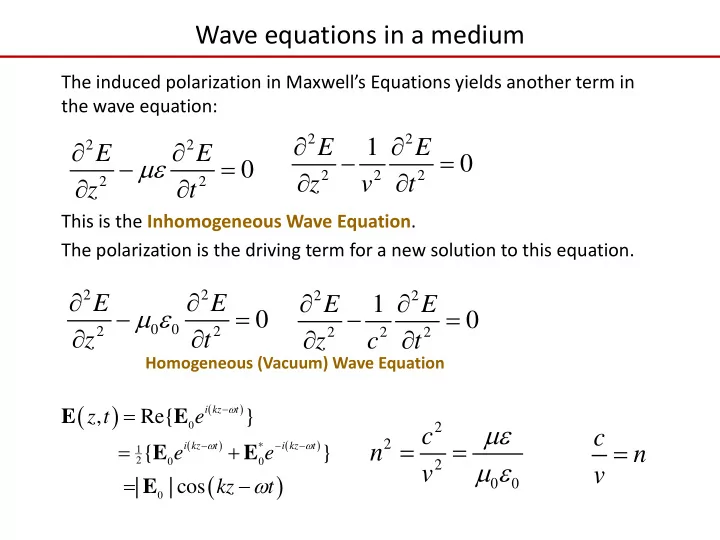
Wave equations in a medium The induced polarization in Maxwells - PowerPoint PPT Presentation

Wave equations in a medium The induced polarization in Maxwell’s Equations yields another term in the wave equation: ∂ ∂ 2 2 ∂ ∂ 1 E E 2 2 E E − = − µε = 0 0 ∂ ∂ 2 2 2 ∂ ∂ z v t 2 2 z t This is the Inhomogeneous Wave Equation . The polarization is the driving term for a new solution to this equation. ∂ ∂ ∂ ∂ 2 2 2 2 1 E E E E − µ ε = − = 0 0 ∂ ∂ 0 0 ∂ ∂ 2 2 2 2 2 z t z c t Homogeneous (Vacuum) Wave Equation ( ) ( ) = − ω i kz t , Re{ } E z t E e µε 0 2 c c = = v = ( ) ( ) = + 2 − ω − − ω i kz t * i kz t n { } n 1 E e E e µ ε 2 0 0 2 v ( ) = − ω | | cos 0 0 E kz t 0

Wave equations

Poynting vector & Intensity of Light S = E×H • Poynting vector describes flows of E-M power • Power flow is directed along this vector • Usually parallel to k • Intensity is equal to the magnitude of the time averaged Poyning vector: I=< S > ε ε 1239.85 ( ) c c ( ) ( ) ω =  = ≡ × = = + [ ] eV 2 2 2 | | 0 0 S I E t H t E E E λ [ ] x y nm 2 2 ε ≈ × − 3 2.654 10 / c A V = ×  − 34 1.05457266 10 Js 0 = example 1 / E V m = 2 ? / I W m

Reflection and Transmission @ dielectric interface

Beyond Snell’s Law: Polarization?

Reflection and Transmission (Fresnel’s equations) Can be deduced from the application of boundary conditions of EM waves.

Reflection and Transmission of Energy @ dielectric interfaces

Energy Conservation

Normal Incidence

Reflectance and Transmittance @ dielectric interfaces