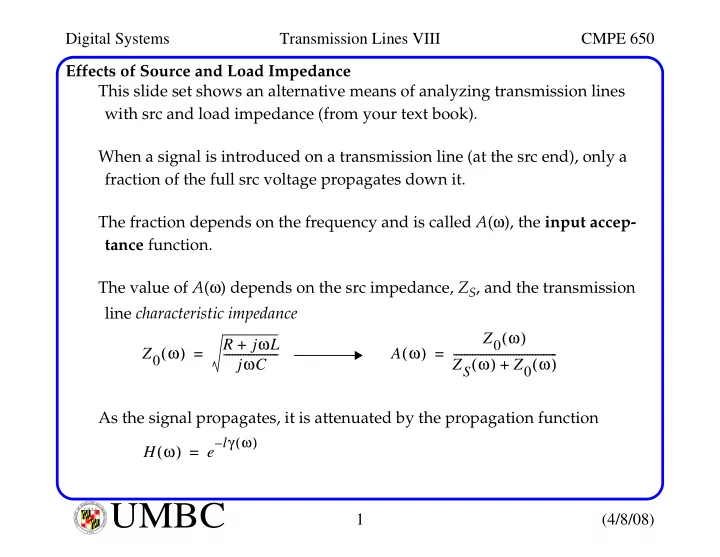

Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance This slide set shows an alternative means of analyzing transmission lines with src and load impedance (from your text book). When a signal is introduced on a transmission line (at the src end), only a fraction of the full src voltage propagates down it. The fraction depends on the frequency and is called A ( ω ), the input accep- tance function. The value of A ( ω ) depends on the src impedance, Z S , and the transmission line characteristic impedance Z 0 ω ( ) j ω L R + Z 0 ω ( ) A ω ( ) = - - - - - - - - - - - - - - - - - - - - = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - j ω C Z S ω ( ) Z 0 ω ( ) + As the signal propagates, it is attenuated by the propagation function e l γ ω ( ) – H ω ( ) = L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 1 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance At the far end of the cable a fraction of the attenuated signal amplitude emerges . This fraction is a function of frequency and is called T( ω ) , the output trans- mission function 2 Z L ω ( ) T ω ( ) (ranges from 0 to 2) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - Z L ω ( ) Z 0 ω ( ) + A reflected portion of the signal also travels back toward the src. As it reflects, the signal crosses over the tail of the incoming signal but does not interfere with it. The fraction of the signal reflected is called R 2 ( ω ) , the far end reflection func- tion Z L ω ( ) Z 0 ω ( ) – R 2 ω ( ) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - Z L ω ( ) Z 0 ω ( ) + L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 2 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance The refl ected signal is again attenuated by H ( ω ) and refl ects of f of the src impedance. The src end reflection coefficient Z S ω ( ) Z 0 ω ( ) – R 1 ω ( ) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - Z S ω ( ) Z 0 ω ( ) + This refl ection and attenuation continues, and each emerging signal at the load , S i , is described as shown in the following figure, i.e., S 0 ω ( ) A ω ( ) H ω ( ) T ω ( ) = Eventually, all signals N = [0, 1, ..., inf ] emerge and their sum is given by: ∞ ∑ S ∞ ω ( ) S N ω ( ) = N = 0 A ω ( ) H ω ( ) T ω ( ) S ∞ ω ( ) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - (closed form solution) ) H 2 ω R 2 ω ( ( ) R 1 ω ( ) 1 – L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 3 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance Refl ections H( ω ) T( ω ) A( ω ) A( ω )H( ω )T( ω ) Z S Z L Z 0 + R 2 ( ω ) - H( ω ) Some signal bounces off Some signal bounces off far end near end R 1 ( ω ) A( ω )H( ω )[R 2 ( ω )H 2 ( ω )R 1 ( ω )]T( ω ) H( ω ) T( ω ) Transmission Signal attenuates on function controls R 2 ( ω ) how much signal each pass H( ω ) gets out each pass R 1 ( ω ) A( ω )H( ω )[R 2 ( ω )H 2 ( ω )R 1 ( ω )] 2 T( ω ) H( ω ) T( ω ) R 2 ( ω ) L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 4 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance In this example, the total wire resistance is only 1.2 Ω and therefore we can ignore it and use Z 0 ( ω ) = 50 Ω (simplifies situation to make refl ections r eal) Trace width = 0.006 in. 15 in. 9 Ω 1-oz. copper Series R DC = 0.081 Ω /in Z 0 75 Ω Risetime = 1 ns + 1-V step - F knee = 500 MHz 1.00 0.955 H Skin effect R = 0.4 Ω /in. 0.847 0.796 T Total series R = 6 Ω R 2 0.159 A( ω ) = 0.847 H 0.150 H( ω ) = 0.94 Wfm observed T( ω ) = 1.200 R 1 at far end -0.104 R 2 ( ω ) = 0.200 -0.116 H -0.097 R 1 ( ω ) = -0.695 T R 2 0.020 50 Ω stripline H Settles to ε r = 4.5 -0.018 0.893 eventually T p = 180 ps/in. R 1 0.013 0.014 2700 ps total delay H 0.012 T L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 5 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance How can we control refl ections on a transmission line? Combining the T( ω ) and R 2 ( ω ) equations: 2 Z L ω ( ) T ω ( ) R 2 ω ( ) T ω ( ) = + 1 = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - Z L ω ( ) Z 0 ω ( ) + A ω ( ) H ω ( ) R 2 ω ( ( ) ) + 1 Z L ω ( ) Z 0 ω ( ) – S ∞ ω ( ) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - R 2 ω ( ) ) H 2 ω = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - Z L ω ( ) Z 0 ω ( ) R 2 ω ( ( ) R 1 ω ( ) + 1 – Assuming that H( ω ) is fixed, we can control two parameters, src and load impedance. The src impedance controls A( ω ) and R 1 ( ω ) while the load impedance controls R 2 ( ω ). For a good digital transmission, we want a fl at fr equency response up to at least the knee frequency. L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 6 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance There are three accepted methods for ensuring a fl atfrequency response from A ω ( ) H x ω ( ) R 2 ω ( ( ) ) + 1 S ∞ ω ( ) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - 2 ω R 2 ω ( ) H x ( ) R 1 ω ( ) 1 – These include end termination , series termination and short line . End Termination: This method sets R 2 ( ω ) to zero. H ω ( ) A ω ( ) S end term = This eliminates the first refl ection (all ener gy exits the cable) and any delayed versions of the original signal. To achieve R 2 ( ω ) = 0, just set Z L = Z 0 . As we indicated, for long cables operating in RC mode, finding a termi- nating network that matches the Z 0 over a wide frequency range is a challenge. L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 7 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance Source Termination : This method sets R 1 ( ω ) to zero. H ω ( ) A ω ( ) R 2 ω [ ( ) ] S src term = + 1 Physically, this eliminates the second refl ection but not the fi rst. To achieve R 1 ( ω ) = 0, just set Z S = Z 0 . Note that with Z S = Z 0 , the acceptance function becomes 1/2. This is usually compensated at the far end by leaving the line untermi- nated , Z L = inf . This, in turn, sets T( ω ) = 2 (and R 2 ( ω ) = 1), i.e., the line voltage is dou- bled at its end, compensating for the halving. c because R 2 ( ω ) = The drawback is the large signal that refl ects back to the sr 1. Circuits connected between the src and end see a mixed signal, a half sized signal propagating down followed by the other half. L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 8 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Digital Systems Transmission Lines VIII CMPE 650 Effects of Source and Load Impedance Short Line : This method sets H( ω ) ~= unity (because the line is short). Under this condition, there is no significant attenuation or phase delay. A ω ( ) R 2 ω ( ( ) ) + 1 S ∞ ω ( ) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - R 2 ω ( ) R 1 ω ( ) 1 – Substituting our previous expressions for these terms and simplifying yields Z L ω ( ) S short line ω ( ) = - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - Z L ω ( ) Z S ω ( ) + Here, we see the line has no effect and we get a simple impedance divider. However, the line must act as a lumped circuit element, i.e. it must be shorter than 1/6 the electrical length of the rising edge. T rise L = line inductance, H/in. 1 < Max Length - - - - - - - - - - - - - - 6 LC C = capacitance, F/in. L A N R Y D UMBC A B M A L T F O U M B C I M Y O R T 9 (4/8/08) I E S R C E O V U I N N U T Y 1 6 9 6
Recommend
More recommend