The random graph revisited every graph on n vertices occurs with - PDF document

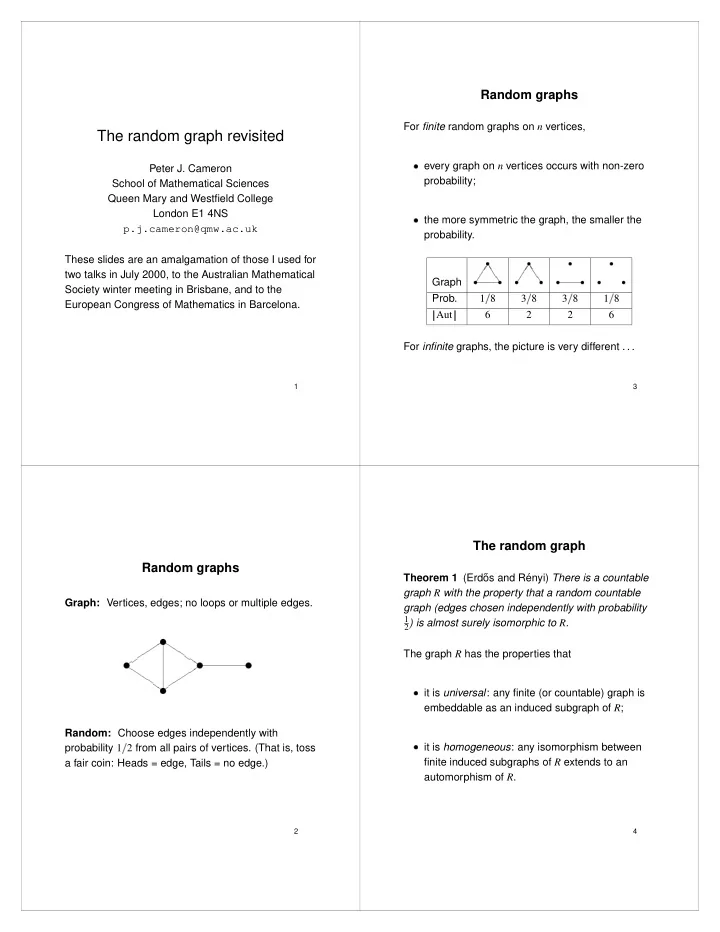
✆ ✞ ✂ ✂ ✂ ✂ ✂ ✂ ✂ ✂ ✂ ✂ ✂ ✂ ✝ ✝ ✝ ✝ ✆ ☎ ✆ ✆ ☎ ✞ ✞ ✞ ✝ ✆ ✆ ✂ ✂ ✁ ✁ ☎ ☎ ✡ ✆ ✆ ✆ ✆ ✆ ✆ ✞ ✞ � � � � ✁ ✁ ✁ ✁ ✁ ✁ ✁ ✁ � ✁ ✁ ✁ ✁ ✝ Random graphs For finite random graphs on n vertices, The random graph revisited every graph on n vertices occurs with non-zero Peter J. Cameron probability; School of Mathematical Sciences Queen Mary and Westfield College London E1 4NS the more symmetric the graph, the smaller the p.j.cameron@qmw.ac.uk probability. ✝✟✞ ✝✠✞ These slides are an amalgamation of those I used for two talks in July 2000, to the Australian Mathematical Graph ✄ 8 ✄ 8 ✄ 8 ✄ 8 Society winter meeting in Brisbane, and to the 1 3 3 1 Prob. ✡ Aut European Congress of Mathematics in Barcelona. 6 2 2 6 For infinite graphs, the picture is very different . . . 1 3 The random graph Random graphs Theorem 1 (Erd˝ os and R´ enyi) There is a countable graph R with the property that a random countable Graph: Vertices, edges; no loops or multiple edges. graph (edges chosen independently with probability 1 2 ) is almost surely isomorphic to R . The graph R has the properties that it is universal : any finite (or countable) graph is embeddable as an induced subgraph of R ; Random: Choose edges independently with ✄ 2 from all pairs of vertices. (That is, toss probability 1 it is homogeneous : any isomorphism between finite induced subgraphs of R extends to an a fair coin: Heads = edge, Tails = no edge.) automorphism of R . 2 4

✌ ☎ ☎ ✌ ☎ ✌ ✒ ☎ Universal homogeneous structures e) R is the unique countable Sketch proof Theorem 2 (Fra¨ ıss´ universal homogeneous graph. ☛ ) Given finite disjoint sets U ☞ V of Property ( ıss´ There are many other examples to which Fra¨ e’s vertices, there is a vertex joined to everything in U theorem or variants apply: and to nothing in V . the random tournament, digraph, hypergraph, Step 1 With probability 1 , a countable random graph etc.; has property ( ☛ ). the universal total order (Cantor), partial order, Uses the fact that a countable union of null sets is etc.; null. the universal triangle-free graph (Henson), Step 2 Any two countable graphs with property ( ☛ ) N-free graph (Covington), locally transitive are isomorphic. tournament (Lachlan), two-graph, etc.; A standard ‘back-and-forth’ argument. the universal locally finite group (Hall), Steiner triple system (Thomas), etc. 5 7 Constructions of R Measure and category Construction 1. Take any countable model of ZF , and join x to y if x y or y x . Measure theory and topology provide two concepts for saying that a set A takes up ‘almost all’ of the In fact we don’t need all of ZF , only the null set, pairing, union, and foundation axioms. So the sample space: it may be of full measure (the standard model of finite set theory (the set N , with complement of a null set) or residual (the y if the x th binary digit of y is 1 ) gives an explicit x complement of a meagre or first category set). construction (Rado). Sometimes these concepts agree (e.g. the random graph is ‘ubiquitous’ in both senses), sometimes they ✍ 1 be the set of primes Construction 2. Let congruent to 1 mod 4 . Join p to q if p is a quadratic don’t (e.g. Henson’s universal triangle-free graph is residual, but a random triangle-free graph is almost residue mod q . surely bipartite). ✍✏✎ 1 , the set of primes congruent to If we use instead ✑ 1 mod 4 , we obtain the random tournament. 6 8

✓ Homogeneous digraphs More generally . . . Cherlin has determined all the countable homogeneous directed graphs. There are There is a more general and powerful version due to uncountably many analogues of (c), but instead of Hrushovski. It constructs pseudoplanes, excluding one complete graph we have to exclude an distance-transitive graphs, and examples related to arbitrary antichain of tournaments. (The examples sparse random graphs (among other things). are due to Henson.) See the survey article by Wagner in Kaye and There are also a few sporadic ones. For example, Macpherson, Automorphisms of First-Order there are just three homogeneous tournaments: the Structures . linearly ordered set ✒ , the coutable ‘local order’, and the random tournament. 9 11 Homogeneous graphs Theorem 3 (Lachlan and Woodrow) The countably infinite homogeneous graphs are the following: First-order graph properties (a) the disjoint union of m complete graphs of size n , The graph R ‘controls’ first-order properties of finite where m and n are finite or countable (and at random graphs. least one is infinite); Theorem 4 ( Glebskii et al.) A first-order sentence in (b) the complements of the graphs under (a); the language of graphs holds in almost all finite graphs if and only if it holds in R . (c) the Fra¨ ıss´ e limit of the class of graphs containing no complete subgraph of size r , for given finite In particular, there is a zero-one law for first-order 3 ; r sentences. Of course, most interesting graph properties are not (d) the complements of the graphs under (c); first-order! (e) the random graph (the Fra¨ ıss´ e limit of the class of all finite graphs). 10 12

☎ ✔ ☎ ✔ ☎ ✒ ✥ ☎ Switching Indestructibility The operation of switching a graph Γ with respect to a set X of vertices, as defined by Seidel, works as R is unchanged by the following operations: follows: interchange edges and non-edges between X and its complement, leaving edges within and deleting finitely many vertices; outside X unaltered. ✕✗✖ Γ ✘ be the set of triples of vertices of Γ Let adding or removing finitely many edges; containing an odd number of edges. Theorem 7 Graphs Γ 1 and Γ 2 on the same vertex complementation (interchanging edges and set are related by switching if and only if non-edges); ✕✙✖ Γ 1 ✘✛✚✜✕✙✖ Γ 2 ✘ . In different language, this says that switching with respect to a finite set (see later). ✄ 2 H 2 ☞✣✢ ✢✤✘✏✚ 0 ✖ simplex In addition, the countable random graph with any given edge-probability p satisfying 0 p 1 is Switching has many applications in finite and isomorphic to R . Euclidean geometry, group theory, strongly regular graphs, etc. 13 15 Reducts Pigeonhole property A subgroup of the symmetric group is closed in the topology of pointwise convergence if and only if it is A structure X has the pigeonhole property if, the automorphism group of a first-order structure whenever X is partitioned into two parts, one of the (which can be taken to be a homogeneous relational parts is isomorphic to X . structure). Theorem 5 The countable graphs with the Theorem 8 (Thomas) There are five closed pigeonhole property are the complete graph, the null subgroups of Sym ✘ containing Aut ✘ , viz. Aut ✖ R ✖ R ✖ R ✘ , graph, and the random graph. the group of automorphisms and anti-automorphisms of R , the group of switching-automorphisms of R , the Theorem 6 (Bonato–Delic) The countable group of switching-automorphisms and tournaments with the pigeonhole property are the anti-automorphisms of R , and Sym ✖ R ✘ . random tournament, ordinal powers of ω , and their converses. Similar results are known in a few other cases, e.g. (as ordered set), random hypergraphs. 14 16