Surfaces in the space of surfaces Theorem (Shah, Ratner) The closure - PowerPoint PPT Presentation

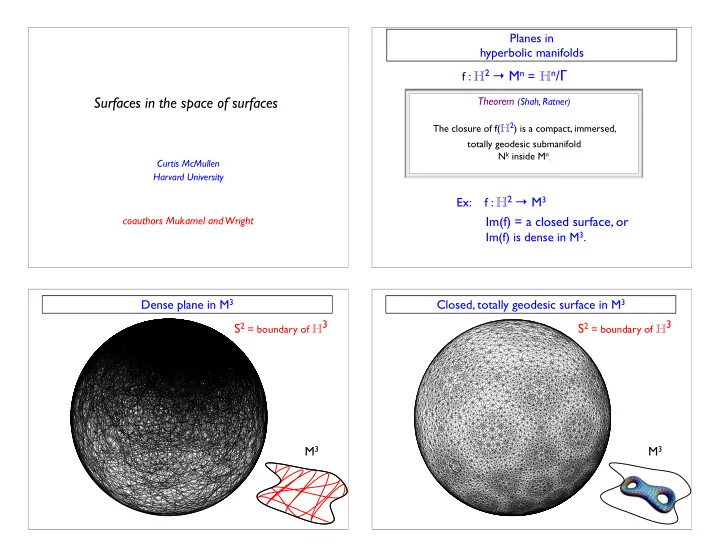
Planes in hyperbolic manifolds f : H 2 → M n = H n / Γ Surfaces in the space of surfaces Theorem (Shah, Ratner) The closure of f( H 2 ) is a compact, immersed, totally geodesic submanifold N k inside M n. Curtis McMullen Harvard University Ex: f : H 2 → M 3 coauthors Mukamel and Wright Im(f) = a closed surface, or Im(f) is dense in M 3 . Dense plane in M 3 Closed, totally geodesic surface in M 3 S 2 = boundary of H 3 S 2 = boundary of H 3 M 3 M 3

Arithmetic tetrahedra Moduli space M g = moduli space of Riemann surfaces X of genus g { } -- a complex variety, dimension 3g-3 Open problem: Do ∞ many closed geodesic surfaces Teichmüller metric ⇒ M is arithmetic? There exists a holomorphic, isometrically immersed complex geodesic f : H 2 → M g through every point in every possible direction. Example of a complex geodesic f : H 2 → M 3 Planes in M g f : H 2 → M g = T g / Mod g τ 1 a τ 2 Theorem (M, Eskin-Mirzakhani-Mohammadi, Filip) b 2002, f( τ 1 ) The closure of f( H 2 ) is an algebraic f( τ 2 ) 2014 H subvariety of moduli space. Example: For g=2 , the closure of f( H 2 ) can be b a Teichmüller curve, a Hilbert modular surface, a or the whole space. f( τ ) = Polygon( τ )/gluing = genus 3 X( τ ) The Hilbert modular surface is not totally geodesic.

Totally geodesic subvarieties Known geodesic subvarieties in M g M g ⊂ P N is a projective variety I. Covering constructions II. Teichmüller curves Almost all subvarieties V ⊂ M g are f contracted. ~ f M g M h M g Y H PROBLEM d V= H / Γ What are the totally geodesic * subvarieties M h X V ⊂ M g ? finite area Im(f) = a totally geodesic subvariety Im(f) = a totally geodesic (*Every complex geodesic tangent to V is contained in V.) curve ~ Example: M 1,2 → M 1,3 Klein quartic Example of a Teichmüller curve τ 1 a f M 3 H b f( τ 1 ) H V= H / Γ (2,7, ∞ ) = the Klein quartic! b a Helaman Ferguson, 1993 Thurston, MSRI director, 1992-1997 168 = 7x24 = |PSL 2 (Z/7)|

Totally geodesic varieties in moduli space Proof that F does not exist V be a totally geodesic hypersurface in M g. Let * V, let q 0 ,...q n be a basis for Q(X) = T X M g . Given [X] in Theorem Assume q 0 generates the normal bundle to V. There is a primitive, totally geodesic complex surface F (the flex locus) Then the highly nonlinear condition : properly immersed into M 1,3. P a i q i Z | P a i q i | = 0 q 0 X Is equivalent to a linear condition of the form X a i b i = 0 . A TREATISE 1 st example of a Teichmüller surface 1879 ON THE The flex locus F ⊂ M 1,3 HIGHER PLAM CURVES : is the set of INTENDED AS A SEQUEL TO (A,P) in M 1,3 : A TREATISE ON CONIC SECTIONS. 1 ∃ degree 3 map π :A → P such that BY (i) P ~ Z = any fiber of π ; and GEORGE SALMON, D.D., D.C.L., LL.D., F.R.S., REGIUS PROFESSOR OF DIVINITY IN THE UNIVERSITY OP DUBLIN. (ii) P ⊂ cocritical points of π . THIRD EDITION. What is the dimension of F? Is F irreducible? .... HODGES, FOSTER, AND FIGGIS, GKAFTON STREET, BOOKSELLERS TO THE UNIVERSITY. MJDCCCLXXIX,

The Polar Conic The Hessian 4 4 4 4 4 = Z(det D 2 f) 2 2 2 2 2 HA A 0 0 0 0 0 A S - 2 - 2 - 2 - 2 - 2 Polar(S,A) - 4 - 4 - 4 - 4 - 4 HA = {S : Pol(A,S) is 2 lines} - 4 - 4 - 4 - 2 - 2 - 2 0 0 0 2 2 2 4 4 4 - 4 - 4 - 2 - 2 0 0 2 2 4 4 The Cayleyan ={lines in Pol(S,A), some S} The flex locus F ⊂ M 1,3 4 CA is: 2 CA ⟶ HA (A,P) in M 1,3 : 2 A P = double(L) ∩ A, for some L in CA 0 CA → F → M 1 - 2 - 4 Corollary: F is 2 dimensional. - 4 - 2 0 2 4

The Solar Configuration The Solar Configuration 6 6 4 4 2 2 Sun 0 0 - 2 - 2 - 4 - 4 - 6 - 6 6 4 2 0 2 4 6 6 4 2 0 2 4 6 Codawn The Solar Configuration The Solar Configuration 6 6 4 4 2 2 Dawn Dawn Sun Sun 0 0 - 2 - 2 Dusk - 4 - 4 - 6 - 6 F ⇔ {(A,Codawn)} 6 4 2 0 2 4 6 6 4 2 0 2 4 6

The gothic locus Ω G ⊂ Ω M 4 From Ω G to F (X, ω ) in Ω G → (A,q) = (X/J, ω 2 /J) Ω G = {(X, ω ) in Ω M 4 (2,2,2) : → (A,P = poles(q)) in F (i) ∃ J with A=X/J of genus 1; (ii) ω is odd for J; (iii) ∃ odd cubic map p: X → B, genus 1; G F (iv) p(Z( ω )) = one point. Theorem: Ω G is an SL 2 ( R ) invariant 4-manifold. Corollary: F is totally geodesic Known Teichmüller curves Cathedral polygons . . . Wright . . . . . . M 6 ? M 5 2a b a a 1 . . . MMW 2016 1 M 4 . . . 2 1 M 2005 (Prym) M 3 . . . b Calta, M 2002 M 2 . . . Theorem Complement. (Jacobian) For every real quadratic field K = Q ( √ d), We have a new infinite series of E 6 , Veech 1989 Teichmüller curves in (the gothic locus G ⊂ ) M 4. there exists a,b in K such that P(a,b)/~ E 7 , V in M 4 . Bouw-Möller 2006 generates a Teichmüller curve E 8

Q. What are the totally geodesic surfaces in M 1,3 ? Why does F exist?