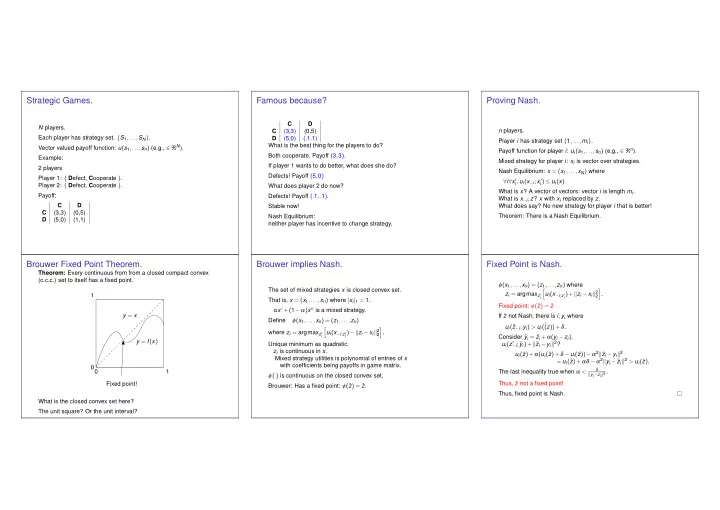
Strategic Games. Famous because? Proving Nash. C D N players. n - PowerPoint PPT Presentation

Strategic Games. Famous because? Proving Nash. C D N players. n players. C (3,3) (0,5) Each player has strategy set. { S 1 ,..., S N } . D (5,0) (.1.1) Player i has strategy set { 1 ,..., m i } . What is the best thing for the players to do? Vector valued payoff function: u ( s 1 ,..., s n ) (e.g., ∈ ℜ N ). Payoff function for player i : u i ( s 1 ,..., s n ) (e.g., ∈ ℜ n ). Both cooperate. Payoff ( 3 , 3 ) . Example: Mixed strategy for player i : x i is vector over strategies. If player 1 wants to do better, what does she do? 2 players Nash Equilibrium: x = ( x 1 ,..., x N ) where Defects! Payoff ( 5 , 0 ) Player 1: { D efect, C ooperate } . ∀ i ∀ x ′ i , u i ( x − i ; x ′ i ) ≤ u i ( x ) . Player 2: { D efect, C ooperate } . What does player 2 do now? What is x ? A vector of vectors: vector i is length m i . Payoff: Defects! Payoff ( . 1 ,. 1 ) . What is x − i ; z ? x with x i replaced by z . C D What does say? No new strategy for player i that is better! Stable now! C (3,3) (0,5) Theorem: There is a Nash Equilibrium. Nash Equilibrium: D (5,0) (1,1) neither player has incentive to change strategy. Brouwer Fixed Point Theorem. Brouwer implies Nash. Fixed Point is Nash. Theorem: Every continuous from from a closed compact convex (c.c.c.) set to itself has a fixed point. φ ( x 1 ,..., x n ) = ( z 1 ,..., z n ) where The set of mixed strategies x is closed convex set. � � i )+ � z i − x i � 2 z i = argmax z ′ u i ( x − i ; z ′ . 1 2 i That is, x = ( x 1 ,..., x n ) where | x i | 1 = 1. Fixed point: φ (ˆ z ) = ˆ z α x ′ +( 1 − α ) x ′′ is a mixed strategy. y = x If ˆ z not Nash, there is i , y i where Define φ ( x 1 ,..., x n ) = ( z 1 ,..., z n ) z − i ; y i ) > u i (ˆ u i (ˆ ( z ))+ δ . � � i ) −� z i − x i � 2 where z i = argmax z ′ u i ( x − i ; z ′ . 2 Consider ˆ y i = ˆ z i + α ( y i − z i ) . i y = f ( x ) u i ( ˆ z − i ; ˆ y i )+ � ˆ z i − y i � 2 ? Unique minimum as quadratic. z i is continuous in x . u i (ˆ z )+ α ( u i (ˆ z )+ δ − u i (ˆ z )) − α 2 � ˆ z i − y i � 2 Mixed strategy utilities is polynomial of entries of x z )+ αδ − α 2 � y i − ˆ z i � 2 > u i (ˆ = u i (ˆ z ) . with coefficients being payoffs in game matrix. 0 δ 0 1 The last inequality true when α < � y i − z i � 2 . φ ( · ) is continuous on the closed convex set. Thus, ˆ Fixed point! z not a fixed point! Brouwer: Has a fixed point: φ (ˆ z ) = ˆ z . Thus, fixed point is Nash. What is the closed convex set here? The unit square? Or the unit interval?

Sperner’s Lemma Proof of Sperner’s. n + 1-dimensional Sperner. For any n + 1-dimensional simplex which is subdivided into smaller simplices. One dimension: Subdivision of [ 0 , 1 ] . All vertices are colored { 1 ,..., n + 1 } . R : counts “rainbow” cells; has all n + 1 colors. Endpoints colored differently. The coloring is proper if the extremal vertices are differently colored. Odd number of multicolored edges. Q : counts “almost rainbow” cells; has { 1 ,..., n } . Note: exactly one color in { 1 ,..., n } used twice. Two dimensions. Each face only contains the colors of the incident corners. Consider ( 1 , 2 ) edges. Rainbow face: n − 1-dimensional, vertices colored with { 1 ,..., n } . Lemma: There exist a simplex that has all the colors. Separates two regions. X : number of boundary rainbow faces. Dual edge connects regions with 1 on right. Y : number of internal rainbow faces. Exterior region has excess out-degree: Number of Face-Rainbow Cell Adjacencies: R + 2 Q = X + 2 Y one more ( 1 , 2 ) than ( 2 , 1 ) . There exist a region with excess in-degree. Rainbow faces on one face of big simplex. Induction = ⇒ Odd number of rainbow faces. ( 1 , 2 , 1 ) triangle has in-degree=out-degree. → X is odd → X + 2 Y is odd R + 2 Q is odd. ( 2 , 1 , 2 ) triangle has in-degree=out-degree. R is odd. Oops. Must be ( 1 , 2 , 3 ) triangle. Where is multicolored? Must be odd number! Where is multicolored? And now? By induction! Sperner to Brouwer Rainbow Cells to Brower. Computing Nash Equilibrium. Consider simplex:S. Closed compact sets can be mapped to this. Let f ( x ) : S → S . PPAD - “Polynomial Parity Argument on Directed Graphs.” Rainbow cell, in S j with vertices x j , 1 ,..., x j , n + 1 . Infinite sequence of subdivisions: S 1 , S 2 ,... j “Graph with an unbalanced node (indegree � = outdegree) must have Each set of points x j S j is subdivision of S j − 1 . Size of cell → 0 as j → ∞ . i is an infinite set in S . another.” A coloring of S j . Recall ∑ i x i = 1 in simplex. → convergent subsequence → has limit point. Exponentially large graph with vertex set { 0 , 1 } n . Big simplex vertices e j = ( 0 , 0 ,..., 1 ,..., 0 ) get j . → All have same limit point as they get closer together. x ∗ is limit point. Circuit given name of graph finds previous, P ( v ) , and next, N ( v ) . For a vertex at x . Sperner: local information gives neighbor. Assign smallest i with f ( x ) i < x i . f ( x ) has no fixed point = ⇒ f ( x ) i ≥ x i for some i . ( ∑ i x i = 1). Exists? Yes. ∑ i f ( x ) i = ∑ i x i . END OF THE LINE. Given circuits P and N as above, if O n is But f ( x j , i ) i < x j , i for all j and i unbalanced node in the graph, find another unbalanced node. Valid? Simplex face is at x j = 0 for opposite j . lim j → ∞ x j , i = x ∗ . Thus f ( x ) j cannot be smaller and is not colored j . PPAD is search problems poly-time reducibile to END OF LINE. Thus, ( f ( x ∗ )) i ≤ x ∗ i by continuity. Contradiction. Rainbow cell, in S j with vertices x j , 1 ,..., x j , n + 1 . NASH → BROUWER → SPERNER → END OF LINE ∈ PPAD.

Other classes. PPA: “If an undirected graph has a node of odd degree, it must have another. PLS: “Every directed acyclic graph must have a sink.” PPP: “If a function maps n elements to n − 1 elements, it must have a collision.” All exist: not NP !!! Answer is yes. How to find quickly? Reduction: END OF LINE → Piecewise Linear Brouwer → 3 D − Sperner → Nash. Uh oh. Nash is PPAD-complete. Who invented? PapaD and PPAD. Perfect together!