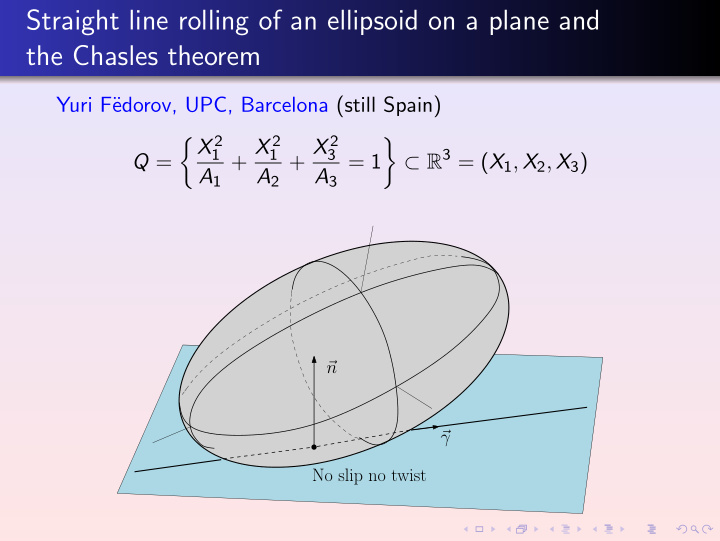
Straight line rolling of an ellipsoid on a plane and the Chasles - PowerPoint PPT Presentation

Straight line rolling of an ellipsoid on a plane and the Chasles theorem Yuri F¨ edorov, UPC, Barcelona (still Spain) � X 2 + X 2 + X 2 � ⊂ R 3 = ( X 1 , X 2 , X 3 ) 1 1 3 Q = = 1 A 1 A 2 A 3 � n � γ No slip no twist

The Jacobi geodesic problem on the ellipsoid Q Linealization of the geodesic flow on Q (Jacobi and Weierstrass): Let λ 1 , λ 2 be the ellipsoidal coordinates on Q , ( A i − λ 1 )( A i − λ 2 ) X 2 i = A i ( A i − A j )( A i − A k ) , i = 1 , 2 , 3 . After time re-parametrization ds = λ 1 λ 2 ds 1 , reduction to quadratures (Jacobi, 1881) d λ 1 d λ 2 + = ds 1 , � � 2 R ( λ 1 ) 2 R ( λ 2 ) λ 1 d λ 1 λ 2 d λ 2 + = 0 , � � 2 R ( λ 1 ) 2 R ( λ 2 ) R ( λ ) = − λ ( λ − A 1 )( λ − A 2 )( λ − A 3 )( λ − c ) where c is a constant of motion such that the geodesic is tangent to the caustic Q ∩ Q c .

Geodesic flow on n − 1-dimensional quadric Family of confocal quadrics in R n ( X 1 , . . . , X n ) X 2 X 2 � � 1 n Q ( c ) = A 1 − c + · · · + A n − c = 1 , c ∈ R . Theorem (Chasles) Let X ( s ) be a geodesic on Q = Q (0) with a natural parameter s and � γ ( s ) = dX / ds be the tangent vector. Then 1) the tangent line ℓ = { X + t � γ | t ∈ R } is also tangent to n − 1 fixed confocal quadrics Q ( c 1 ) = Q (0) , Q ( c 2 ) , . . . , Q ( c n − 1 ). 2) Let � q j be a unit normal vector of Q ( c j ) at the contact point p j = ℓ ∩ Q ( c j ). The vectors � q 1 , . . . ,� q n − 1 , γ form an orthogonal frame in R n .

Theorem (following J.Moser) When X ( s ) traces a geodesic on Q , the evolution of � q 1 , . . . ,� q n − 1 , γ is described by d d ds � q j = − Ω� q j , j = 1 , . . . , n − 1 , ds � γ = − Ωγ, Ω = � X , A − 2 X � A − 1 X ∧ A − 1 γ = � q ∧ A − 1 γ . Corollary. In the reference frame R = { � q 1 , . . . ,� q n − 1 , γ } the ellipsoid Q rolls on the hyperplane H = span( � q 1 2 , . . . ,� q n − 1 , γ ) without slipping and twisting. On H the contact point Q ∩ H moves alongs the line L =span( � γ ). On the ellipsoid Q the contact point traces the geodesic X ( s ). In the frame R the angular velocity of Q has the form   0 Ω 12 · · · Ω 1 , n Ω 12 0 · · · 0   ¯ Ω =  . . .  ... . . .   . . .   Ω 1 , n 0 · · · 0 For n = 3 the angular velocity vector of Q satisfies � ¯ ω,� q 1 � = 0

Relation with the Neumann system (H. Kn¨ orrer) Let X ( s ) be a geodesic on Q , and q ( s ) = q 1 ( s ) be the normal unit vector of Q at the point X . Then q ( s 1 ) is a solution of the Neumann problem on S n − 1 = {� q , q �} = 1 with H = 1 q � − � q , Iq � ), I = A − 1 : 2 ( � ˙ q , ˙ d 2 q = Iq + ν q , ds 2 1 provided that ds = � X , A − 2 X � ds 1 .

Separation of variables for the Neumann system Let λ 1 , . . . , λ n − 1 be spheroconical coordinates on S n − 1 = {� q , q � = 1 } : i = ( I i − λ 1 ) · · · ( I i − λ n − 1 ) q 2 , � j � = i ( I i − I j ) then  d λ 1 d λ n − 1 + · · · + = 0 ,   � � 2 R ( λ 1 ) 2 R ( λ n − 1 )      · · · · · · λ n − 2 + · · · + λ n − 2  n − 1 d λ n − 2 d λ 1   1  = ds 1 ,   � � 2 R ( λ 1 ) 2 R ( λ n − 1 )  R ( λ ) = − ( λ − I 1 ) · · · ( λ − I n ) · ( λ − C 1 ) · · · ( λ − C n − 1 ) , C 1 = 0 , C j = 1 / c j j = 2 , . . . , n − 1

The rotation matrix of Q in the fixed frame R = { � q 1 , . . . ,� q n − 1 , γ } : q n − 1 γ ) T ∈ SO ( n ) R = ( � q 1 · · · � in terms of points P 1 = ( λ 1 , µ 1 ) , . . . , P n − 1 = ( λ n − 1 , µ n − 1 ) on the genus g = n − 1 hyperelliptic curve Γ : µ 2 = − ( λ − I 1 ) · · · ( λ − I n ) · ( λ − C 1 ) · · · ( λ − C n − 1 ) , C 1 = 0 � U ( I i ) q 1 , i = , i = 1 , . . . , n , � Ψ ′ ( I i ) n − 1 � � U ( I i ) U ( C s ) � µ k q s , i = ( I i − λ k )( C s − λ k ) , s = 2 , . . . , n − 1 , � � Ψ ′ ( I i ) ψ ′ ( C s ) k =1 n − 1 � � U ( I i ) U (0) � µ k γ i = ( I i − λ k ) λ k , � � Ψ ′ ( I i ) ψ ′ (0) k =1 where U ( r ) = ( r − λ 1 ) · · · ( r − λ n − 1 ) , Ψ( λ ) = ( λ − I 1 ) · · · ( λ − I n ) , ψ ( λ ) = ( λ − C 1 ) · · · ( λ − C n − 1 ) . ( Following Yu. F., B. Jovanovi´ c. J. Nonl. Sci. 2004)

The angular velocity of Q in the frame R = { � q 1 , . . . ,� q n − 1 , γ }   0 Ω 12 · · · Ω 1 , n Ω 12 0 · · · 0   ¯ Ω =  . . .  ... . . .   . . .   Ω 1 , n 0 · · · 0 with n − 1 � � U (0) U ( C s ) µ k � Ω 1 , s = λ k ( C s − λ k ) , s = 2 , . . . , n − 1 , � � ψ ′ (0) ψ ′ ( C s ) k =1 � U (0) Ω 1 , n = . � ψ ′ (0) The coordinates of the center of Q in R : ( � X , q 1 � , · · · , � X , q n − 1 � , s + � X , γ � ) , � � X = λ 1 · · · λ n − 1 Aq 1 , s = λ 1 · · · λ n − 1 ds 1 .

The angular velocity of the frame R = { � q 1 , . . . ,� q n − 1 , γ } with respect to the axes { X 1 , . . . , X n } of the ellipsoid Q : n − 1 � � U ( I i ) U ( I j ) µ k � ω ij = ( I i − λ k )( I j − λ k ) , 1 ≤ i < j ≤ n . � � Ψ ′ ( I i ) Ψ ′ ( I j ) k =1

Theta-function solution Let B be the g × g period matrix of the genus g = n − 1 curve Γ : µ 2 = − ( λ − I 1 ) · · · ( λ − I n ) · ( λ − C 1 ) · · · ( λ − C n − 1 ) , C 1 = 0 . Introduce the corresponding theta-function � θ ( z | B ) = exp( � BM , M � / 2 + � M , z � ) , M ∈ Z g g g � � z ∈ C g , � M , z � = M i z i , � BM , M � = B ij M i M j , i =1 i , j =1 as well as theta-functions with characteristics α = ( α 1 , . . . , α g ), β = ( β 1 , . . . , β g ), α j , β j ∈ R , which are obtained from θ ( z | B ) by shifting the argument z and multiplying by an exponent: � α � θ ( z ) = exp {� B α, α � / 2 + � z + 2 πβ, α �} θ ( z + 2 πβ + B α ) . β

Theta-function solution (II) • Half-integer theta-characteristics η i = [ η ′′ i , η ′ i ] such that � ( I i , 0) � ( C i , 0) 2 π η ′′ i + B η ′ i = ω ¯ or = ω ¯ ∞ ∞ The rotation matrix of Q in the fixed frame R is  θ [ η I 1 ]( z ) θ [ η I 1 + η C 2 ]( z ) θ [ η I 1 + η C 1 ]( z )  σ 1 ε 1 κ 1 θ ( z ) θ ( z ) θ ( z )     θ [ η I 2 ]( z ) θ [ η I 2 + η C 2 ]( z ) θ [ η I 2 + η C 1 ]( z )   R = σ 2 ε 2 κ 2 ,   θ ( z ) θ ( z ) θ ( z )    θ [ η I 3 ]( z ) θ [ η I 3 + η C 2 ]( z ) θ [ η I 3 + η C 1 ]( z )   σ 3 ε 3  κ 3 θ ( z ) θ ( z ) θ ( z ) v ∈ C n , z 0 = const ∈ C n . z = v s 1 + z 0 ,