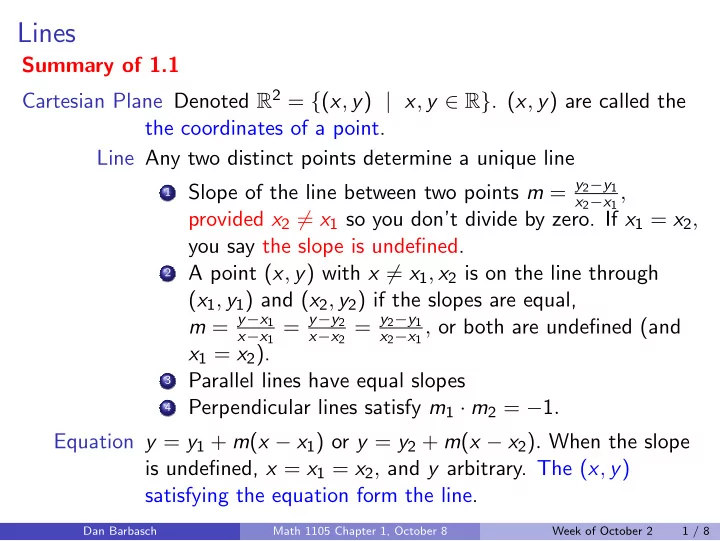

Lines Summary of 1.1 Cartesian Plane Denoted R 2 = { ( x , y ) | x , y ∈ R } . ( x , y ) are called the the coordinates of a point. Line Any two distinct points determine a unique line 1 Slope of the line between two points m = y 2 − y 1 x 2 − x 1 , provided x 2 � = x 1 so you don’t divide by zero. If x 1 = x 2 , you say the slope is undefined. 2 A point ( x , y ) with x � = x 1 , x 2 is on the line through ( x 1 , y 1 ) and ( x 2 , y 2 ) if the slopes are equal, m = y − x 1 x − x 1 = y − y 2 x − x 2 = y 2 − y 1 x 2 − x 1 , or both are undefined (and x 1 = x 2 ). 3 Parallel lines have equal slopes 4 Perpendicular lines satisfy m 1 · m 2 = − 1 . Equation y = y 1 + m ( x − x 1 ) or y = y 2 + m ( x − x 2 ) . When the slope is undefined, x = x 1 = x 2 , and y arbitrary. The ( x , y ) satisfying the equation form the line. Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 1 / 8
Examples I Example (Fahrenheit and Celsius) F = 32 + 9 5 C , C = 5 9( F − 32) . We say that F and C satisfy a linear relation. Graphically it is the line determined by (0 , 32) and (100 , 212) . One is the freezing temperature of water, the other is the boiling temperature of water. Example (35 in 1.1) Do the points (1 , 4) , (3 , 2) , (1 , 2), (0 2), and ( − 18 , − 12) lie on the same line? Explain why or why not. Do the points (1 , 4) , (3 , 2) and ( − 18 , − 12) form a right triangle? Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 2 / 8
Examples II Example (44 in 1.1) Consider the equation x a + y b = 1. a. Show that the equation represents a line by writing it as y = mx + b . b. Find the x − and y − intercepts of this line. c. Explain in your own words why the equation in this exercise is known as the intercept form of a line. Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 3 / 8
Examples III Example (72 in 1.1. Global Warming) In 1990, the Intergovernmental Panel on Climate Change predicted that the average temperature on Earth would rise 0 . 3 ◦ C per decade in the absence of international controls on greenhouse emissions. Let t measure the time in years since 1970, when the average global temperature was 15 ◦ C . Source: Science News. a. Find a linear equation giving the average global temperature in degrees Celsius in terms of t, the number of years since 1970. b. Scientists have estimated that the sea level will rise by 65 cm if the average global temperature rises to 19 ◦ C . According to your answer to part a, when would this occur? Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 4 / 8
Linear Functions I Write a linear cost function for each situation. Identify all variables used. Example (22, in 1.2) 22. For a one-day rental, a car rental firm charges $44 plus 28 cents per mile. Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 5 / 8
Linear Functions II Example (48 in 1.2, Education Cost) The 2009 − 2010 budget for the California State University system projected a fixed cost of $486,000 at each of five off-campus centers, plus a marginal cost of $1140 per student. Source: California State University. a. Find a formula for the cost at each center, C ( x ) , as a linear function of x , the number of students. b. The budget projected 500 students at each center. Calculate the total cost at each center. c. Suppose, due to budget cuts, that each center is limited to $1 million. What is the maximum number of students that each center can then support? Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 6 / 8
Linear Systems of Equations I Example Solve x 1 + 2 x 2 + x 3 = 1 − x 1 + 3 x 2 + x 3 = 2 2 x 1 + x 2 + x 3 = 3 Example Solve � x 1 + 2 x 2 + x 3 = 1 − x 1 + 3 x 2 + x 3 = 2 Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 7 / 8
Linear Systems of Equations II Exercise Solve − x + y = 1 3 x − 2 y = 2 2 x − y = − 2 Find the triangle determined by the three lines in the linear system. Dan Barbasch Math 1105 Chapter 1, October 8 Week of October 2 8 / 8
Recommend
More recommend