Simulation II An infinite population of flocks each having its own - PDF document

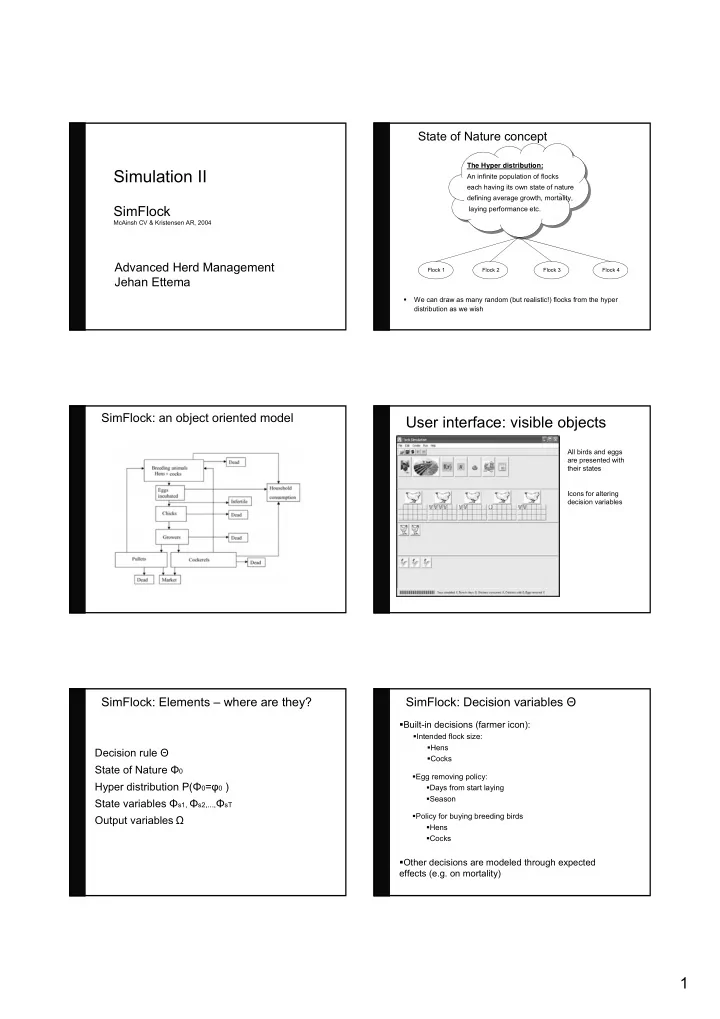
State of Nature concept The Hyper distribution: Simulation II An infinite population of flocks each having its own state of nature defining average growth, mortality, SimFlock laying performance etc. McAinsh CV & Kristensen AR, 2004 Advanced Herd Management Flock 1 Flock 2 Flock 3 Flock 4 Jehan Ettema � We can draw as many random (but realistic!) flocks from the hyper distribution as we wish SimFlock: an object oriented model User interface: visible objects All birds and eggs are presented with their states Icons for altering decision variables SimFlock: Elements – where are they? SimFlock: Decision variables Θ � Built-in decisions (farmer icon): � Intended flock size: � Hens Decision rule Θ � Cocks State of Nature Φ 0 � Egg removing policy: Hyper distribution P( Φ 0 = φ 0 ) � Days from start laying � Season State variables Φ s1 , Φ s2 ,..., Φ sT � Policy for buying breeding birds Output variables Ω � Hens � Cocks � Other decisions are modeled through expected effects (e.g. on mortality) 1

SimFlock: State of Nature Parameters The SimFlock survival rate model � In SimFlock, a state of nature is described by 42 � Logit (p ij ) = µ + α j + F i + ( α F) ij parameters: � µ = intercept � α 1 , α 2 , α 3 , α 4 systematic effects of bird groups � Survival rates, logistic model � F i ~ N(0, σ F ) random effect of flock Logit (pij) = µ + α j + Fi + ( α F)ij � ( α F) ij ~ N(0, σ α F ) random interaction flock and bird group � µ = intercept � Hyper parameters in Simflock: � α 1 , α 2 , α 3 , α 4 are the systematic effects of bird groups (i.e. chick, Intercept: µ: 0.2526 growers, pullets and cockerels) α 1 : 0.559 Effect chick: � F i ~ N(0, σ F ) is the random effect of flock α 2 : -0.398 Effect grower: � ( α F) ij ~ N(0, σ α F ) is the random interaction between flock and α 3 : 0.000 Effect pullet: bird group α 4 : 0.000 Effect cockerel: σ F : 0.156 Random effect flock: F i ~ N(0,0.156) σ α F: 0.557 Random interaction: ( α F) ij ~ N(0, 0.557) The SimFlock survival rate model The SimFlock survival rate model � Logit (p 11 ) = µ + α 1 + F 1 + ( α F) 11 : mortality chick (group 1), in flock 1 � Logit (p 11 ) = µ + α 1 + F 1 + ( α F) 11 : mortality chick (group 1), in flock 1 � Hyper parameters in Simflock: � Hyper parameters in Simflock: Intercept: µ: 0.2526 Intercept: µ: 0.2526 α 1 : 0.559 α 1 : 0.559 Effect chick: Effect chick: Random effect flock: F 1 ~ N(0,0.156): draw sample > 0.111 Random effect flock: F 1 ~ N(0,0.156): draw sample > 0.050 Random interaction: ( α F) 11 ~ N(0, 0.557) draw sample > -0.222 Random interaction: ( α F) 11 ~ N(0, 0.557) draw sample > 0.345 � Logit (p 11 ) = 0.2526 + 0.559 + 0.111 + -0.222 � Logit (p 11 ) = 0.2526 + 0.559 + 0.050 + 0.345 � Logit (p 11 ) = 0.7006 = y ij � Logit (p 11 ) = 1.2066 = y ij � P 11 =1/(e -y ij + 1) = 0.668 = survival rate for chick: � P 11 =1/(e -y ij + 1) = 0.770 = survival rate for chick: 1 parameter of the State of Nature...1 down, 41 to go! SimFlock: State of Nature Parameters SimFlock: State of Nature Parameters � In SimFlock, a state of nature is described by 42 � In SimFlock, a state of nature is described by 42 parameters: parameters: � Daily gains of birds, general linear model � Full grown weights, normal distribution Y ijk = µ + α i + F j + ( α F) ij + B K � Age at puberty � Number of eggs before incubation � Egg fertilization probability, beta distribution N ~ (µ, σ 2) Beta ~ (a,b) � Egg hatching probability, logistic model Logit (pij) = µ + A i � Each time a parameter is defined, a hyper distribution is specified. 2

SimFlock: Hyper distribution SimFlock: Hyper distribution � The hyper distribution of the state of nature is specified � Most of the hyper parameters estimated from the field data through 64 hyper parameters. collected in 30 flocks. � Logit (p ij ) = µ + α j + F i + ( α F) ij � Survival rate for 4 groups (j=1,2,3,4): 4 State of Nature parameters � The hyper distribution represents the whole population of flocks under the conditions in question. � 7 Hyper parameters: Intercept: µ: 0.2526 � A state of nature drawn from the hyper distribution α 1 : 0.559 Effect chick: represents one (hypothetical) flock. α 2 : -0.398 Effect grower: � By drawing e.g. many states of nature we can generate α 3 : 0.000 Effect pullet: many realistic hypothetical flocks. α 4 : 0.000 Effect cockerel: � Decision rules may have different effects in different σ F : 0.156 Random effect flock: F i ~ N(0,0.156) flocks. σ α F: 0.557 Random interaction: ( α F) ij ~ N(0, 0.557) SimFlock: State variables States of a bird � The state variables of day i are the states of the individual � All birds: birds and eggs on that day: � Unique ID (given at hatching, next integer) � Eggs: � Age (updated daily) � Fertilized /not fertilized � Weight (updated daily) � Birds: � Gender (drawn at random hatching) � Age � Full grown weight (drawn at random at hatching) � Weight � Grown potential (permanent, drawn at hatching) � Growth potential � Full grown weight � Chicks and growers: � Laying capacity � Growth state (drawn at hatching/transition) � Gender � Farmer: � Cocks: � Needs meat � No further states � There are millions of state variables in a simulation run States of a pullet and cockerel States of a Hen � In addition to the general states: � In addition to the general states: � Pullet � Laying capacity (drawn at transition) � Age at first egg ”puberty” (drawn at transition) � State in cycle (laying, incubating, brooding, � Growth state (drawn at transition) barren) – updated daily. � Days since transition in cycle – updated daily � Cockerel � Eggs at incubating (drawn at transition in cycle) � Age at ”puberty” (drawn at transition) � Eggs in nest – updated daily. � Growth state (drawn at transition) � Fertile eggs in nest – updated daily. � Behavior, not used? (drawn at transition) 3

SimFlock: Output variables Ω SimFlock: Simulation � The farmer, birds and eggs are represented as � A total of 40 are defined: objects in the model � Realised gain � Realised mortality � Each simulated day, the states of all objects are � Eggs removed updated: � Chickens produced � Age � ... � Weight � Survival � Usual technical and economical key figures � Transition (e.g. egg → chick, chick → grower, etc.) � Total income � Eggs in the nest � Total costs � ... � Net returns � ... Use of the simulation model System comprehension � Usually carried out under one state of nature � System comprehension � Answer questions like: � Answering ”what if” questions � If we assume the state of nature parameters are Φ 0 = φ i , what � What if decision rules Θ change are then the consequences? � What if we could improve the survival rate of chicks? � General decision support (at population level) � Vary the survival rate systematically – run simulations and � Main purpose of SimFlock explore the results � Etc. � Decision support at (specific) flock level � Weakness: State of nature parameters are mutually � Not yet possible correlated! General decision support Decision support at flock level � Should “Jens Hansen” change his management from � Population level decision rule Θ 1 to Θ 2 ? � Carried out under multiple states of nature � Questions like: � Problems: � Under what circumstances does it pay to change the decision � We don’t know the state of nature for JH’s flock rule from Θ 1 to Θ 2 ? � Mortality in his flock? � Generate multiple states of nature (Random flocks) � Age at puberty in his flock? � Run a simulation job under Θ 1 � Not so difficult in SimFlock (only) 42 parameters � Run a simulation job under Θ 2 � Tough in SimHerd (Kudahl) 353 parameters � Identify the states of nature where it pays � Not yet possible in any simulation model? � In Simherd it has been done: generalize, assume and ignore 4