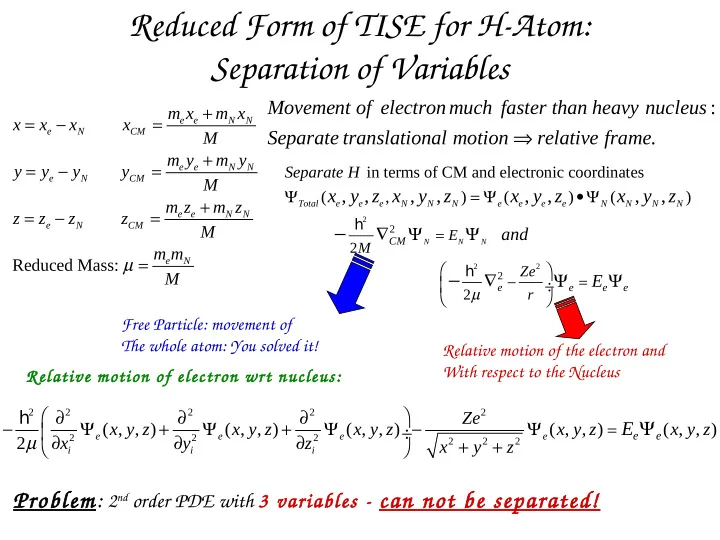
Reduced Form of TISE for H-Atom: Separation of Variables Movement - PowerPoint PPT Presentation

Reduced Form of TISE for H-Atom: Separation of Variables Movement of electronmuch faster than heavy nucleus : + m x m x = − = x x x x e e N N ⇒ e N CM Separate translational motion relative frame . M + m y m y = − = y y y y e e N N Separate H in terms of CM and electronic coordinates e N CM M Ψ x y z x , , , y , z = Ψ x y z , , •Ψ x , y , z ( , ) ( ) ( ) + m z m z Total e e e N N N e e e e N N N N = − = z z z z e e N N 2 h e N CM − M ∇ Ψ Ψ 2 and = E CM N N N 2 M m m µ = Reduced Mass: e N   2 2 h Ze − M ∇ Ψ Ψ 2 E − =  ÷ e e e e µ  2 r  Free Particle: movement of The whole atom: You solved it! Relative motion of the electron and With respect to the Nucleus Relative motion of electron wrt nucleus:   ∂ ∂ ∂ h 2 2 2 2 2 Ze Ψ − Ψ + Ψ + Ψ − Ψ E ( , , ) x y z ( , , ) x y z ( , , ) x y z ( , , ) x y z = ( , , ) x y z  ÷ e e µ ∂ e ∂ e ∂ e e 2 2 2 2 x y z + +   2 2 2 x y z i i i Problem: 2 nd order PDE with 3 variables - can not be separated!

Spherical Polar Coordinates Conversion from Cartesian coordinates Used for spherically symmetric systems

Hamiltonian: Spherical Polar Coordinates Looks can be deceiving! Looks can be deceiving! Solve this PDE  need to separate variables r , θ, φ : POSSIBLE

TISE for H-Atom in spherical-polar coordinates   ∂ ∂ ∂ ∂ ∂ 2     1 1 1 Ψ θ φ + θ Ψ θ φ + Ψ θ φ 2 r ( , , ) r sin ( , , ) r ( , , ) r   ÷  ÷  ∂ ∂ θ ∂ θ ∂ θ θ ∂ φ 2 2 2 r r  r  sin   sin   = ( , , ) 0   µ 2 2 Ze + + Ψ θ φ E r  ÷ h 2 r   2 nd order Partial Differential Equation with three variables µ µ µ µ = + θ + φ Ψ θ φ = Θ θ Φ φ Special olution if H s H r ( ) H ( ) H ( ) : ( , , ) r R r ( ) ( ) ( )       d d 1 d d [ ] [ ] Θ θ Φ φ + θ Θ θ Φ φ 2 r R r ( ) ( ). ( ) sin ( ) R r ( ). ( )  ÷  ÷   θ θ θ dr  dr  sin d  d  1     2 r 2 1 d [ ] + Φ φ Θ θ ( ) R r ( ). ( )   θ φ 2 2 sin d   = ( ) 0   µ 2 2 Ze + + Θ θ Φ φ E R ( ) ( ) r  ÷ 2 h r  

Separation of Variables r , θ , φ ΘΦ R . . Ψ θ φ = Θ θ Φ φ = ( , , ) r R r ( ). ( ). ( ) Θ Φ  R        Φ Θ µ 2 2 1 d d R d d R d 2 Ze ΘΦ R ΘΦ + θ + + + = 2 r sin E 0    ÷  ÷  ÷ θ θ θ θ φ 2 2 2 2 r dr dr sin d d sin d h r         θ 2 2 r sin Multiply by and rearrange : Θ Φ R . .   θ θ Θ µ Φ 2     2 2 sin d dR sin d d 2 Ze 1 d + θ + + θ = − 2 2 2 r sin E r sin  ÷  ÷  ÷ Θ θ θ Φ φ 2 2 h R dr  dr  d  d  r d   = θ = φ = ⇒ θ = φ = = 2 LHS F r ( , ) G ( ) RHS F r ( , ) G ( ) Const . m ( say ) ∂ Φ 2 1 Solve 2 nd order DE to ∴ + = 2 m 0 obtain functional form of Φ ∂ φ 2 Φ(φ)

Solving φ -part is relatively simple! ∂Φ = ± Φ Q im ∂ 2 1 ± φ ∂ φ im ∴ Φ φ + = 2 A e ( ) m 0 Φ φ = : ( ) Solution Φ φ ∂ φ 2 ( ) ∂ Φ = − 2 Φ 2 & m ∂ φ 2 Boundary Condition Another Quantum Number “popped out” out of Boundary Conditions: Quantization of Angular Momentum! Magnetic Quatnum Number: Can take any integral value (including zero); Restricted by another quantum number (m<l). Loosely relates to direction of orbital angular momentum; splitting of energy levels in magnetic field.

Solving R(r) and Θ ( θ ) part not so simple, but can be done (in ~5-6 lectures!)   θ θ ∂ Θ µ 2 2 2     sin d dR sin d 2 r Ze − 2 m + θ + + θ = 2 2 r sin E sin 0  ÷  ÷  ÷ Θ ∂ θ θ 2 h R dr  dr   d  r   θ 2 Simply d ivide by sin and rearrange :   µ Θ   2 2 2   1 d dR 2 r Ze m 1 d d + + = − θ = β 2 r E sin ( const .)  ÷  ÷  ÷ θ Θ θ θ θ h 2 2 R dr  dr  r sin sin d  d    Boundary Only θ : Can be solved Only r : Can be solved conditions to obtain Θ(θ) part to obtain R(r) part applied! Need mathematical skills to solve the Differential Equations for R(r) and Θ ( θ ) - it will take several lectures to do so….

Θ(θ)Φ(φ) are Spherical Harmonics Y l m ( θ,φ ) Easier to solve if written differently: Rigid-Rotor  already solved the angular ( θ, φ ) part: Related to Angular Momentum! ·   ∂ ∂ ∂ 2 1 1 µ θ + Ψ θ φ = Ψ θ φ 2 sin ( , ) L ( , )   θ ∂ θ ∂ θ θ ∂ φ 2 2 sin sin   L 2  Square of angular momentum: Eigenfunctions “Spherical Harmonics” l=Azimuthal Quantum Number or orbital quantum number l ≤ n-1

Solve for R(r): Quantized Energies Radial Wavefunction depends on n and l: n= Principal Quantum Number=1,2,3,… Energies: E n : Essentially same as Bohr’s Equation, with slight changes Only n dependence of E: V term in H is needed for providing energies as eigenvalues. Angular parts does not have it!!!

Particle in 3D-Box:Three Quantum Numbers 3 Quantum Numbers needed to Describe the system completely Normalization Conditions (each dimension)

How to obtain normalized Ψ n,l,m ( r ,θ,π) ?

Loose Meaning of Quantum Numbers L=0  s-orbital L=1  p-orbital L=2  d-orbital L=3  f-orbital

Radial Solutions depend on n and l (l=n-1)   Zr na −    ÷ r  ÷ 2 Z + e = l 2 l 1 0   R A n l ( , ). r . L .  ÷ + nl n l   na 0 Exponential f(r,n,l,Z) g(r,Z) Decay g(r) Note: R nl  0 as r  infinity Additional restrictions on l: n ≥ l+1 arise when DE is solved

Complete Wavefunction of H-Atom Lets not get intimidated: Please do not even try to remember

H-Atom Complete Ψ (r, θ,φ ) for n=1,2 σ  r/a 0 F(r) only 1s 2s F(r) only 2p z F(r, θ ) 2p x, F(r, θ,φ ) y Linear combination Of two solutions is Also a solution