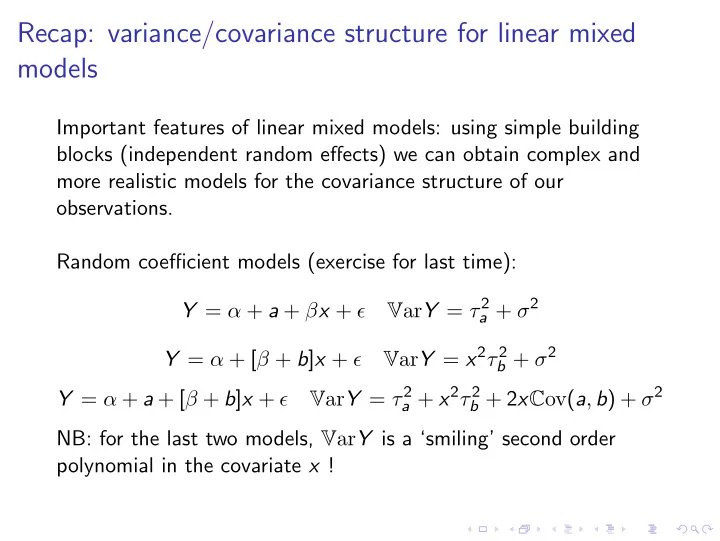
Recap: variance/covariance structure for linear mixed models - PowerPoint PPT Presentation

Recap: variance/covariance structure for linear mixed models Important features of linear mixed models: using simple building blocks (independent random effects) we can obtain complex and more realistic models for the covariance structure of our observations. Random coefficient models (exercise for last time): V ar Y = τ 2 a + σ 2 Y = α + a + β x + ǫ V ar Y = x 2 τ 2 b + σ 2 Y = α + [ β + b ] x + ǫ V ar Y = τ 2 a + x 2 τ 2 b + 2 x C ov ( a , b ) + σ 2 Y = α + a + [ β + b ] x + ǫ NB: for the last two models, V ar Y is a ‘smiling’ second order polynomial in the covariate x !

Variance is sum of covariances between random terms: V ar Y = V ar ( α + a + β x + bx + ǫ ) = C ov ( a , a ) + C ov ( a , bx ) + C ov ( bx , bx ) + C ov ( bx , a ) + C ov ( ǫ, ǫ ) = V ar ( a ) + V ar ( bx ) + V ar ǫ + 2 x C ov ( a , b ) = b + σ 2 + 2 x ρτ a τ b τ 2 a + x 2 τ 2 NB: a and b are assumed to be independent of ǫ so e.g. C ov ( a , ǫ ) = 0 NB: C ov ( a , b ) ρ = C orr ( a , b ) = √ ⇒ C ov ( a , b ) = ρτ a τ b V ar a V ar b

Orthodont data Distance (related to jaw size) between pituitary gland and the pterygomaxillary fissure (two distinct points on human skull) for children of age 8-14 Distance versus age: 30 25 distance 20 8 9 10 11 12 13 14 age

Orthodont data Distance (related to jaw size) between pituitary gland and the pterygomaxillary fissure (two distinct points on human skull) for children of age 8-14 Distance versus age grouped Distance versus age: according to child 30 30 25 distance 25 distance 20 20 8 9 10 11 12 13 14 8 9 10 11 12 13 14 age age Different intercepts for different children !

Variance of empirical mean for one-way anova (exercise 7) Covariances:  i � = i ′ 0   i = i ′ , j � = j ′ C ov [ Y ij , Y i ′ j ′ ] = (1) V ar U i  i = i ′ , j = j ′ V ar U i + V ar ǫ ij  ( km ) 2 V ar ¯ � Y ·· = C ov [ Y ij , Y i ′ j ′ ] = i , j , i ′ , j ′ � � V ar Y ij + C ov [ Y ij , Y i ′ j ′ ] = i , j i , j � = j ′ km ( σ 2 + τ 2 ) + km ( m − 1) τ 2 That is, = τ 2 k + σ 2 1 km σ 2 + km τ 2 + km ( m − 1) τ 2 � V ar ¯ � Y ·· = ( mk ) 2 km

Design of experiment (exercise 9) From expression of V ar ¯ Y ·· obvious that we should take k =100 if km (total number of experiments) fixed at 100. Suppose budget is 5000 kr. 200 fee for each lab and 10 kr. for each experiment. Then 5000 = k 200 + km 10 ⇔ m = 5000 − k 200 = 500 − 20 k 10 k k Thus we shall minimize Y ·· = 1 3 = 1 3 V ar ¯ k + k + k 500 − 20 k 500 − 20 k k This gives optimal k = 18.