Precision Neutrino Physics of the Future Alfons Weber University of - PowerPoint PPT Presentation

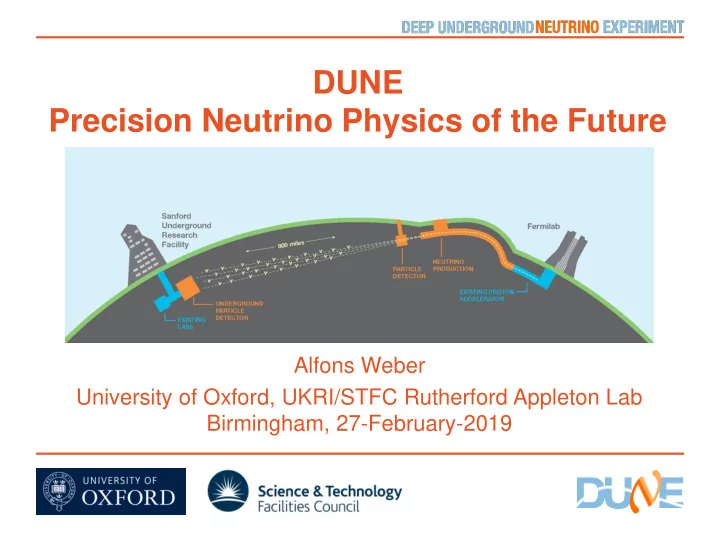
DUNE Precision Neutrino Physics of the Future Alfons Weber University of Oxford, UKRI/STFC Rutherford Appleton Lab Birmingham, 27-February-2019

Neutrino Mixing The PMNS Matrix • Assume that neutrinos do have mass: - mass eigenstates  weak interaction eigenstates - Analogue to CKM-Matrix in quark sector!       e 1      =  U      2            3   −         i 1 0 0 0 0 1 0 0 U U U c s e c s e 1 e 2 e 3  13 13  12 12         = = − i U U U U 0 c s  0 1 0  s c 0 0 e 0 2           1 2 3 23 23 12 12           − −  i i        U U U 0 s c s e 0 c 0 0 1 0 0 e   3    1 2 3 23 23 13 13 =  = = = ( θ ) θ Δm 2 2 with cos( ), sin , mixing angle and mass difference c s ij ij ij ij ij ij 2 27-Feb-2018 A.Weber | DUNE

The Who-is-Who æ ö æ ö æ ö æ ö s 13 e - i d c 13 c 12 s 12 0 0 ç ÷ ç ÷ 1 0 0 ç ÷ 1 0 0 ç ÷ ç ÷ ç ÷ ç ÷ i d 2 ç ÷ U = - s 12 c 23 s 23 c 12 e 0 0 1 0 0 0 0 ç ÷ ç ÷ ç ÷ ç ÷ ç ÷ - s 13 e i d i d 3 ç ÷ c 13 ç ÷ e ç ÷ - s 23 0 c 23 0 0 0 0 0 1 è ø è ø è ø è ø ν μ disappearance Solar neutrino oscillation ν– less double beta decay ν e appearance in ν μ beam Or reactor neutrino experiments 3 27-Feb-2018 A.Weber | DUNE

Mass Ordering Normal Inverted 4 27-Feb-2018 A.Weber | DUNE

Oscillations for Dummies    or      , 1 2              cos sin 2 2 1.27 m L  =  →  =  1 2         ( ) sin (2 )sin P    −           sin cos E   2 • Measure prob. - Survival - Appearance  =  − 2 3 2 m 3 10 eV • Result  = 2 sin ( 2 ) 1 = L 735 km - Mixing angle - Mass differences 5 27-Feb-2018 A.Weber | DUNE

Matter Effects • Simplified treatment: two neutrinos only In vacuum in matter       2 2 m L m L     →  =      →  =  2 2 2 2 m P ( ) sin ( 2 ) sin   P ( ) sin ( 2 ) sin   e e m   4 E   4 E ( )  ( ) sin 2  = with sin 2 ( ) ( ) m  − −  2 2 cos 2 sin 2 A ( ) ( )  =   − −  2 2 2 2 m m cos 2 A sin 2 m 2 2 G N E =  F e A  2 m • Matter modifies oscillation probability - Sign of mass difference matters (opposite for anti-v) - Larger effect at higher energies 6 27-Feb-2018 A.Weber | DUNE

The Full Monty • Life isn’t that easy - 3 Flavour oscillations    2 m L     →  =  2 2 P ( ) sin ( 2 ) sin   - Matter effects e   4 E • The full formula 7 27-Feb-2018 A.Weber | DUNE

The T2K Experiment • Neutrino Beam from j-parc - Beam power 50 – 480 kW • Far Detector - SuperKamiokande - 40 kton water Cherenkov 8 27-Feb-2018 A.Weber | DUNE

Producing Neutrinos off-axis (2.5°) (30 GeV from MR π→μν synchrotron) 118 m 0.43 E π E = + 0º ν 2 2 1 γ θ 9 27-Feb-2018 A.Weber | DUNE

Super-Kamiokande PID  - e 10 27-Feb-2018 A.Weber | DUNE

Muon Neutrino Disappearance Oscillation probability   L  →  = −   2 2 2   ( ) 1 sin (2 )sin 1.27 P m E      neutrinos anti-neutrinos 11 27-Feb-2018 A.Weber | DUNE

NOvA 12 27-Feb-2018 A.Weber | DUNE

NOvA Detector Concept 13 27-Feb-2018 A.Weber | DUNE

NOvA Events 14 27-Feb-2018 A.Weber | DUNE

NOvA Disappearance 15 27-Feb-2018 A.Weber | DUNE

A word of caution 16 27-Feb-2018 A.Weber | DUNE

The Happy Family 17 27-Feb-2018 A.Weber | DUNE

Electron Neutrino Appearance T2K NOvA 18 27-Feb-2018 A.Weber | DUNE

The Full Monty sin( δ ) changes sign for anti-neutrinos • δ is CP-violating phase • Matter  anti-matter difference 19 27-Feb-2018 A.Weber | DUNE

T2K Results 20 27-Feb-2018 A.Weber | DUNE

NOvA Results 21 27-Feb-2018 A.Weber | DUNE

General Setup • LBNF/DUNE will consist of - An intense 1.2 MW upgradeable 𝜉 -beam fired from Fermilab - A massive 68 kt (40kt instrumented) deep underground LAr detector in South Dakota and a large Near Detector at Fermilab - A large international collaboration 1300 km South Dakota Chicago     &  e STT� Module� Barrel� Backward� ECAL� Barrel� � ECAL� RPCs� End� FD Magnet� RPCs� Coils� Forward� ECAL� End� RPCs� ND 23 27-Feb-2018 A.Weber | DUNE

Physics Program • Neutrino Oscillations - Search for leptonic CP violation - Determine neutrino mass ordering - Precision PMNS measurements • Supernova Physics - Observation of time and flavour profile provides insight into collapse and evolution of supernova - Unique sensitivity to electron neutrinos • Baryon number violation - Predicted by many BSM theories - LAr TPC technology well-suited to certain proton decay channels ( e.g. , p→K+ 𝜉 ) - 𝛦 (B-L) ≠ 0 channels accessible ( e.g. , n→n̅) 24 27-Feb-2018 A.Weber | DUNE

The DUNE Collaboration Sep 2018 25 27-Feb-2018 A.Weber | DUNE

The DUNE Collaboration 26 27-Feb-2018 A.Weber | DUNE

Beam • Proton beam energy 60-120 GeV • Power 1.2 MW ➔ 2.4 MW • Neutrinos and anti-neutrinos 27 27-Feb-2018 A.Weber | DUNE

How to Measure Oscillations • Oscillation probabilities 𝑔𝑏𝑠 𝐹 𝜉 𝑔𝑏𝑠 𝐹 𝜉 𝜚 𝜉 𝑓 𝜚 𝜉 𝑓 𝑄 𝜉 𝜈 →𝜉 𝑓 𝐹 𝜉 = = 𝑜𝑓𝑏𝑠 𝐹 𝜉 ∗ 𝐺 𝑔𝑏𝑠,𝑜𝑝−𝑝𝑡𝑑 𝐹 𝜉 𝜚 𝜉 𝜈 𝑔𝑏𝑠/𝑜𝑓𝑏𝑠 (𝐹 𝜉 ) 𝜚 𝜉 𝜈 • Number of events/energy spectrum Well known (1-2%) 𝑒𝑓𝑢 𝑒𝑂 𝜉 𝑒𝑓𝑢 𝐹 𝜉 ∗ 𝜏 𝜉 𝜈 𝐵𝑠 𝐹 𝜉 = 𝜚 𝜉 𝜈 𝑒𝐹 𝜉 • In reality 𝑒𝑓𝑢 𝑒𝑂 𝜉 𝑢𝑏𝑠𝑕𝑓𝑢 𝐹 𝜉 ∗ 𝑈 𝑒𝑓𝑢 𝐹 𝜉 ∗ 𝜏 𝜉 𝑒𝑓𝑢 𝐹 𝑤 , 𝐹 𝑠𝑓𝑑 = න 𝜚 𝜉 𝑒𝐹 𝜉 𝜉 𝜈 𝑒𝐹 𝑠𝑓𝑑 • Folding of detector effects - Prevents (easy) cancellations of many systematic effects - Needs unfolding 28 May-2018 A.Weber | DUNE ND Status

Are there cancellations? • Oscillation signal Small theo. uncertainty or measurement 𝑔𝑏𝑠 𝑒𝑂 𝜉 𝑓 𝐵𝑠 𝐹 𝜉 𝜏 𝜉 𝑓 𝑒𝐹 𝑤 ൚ = 𝑄 𝜉 𝜈 →𝜉 𝑓 𝐹 𝜉 ∗ ∗ 𝐺 𝑔𝑏𝑠/𝑜𝑓𝑏𝑠 (𝐹 𝜉 ) 𝑜𝑓𝑏𝑠 𝐵𝑠 𝐹 𝜉 𝑒𝑂 𝜉 𝜈 𝜏 𝜉 𝜈 𝑒𝐹 𝑤 • Near muon/electron ratio 1-2% uncertainty 𝑜𝑓𝑏𝑠 𝑒𝑂 𝜉 𝑓 𝐵𝑠 𝐹 𝜉 𝑜𝑓𝑏𝑠 𝐹 𝜉 𝜏 𝜉 𝑓 𝜚 𝜉 𝑓 𝑒𝐹 𝑤 = ∗ ൚ 𝑜𝑓𝑏𝑠 𝐹 𝜉 𝐵𝑠 𝐹 𝜉 𝑜𝑓𝑏𝑠 𝑒𝑂 𝜉 𝜈 𝜚 𝜉 𝜈 𝜏 𝜉 𝜈 𝑒𝐹 𝑤 Not so small • Need to know uncertainty - Flux & cross section ratios - Far/near extrapolation A.Weber | DUNE ND Status 29 May-2018

But in Reality 𝑔𝑏𝑠 𝑒𝑂 𝜉 𝑓 𝑔𝑏𝑠 𝐹 𝑤 , 𝐹 𝑠𝑓𝑑 𝑜𝑓𝑏𝑠 𝐹 𝜉 ∗ 𝐺 𝐵𝑠 𝐹 𝜉 ∗ 𝑈 ׬ 𝑄 𝜉 𝜈 →𝜉 𝑓 𝐹 𝜉 ∗ 𝜚 𝜉 𝜈 𝑔𝑏𝑠/𝑜𝑓𝑏𝑠 (𝐹 𝜉 ) ∗ 𝜏 𝜉 𝑓 𝑒𝐹 𝜉 𝑒𝐹 𝑠𝑓𝑑 𝜉 𝑓 = 𝑜𝑓𝑏𝑠 𝐹 𝜉 ∗ 𝜏 𝜉 𝜈 𝐵𝑠 𝐹 𝜉 ∗ 𝑈 𝑜𝑓𝑏𝑠 𝐹 𝑤 , 𝐹 𝑠𝑓𝑑 𝑜𝑓𝑏𝑠 𝑒𝑂 𝜉 𝜈 ׬ 𝜚 𝜉 𝜈 𝑒𝐹 𝜉 𝜉 𝜈 𝑒𝐹 𝑠𝑓𝑑 • No cancellations - Unless you unfold • Need to understand especially - Detector effects in near and far detector - Relation of visible to neutrino energy - Cross section ratios - Near to far flux extrapolation • Flux normalisation cancels - Shape is more important 30 May-2018 A.Weber | DUNE ND Status

Near Detector Complex • Multiple Near Detectors - characterise beam & neutrino interactions & detector response - LAr TPC (similar to FD) - High pressure gaseous argon TPC tracker - Calorimeter and muon systems 27-Feb-2018 31 A.Weber | DUNE

ArgonCube 2X2 prototype (proto-DUNE-ND) Engineering concept In the laboratory in Bern First cool down starts next week Will be brought to Fermilab after testing at Bern. To be placed in the NuMI beam MINOS ND Hall 32 1/28/2019 Alan Bross | NDDG Status