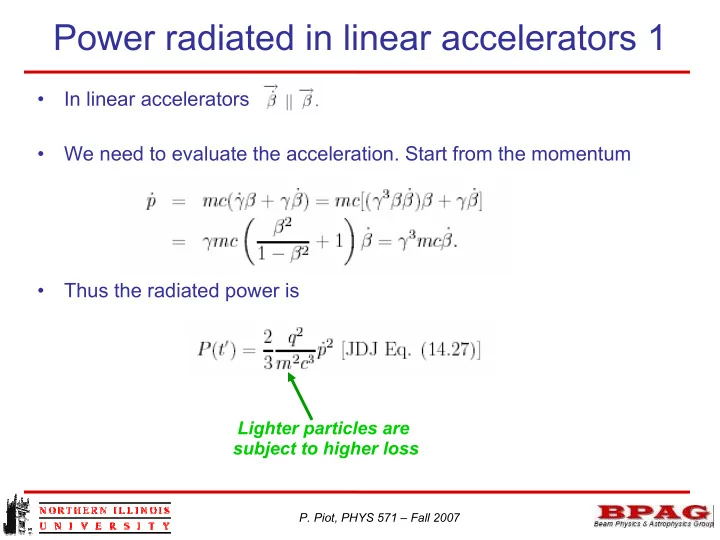
Power radiated in linear accelerators 1 In linear accelerators - PowerPoint PPT Presentation

Power radiated in linear accelerators 1 • In linear accelerators • We need to evaluate the acceleration. Start from the momentum • Thus the radiated power is Lighter particles are subject to higher loss P. Piot, PHYS 571 – Fall 2007

Power radiated in linear accelerators 2 • One important question is how does the emission of radiation influence the charge particle dynamics. • The accelerator induce a momentum change of the form (where we assumed the acceleration is along the z- axis) • Let be the power associated to the external force. The particle dynamics is affected when P ext is comparable to the radiated power: P. Piot, PHYS 571 – Fall 2007

Power radiated in linear accelerators 3 • Consider a relativistic electron then • ….. • So the effect seems to be negligible. • This is actually part of the story some coherent effect can kick in an induce some distortion when considering highly charged electron bunches for instance… P. Piot, PHYS 571 – Fall 2007

Power radiated in circular accelerators 1 • Now and • The radiated power is where E is the energy. Let’s introduce • So radiative energy loss per turn is P. Piot, PHYS 571 – Fall 2007

Power radiated in circular accelerators 2 • That is • For an e- synchrotron this becomes • Take E= 1 TeV, R= 2 km we have • Conclusion: – bad idea to build electron circular accelerator for HEP – but good as copious radiation sources (e.g. APS in Argonne). P. Piot, PHYS 571 – Fall 2007

Angular distribution of radiation emitted by an accelerated charge • Starting from the radiation field, we have where we used P. Piot, PHYS 571 – Fall 2007

Angular distribution for linear motion 1 Introducing θ , we have: • • And the numerator becomes • So the radiated power writes P. Piot, PHYS 571 – Fall 2007

Angular distribution for linear motion 2 • The power distribution has maxima given by • With solutions Only cos θ + is possible so: • P. Piot, PHYS 571 – Fall 2007

Angular distribution for linear motion 3 P. Piot, PHYS 571 – Fall 2007

Angular distribution for linear motion 4 • Small angle approxi- mation for ultra-relativistic case: JDJ equation 14.41 P. Piot, PHYS 571 – Fall 2007

Angular distribution for circular motion 1 • We have: • That is • Which gives: • In the ultra-relativistic limit (small angle approximation): P. Piot, PHYS 571 – Fall 2007

Angular distribution for circular motion 2 • Note that • So we have P. Piot, PHYS 571 – Fall 2007