Poincar e-Verdier duality Having proved Verdier duality, our next - PowerPoint PPT Presentation

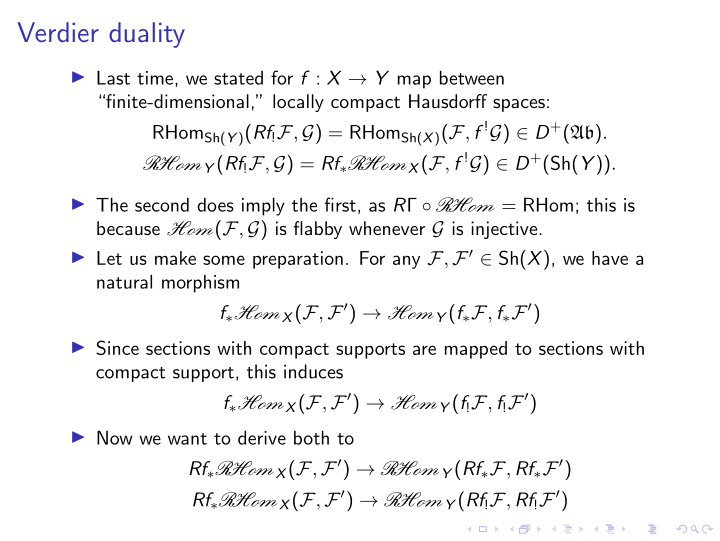
Verdier duality ◮ Last time, we stated for f : X → Y map between “finite-dimensional,” locally compact Hausdorff spaces: RHom Sh( Y ) ( Rf ! F , G ) = RHom Sh( X ) ( F , f ! G ) ∈ D + ( Ab ) . H om X ( F , f ! G ) ∈ D + (Sh( Y )) . R H om Y ( Rf ! F , G ) = Rf ∗ R ◮ The second does imply the first, as R Γ ◦ R H om = RHom; this is because H om ( F , G ) is flabby whenever G is injective. ◮ Let us make some preparation. For any F , F ′ ∈ Sh( X ), we have a natural morphism f ∗ H om X ( F , F ′ ) → H om Y ( f ∗ F , f ∗ F ′ ) ◮ Since sections with compact supports are mapped to sections with compact support, this induces f ∗ H om X ( F , F ′ ) → H om Y ( f ! F , f ! F ′ ) ◮ Now we want to derive both to H om X ( F , F ′ ) → R H om Y ( Rf ∗ F , Rf ∗ F ′ ) Rf ∗ R Rf ∗ R H om X ( F , F ′ ) → R H om Y ( Rf ! F , Rf ! F ′ )

Sheaf Hom and derived functors f ∗ H om X ( F , F ′ ) → H om Y ( f ∗ F , f ∗ F ′ ) , f ∗ H om X ( F , F ′ ) → H om Y ( f ! F , f ! F ′ ) ◮ There are some issues. To begin with, to have Rf ∗ F or Rf ! F on the left of a derived Hom we need the complex to be in D − (Sh( X )). Even if we assume F ∈ D b (Sh( X )) (or just a sheaf), we still need Rf ∗ to preserve the bounded above property. ◮ There is a fact that flabby dimension ≤ c-soft dimension +1. That is, if we know H i c ( X ; F ) = 0 for F ∈ Sh( X ) and all i > n , then we can conclude every sheaf F has a flabby resolution 0 → F → I 0 → I 1 → ... → I n +1 → 0. ◮ Using this, we have F ∈ D b (Sh( X )) ⇒ Rf ∗ F ∈ D b (Sh( X )). We already know this for Rf ! F . ◮ Now how do we derive the transformations at the top? For the first, we can use a flabby resolution of F ′ given by direct product of skyscraper sheaves. Then f ∗ F ′ is also a direct product of skyscraper sheaves, and so is H om X ( F , F ′ ), so we are happy. ◮ But what about the f ! one? I think this should be do-able by replacing flabby with c-soft appropriately, but I haven’t figured out how. Kashiwara-Shapira leaves this as an exercise (2.6.25).

Sheaf version Verdier duality H om X ( F , F ′ ) → R H om Y ( Rf ! F , Rf ! F ′ ) Rf ∗ R ◮ Now we have H om X ( F , f ! G ) → R H om ( Rf ! F , Rf ! f ! G ) → R Rf ∗ R H om ( Rf ! F , G ) thanks to the ordinary Verdier duality Rf ! ◦ f ! → id D + (Sh( Y )) . To prove that this an (quasi)-isomorphism, we verify for any U ⊂ Y open H om X ( F , f ! G )( U ) H om X ( F , f ! G )( U )) R k f ∗ R H k ( Rf ∗ R = H om ( F , f ! G ))) H k ( R Γ( f − 1 ( U ) , R H om ( F , f ! G ))) H k ( R Γ( U , Rf ∗ R = = H k (RHom Sh( f − 1 ( U )) ( F , f ! G )) Hom Sh( f − 1 ( U )) ( F , f ! G [ k ]) = = H k (RHom Sh( U ) ( Rf ! F , G )) = Hom Sh( U ) ( Rf ! F , G [ k ]) = H k ( R Γ( U , R R k R = H om U ( Rf ! F , G ))) = H om Y ( Rf ! F , G )( U ) Here we have abuse F for F| f − 1 ( U ) , etc. The sixth equality - the one at the beginning of fourth line - is the original Verdier duality.

Poincar´ e-Verdier duality ◮ Having proved Verdier duality, our next goal is to compute f ! G , at least for very nice maps for constant sheaf? The most important assertion is that: ◮ Theorem. Suppose f : X → Y is a topological submersion of (real) dimension n (i.e. locally on U ⊂ X it looks like U = f ( U ) × R n → f ( U )) where Y is locally compact Hausdorff. Then f ! Z Y = O Y / X [ n ], where O Y / X is the locally constant sheaf on X with fiber Z that records the orientation of the fibers. ◮ Corollary. Suppose f : X → Y is a smooth morphism of complex variety of (complex) dimension n . Then f ! Z Y = Z X [2 n ]. ◮ How do we prove this? The expectation that f ! Z Y concentrates at one degree helps, cause it suffices to compute H k ( f ! Z Y ). ◮ But H k ( f ! Z Y ) is the sheafification of U �→ R k Γ( U ; f ! Z | U ). ◮ We have R Γ( U ; ( f ! Z Y ) | U ) = RHom Sh( U ) ( Z U , ( f ! Z Y ) | U ) = RHom Sh( f ( U )) ( Rf ! Z U , Z f ( U ) ) .

Poincar´ e-Verdier duality, II H k ( f ! Z Y ) is the sheafification of U �→ R k Γ( U ; f ! Z | U ), and R k Γ( U ; f ! Z | U ) = H k RHom Sh( f ( U )) ( Rf ! Z U , Z f ( U ) ) ◮ It suffices to consider those U shrunk so that U = f ( U ) × R n . Proper base change tells us R k f ! Z U is locally (on f ( U )) given by � 0 , if k � = n H k c ( R n ; Z ) = Z , if k = n ◮ We have RHom Sh( f ( U )) ( Rf ! Z U , Z f ( U ) ) = RHom Sh( f ( U )) ( Z [ − n ] , Z ). ◮ If we further shrink U so that U is contractible 1 , then RHom Sh( f ( U )) ( Z [ − n ] , Z ) = Z . ◮ This proves that f ! Z Y only supported at degree − n with a locally constant sheaf with stalk Z there. Carefully tracking the c ( R n ; Z ) ∼ isomorphism H n = Z will lead us to the fiber/relative orientation sheaf - let’s leave it to next time. 1 This assumes Y locally contractible; see [KS, Prop. 3.3.2] for a more careful proof without this assumption.