- pm characteristic of field 0 / O w H ( a t b Y ' = at t b t - PDF document

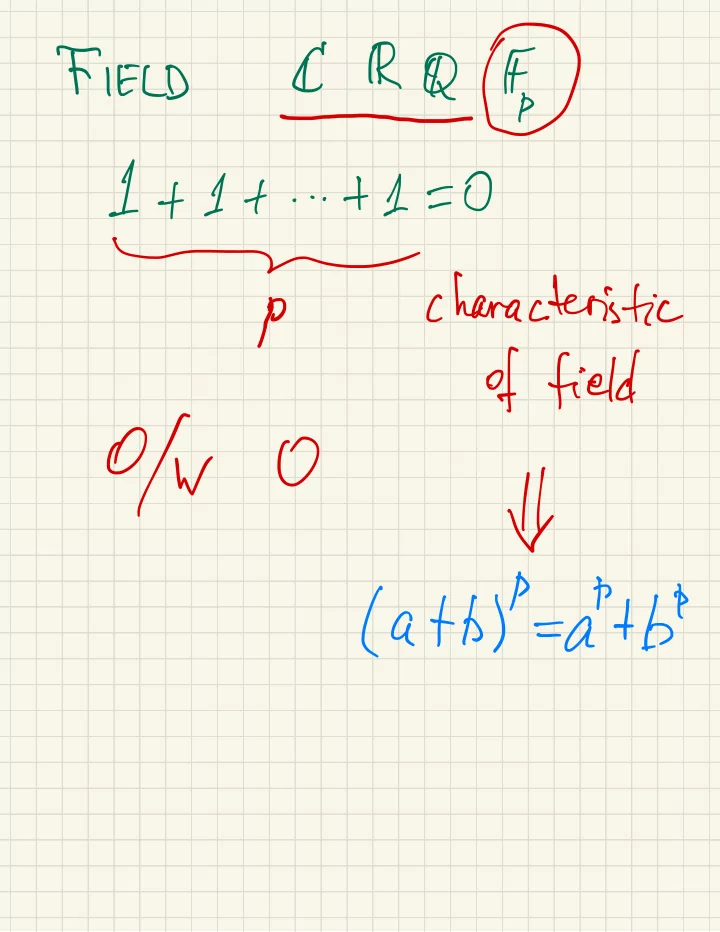
1%20 FIELD I t I t . t 1 = O . . - pm characteristic of field 0 / O w H ( a t b Y ' = at t b t

Hypergraph . , E ) vertex ( V set bertie laniary V E PCV ) E " IV Hh edges v IE km

STERNER 'S TAM .÷m4I Uniform hypergraph : Hedge same size km :@ HE E) HAK k ) - 2- uniform hyper : GRAPH . oh -

Bipartite graph Oi :# . ran - - ( V , E ) H - ¥ . " incidence graph of Tl

- ( v , E ) se - . , Am } E - - { Ae , . . K EV an independent set k DEI is it Kill Ai Ek )

" SET 81=3 CARDS cards - V =ffz4 " } { ={ " SETS 40,21471 T T affine lines vertex - uniform 3 Steiner triple system STS off on table , 12 Cards try to pick SET a 16 cards w/o { o , 134 SET : . I ) ( O , I , I

IN DEFENCE NUMBER : DEF L ( Jl ) = wax size of an indep set - L ( SET gone ) 216 up d( SET gear ) look - d- dine SET : STS ✓ = Ed E : affine lines - { Etbtltet ) ← b. to

24216W Ld =L ( d - din EET ) E 3d 24 E xd vertices : ( x . - xd ) , Xi Eff d 2 E Fd E3 : - ein Id 'd the L exists E L E 3 2 Actually ↳ 2 was TAM L <3 major open problem =

? L exists fee Z di de EI Super multiplicative FEKETE 's Lenne > 0 an sequence , an , then seep meet . F lima ! = sup a' in h h → no - Z a' ¥ S L desk toy - c) d a 43 L > 2 - za c > % art n - -

HW find int . many Ltte ) > I STS s s .t . # Covering number T ( transversal # ) ' tan Hitting number hitting set ← min Vertex cover

vertex : deg (a) Ded of ne Ai } # il ← edges - COVER ALGOR : GREEDY vertex of highest degree pick remove all incident edges repeat - THMCL-ovisetgreedyaEE.am E = win size of cover N P - hard even for graphs

⇒ K - unit hypergraph repeated who edges me - Complete k - uniform hypergraph : k¥2 m = he (E) d k . h m luhcnaknkraak ( H ) = me x { I Al l AEE }

Chromatic number X Ichi woe air . c : V → { colors ) no edge is sit . monochromatic i. e. HAE E ) ( I 4 All 22 ) X ( Jl ) = win # colors in legal coloring

- k¥4 Je k - uniform ÷ . we : ④ ' : Complete k - arif hyp Knc " . vertices .¥I"÷* at I Lh

↳ " ¥tn¥ :* used 2 t colors = E. n KI - K =3 n 75 ⇒ X > 2 case •• PHP general case HW

m Cr ) = aim # edges of r - unit . hypergraph an that 2- adorable is Not - - I > I ERDE 's m Cr ) BONUS • ye r - unit . if i. e - I r E 2 m 2 . adorable → - random coloring shorP(bga CH m ( r ) e . I c. r2 ERDO 'S - ie m

matching : set of disjoint edges O O O z ( Jl ) = wax # dig edges ( hee - ① so E t ② . so if R K - unit c- Ek - " dual quantities " systems Linear programming Finis of -