notation a real variable ( Pf ) 2 0 Pf the prevision of f P : - PowerPoint PPT Presentation

notation a real variable ( Pf − µ ) 2 0 µ Pf the prevision of f P : a prevision (expectation operator) f : a gamble (bounded real function)

variance notation the variance P ( f − Pf ) 2 of f under P ( Pf − µ ) 2 + V P f V P f µ Pf

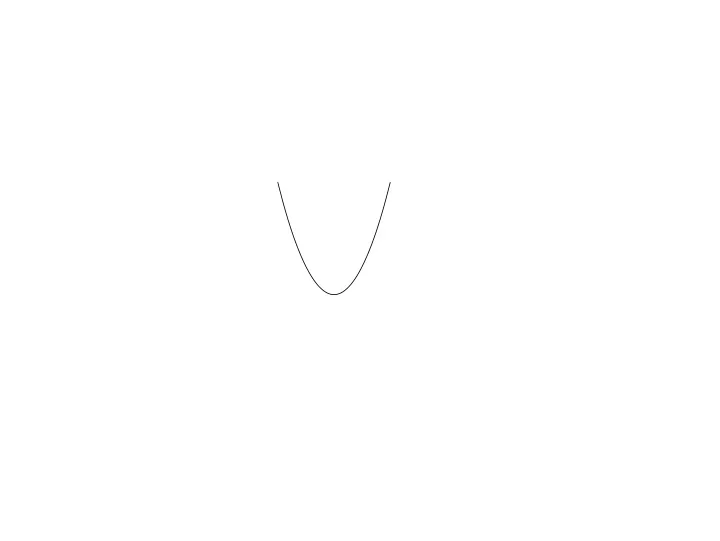
variance the variance P ( f − µ + µ − Pf ) 2 of f under P ( Pf − µ ) 2 + V P f = P ( f − µ ) 2 µ ∈ R P ( f − µ ) 2 V P f := min µ Pf the variance of f under P as an optimization problem ( f − µ ) 2 : a gamble for every µ

notation the credal set M P has 3 extreme points µ Pf Pf the lower prevision of f the upper prevision of f P : a lower prevision P : the conjugate upper prevision; Pf = − P ( − f )

envelopes P ( f − µ ) 2 = max P ∈M P P ( f − µ ) 2 P ( f − µ ) 2 = min P ∈M P P ( f − µ ) 2 µ Pf Pf

envelopes and a set P ( f − µ ) 2 = max P ∈M P P ( f − µ ) 2 � µ ∈ R P ( f − µ ) 2 � � min � P ∈ M P � P ( f − µ ) 2 = min P ∈M P P ( f − µ ) 2 µ Pf Pf

Lower & upper variance notation P ( f − µ ) 2 = max P ∈M P P ( f − µ ) 2 µ ∈ R P ( f − µ ) 2 V P f := min � � � V P f � P ∈ M P � µ ∈ R P ( f − µ ) 2 P ( f − µ ) 2 = min V P f := min P ∈M P P ( f − µ ) 2 µ Pf Pf the lower and upper variance of f under P as optimization problems

Lower & upper variance P ( f − µ ) 2 = max P ∈M P P ( f − µ ) 2 µ ∈ R P ( f − µ ) 2 V P f := min � � � V P f � P ∈ M P � µ ∈ R P ( f − µ ) 2 P ( f − µ ) 2 = min V P f := min P ∈M P P ( f − µ ) 2 µ Pf Pf Walley’s variance envelope theorem: V P f = min and V P f = max P ∈M P V P f P ∈M P V P f .

Lower & upper co variance Erik Quaeghebeur SMPS 2008

notation a real variable ( Pf − µ ) · ( Pg − ν ) ν Pg 0 µ Pf prevision of the gamble g

covariance notation � ( f − Pf ) · ( g − Pg ) � the covariance P of f and g under P ( Pf − µ ) · ( Pg − ν ) + C P { f , g } ν Pg C P { f , g } µ Pf

covariance � ( f − µ + µ − Pf ) · ( g − ν + ν − Pg ) � the covariance P of f and g under P ( Pf − µ ) · ( Pg − ν ) + C P { f , g } ν � ( f − µ ) · ( g − ν ) � = P Pg C P { f , g } µ Pf

covariance α = µ + ν 2 ( Pf − µ ) · ( Pg − ν ) + C P { f , g } ν � ( f − µ ) · ( g − ν ) � = P P f + g 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � C P { f , g } := min α ∈ R max β ∈ R P 2 2 P f − g 2 µ β = µ − ν the covariance of f and g under P 2 as an optimization problem − α ) 2 − ( f − g ( f + g − β ) 2 : 2 2 a gamble for every α and β

covariance α ( Pf − µ ) · ( Pg − ν ) + C P { f , g } � ( f − µ ) · ( g − ν ) � = P P f + g 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � C P { f , g } := min α ∈ R max β ∈ R P 2 2 P f − g f + g f − g = V P − V P 2 2 2 β

1 . 2 4 1 3 . 7

the credal set M P has 4 extreme points ν 1 . 2 Pg 4 Pg 1 3 . 7 µ Pf Pf

P f + g α 2 the credal set M P has 4 extreme points 1 . 2 4 P f + g 2 P f − g 1 3 . 7 2 P f − g β 2

envelopes P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = min P ∈M P P P f + g 2 P f − g 2 P f − g β 2

envelopes P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = max P ∈M P P P f + g 2 P f − g 2 P f − g β 2

envelopes and a set P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = min P ∈M P P P f + g 2 P f − g 2 P f − g β 2 − α ) 2 − ( f − g � − β ) 2 � � � � ( f + g min α ∈ R max β ∈ R P � P ∈ M P � 2 2

envelopes and a set P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = max P ∈M P P P f + g 2 P f − g 2 P f − g β 2 − α ) 2 − ( f − g � − β ) 2 � � � � ( f + g min α ∈ R max β ∈ R P � P ∈ M P � 2 2

Lower & upper covariance notation P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = min P ∈M P P P f + g 2 � � � C P { f , g } � P ∈ M P � P f − g 2 the lower covariance of f and g under P P f − g β 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � C P { f , g } := min α ∈ R max β ∈ R P 2 2 ?

Lower & upper covariance notation P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = max P ∈M P P P f + g 2 � � � C P { f , g } � P ∈ M P � P f − g 2 the upper covariance of f and g under P P f − g β 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � C P { f , g } := max β ∈ R min α ∈ R P 2 2 ?

Lower & upper covariance P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = min P ∈M P P P f + g 2 � � � C P { f , g } � P ∈ M P � P f − g 2 the covariance envelope theorem P f − g β 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � C P { f , g } := min α ∈ R max β ∈ R P 2 2 = min P ∈M P C P { f , g }

Lower & upper covariance P f + g α 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � P 2 2 � ( f + g 2 − α ) 2 − ( f − g 2 − β ) 2 � = max P ∈M P P P f + g 2 � � � C P { f , g } � P ∈ M P � P f − g 2 the covariance envelope theorem P f − g β 2 − α ) 2 − ( f − g � ( f + g − β ) 2 � C P { f , g } := max β ∈ R min α ∈ R P 2 2 = max P ∈M P C P { f , g }

Conclusion We have found a definition of lower and upper covariance under coherent lower previsions that ◮ is direct, in the sense that it does not make use of the credal set of the lower prevision; ◮ and satisfies a covariance envelope theorem. Moreover, it generalizes – as it should – the existing optimization problem definitions for covariance and (lower and upper) variance

Open questions ◮ Can this idea be extended to other, higher order central moments? In other words, can a definition be found for lower and upper versions of these moments under a coherent lower prevision that ◮ is direct, in the sense that it does not make use of the credal set of the lower prevision; ◮ and satisfies a higher order central moment envelope theorem? ◮ What is the (behavioral) meaning of an upper and lower covariance or, for that matter, lower and upper variance?