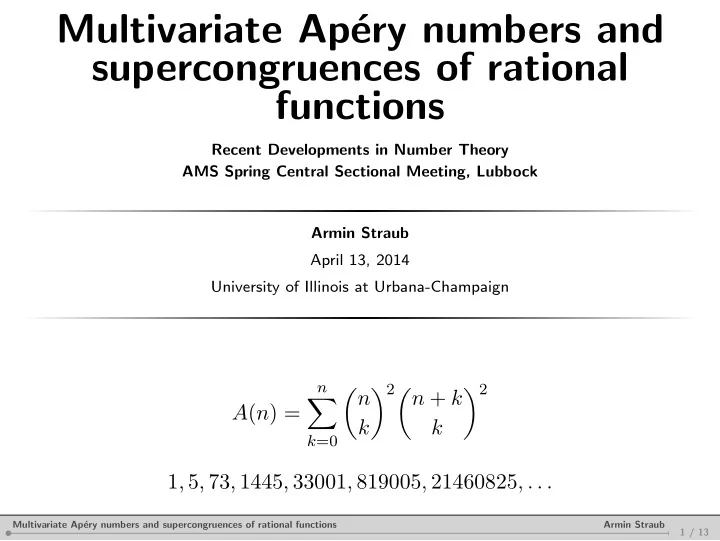

Multivariate Ap´ ery numbers and supercongruences of rational functions Recent Developments in Number Theory AMS Spring Central Sectional Meeting, Lubbock Armin Straub April 13, 2014 University of Illinois at Urbana-Champaign n � 2 � n + k � 2 � n � A ( n ) = k k k =0 1 , 5 , 73 , 1445 , 33001 , 819005 , 21460825 , . . . Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 1 / 13
Ap´ ery numbers and the irrationality of ζ (3) • The Ap´ ery numbers 1 , 5 , 73 , 1445 , . . . n � 2 � n + k � 2 � n � A ( n ) = k k satisfy k =0 ( n + 1) 3 u n +1 = (2 n + 1)(17 n 2 + 17 n + 5) u n − n 3 u n − 1 . Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 2 / 13
Ap´ ery numbers and the irrationality of ζ (3) • The Ap´ ery numbers 1 , 5 , 73 , 1445 , . . . n � 2 � n + k � 2 � n � A ( n ) = k k satisfy k =0 ( n + 1) 3 u n +1 = (2 n + 1)(17 n 2 + 17 n + 5) u n − n 3 u n − 1 . ζ (3) = � ∞ 1 THM n 3 is irrational. n =1 Ap´ ery ’78 The same recurrence is satisfied by the “near”-integers proof n n k � 2 � n + k � 2 ( − 1) m − 1 � n 1 � � � . B ( n ) = j 3 + 2 m 3 � n �� n + m � k k m m j =1 m =1 k =0 Then, B ( n ) A ( n ) → ζ (3) . But too fast for ζ (3) to be rational. Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 2 / 13
Ap´ ery-like numbers • Recurrence for Ap´ ery numbers is the case ( a, b, c ) = (17 , 5 , 1) of ( n + 1) 3 u n +1 = (2 n + 1)( an 2 + an + b ) u n − cn 3 u n − 1 . Are there other tuples ( a, b, c ) for which the solution defined by Q u − 1 = 0 , u 0 = 1 is integral? Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 3 / 13
Ap´ ery-like numbers • Recurrence for Ap´ ery numbers is the case ( a, b, c ) = (17 , 5 , 1) of ( n + 1) 3 u n +1 = (2 n + 1)( an 2 + an + b ) u n − cn 3 u n − 1 . Are there other tuples ( a, b, c ) for which the solution defined by Q u − 1 = 0 , u 0 = 1 is integral? • Essentially, only 14 tuples ( a, b, c ) found. (Almkvist–Zudilin) • 4 hypergeometric and 4 Legendrian solutions • 6 sporadic solutions Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 3 / 13
Ap´ ery-like numbers • Recurrence for Ap´ ery numbers is the case ( a, b, c ) = (17 , 5 , 1) of ( n + 1) 3 u n +1 = (2 n + 1)( an 2 + an + b ) u n − cn 3 u n − 1 . Are there other tuples ( a, b, c ) for which the solution defined by Q u − 1 = 0 , u 0 = 1 is integral? • Essentially, only 14 tuples ( a, b, c ) found. (Almkvist–Zudilin) • 4 hypergeometric and 4 Legendrian solutions • 6 sporadic solutions • Similar (and intertwined) story for: • ( n + 1) 2 u n +1 = ( an 2 + an + b ) u n − cn 2 u n − 1 (Beukers, Zagier) • ( n + 1) 3 u n +1 = (2 n + 1)( an 2 + an + b ) u n − n ( cn 2 + d ) u n − 1 (Cooper) Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 3 / 13
Ap´ ery-like numbers • Hypergeometric and Legendrian solutions have generating functions � 1 � 2 � � 2 , α, 1 − α � 1 � α, 1 − α − C α z � � 3 F 2 � 4 C α z , 1 − C α z 2 F 1 , � � 1 , 1 1 1 − C α z � 6 and C α = 2 4 , 3 3 , 2 6 , 2 4 · 3 3 . with α = 1 2 , 1 3 , 1 4 , 1 • The six sporadic solutions are: ( a, b, c ) A ( n ) k ( − 1) k 3 n − 3 k � n � (3 k )! �� n + k (7 , 3 , 81) � 3 k n k ! 3 � 3 �� 4 n − 5 k − 1 �� k ( − 1) k � n � 4 n − 5 k � � (11 , 5 , 125) + k 3 n 3 n � 2 � 2 k � n �� 2( n − k ) � (10 , 4 , 64) � k k k n − k � 2 � 2 � 2 k � n � (12 , 4 , 16) k k n � 2 � n � n �� k �� k + l � � (9 , 3 , − 27) k,l k l l n � 2 � 2 � n + k � n (17 , 5 , 1) � k k n Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 4 / 13
Modularity of Ap´ ery-like numbers • The Ap´ ery numbers 1 , 5 , 73 , 1145 , . . . n � 2 � n + k � 2 � n � A ( n ) = k k satisfy k =0 � η ( τ ) η (6 τ ) � 12 n η 7 (2 τ ) η 7 (3 τ ) � = A ( n ) . η 5 ( τ ) η 5 (6 τ ) η (2 τ ) η (3 τ ) n � 0 modular form modular function Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 5 / 13
Modularity of Ap´ ery-like numbers • The Ap´ ery numbers 1 , 5 , 73 , 1145 , . . . n � 2 � n + k � 2 � n � A ( n ) = k k satisfy k =0 � η ( τ ) η (6 τ ) � 12 n η 7 (2 τ ) η 7 (3 τ ) � = A ( n ) . η 5 ( τ ) η 5 (6 τ ) η (2 τ ) η (3 τ ) n � 0 modular form modular function Not at all evidently, such a modular parametrization exists for FACT all known Ap´ ery-like numbers! • Context: f ( τ ) modular form of weight k x ( τ ) modular function y ( x ) such that y ( x ( τ )) = f ( τ ) Then y ( x ) satisfies a linear differential equation of order k + 1 . Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 5 / 13
Supercongruences for Ap´ ery numbers • The Ap´ ery numbers satisfy the supercongruence ( p � 5 ) Chowla–Cowles–Cowles ’80 A ( mp r ) ≡ A ( mp r − 1 ) mod p 3 r . Gessel ’82 Beukers, Coster ’85, ’88 Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 6 / 13
Supercongruences for Ap´ ery numbers • The Ap´ ery numbers satisfy the supercongruence ( p � 5 ) Chowla–Cowles–Cowles ’80 A ( mp r ) ≡ A ( mp r − 1 ) mod p 3 r . Gessel ’82 Beukers, Coster ’85, ’88 Simple combinatorics proves the congruence EG � 2 p � � p �� � p � mod p 2 . = ≡ 1 + 1 p − k p k k For p � 5 , Wolstenholme’s congruence shows that, in fact, � 2 p � mod p 3 . ≡ 2 p Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 6 / 13
Supercongruences for Ap´ ery-like numbers • Conjecturally, supercongruences like A ( mp r ) ≡ A ( mp r − 1 ) mod p 3 r hold for all Ap´ ery-like numbers. Osburn–Sahu ’09 • Current state of affairs for the six sporadic sequences from earlier: ( a, b, c ) A ( n ) k ( − 1) k 3 n − 3 k � n � (3 k )! �� n + k (7 , 3 , 81) � open!! 3 k n k ! 3 � 3 �� 4 n − 5 k − 1 �� k ( − 1) k � n � 4 n − 5 k � � (11 , 5 , 125) + Osburn–Sahu–S ’13 k 3 n 3 n � 2 � 2 k � n �� 2( n − k ) � (10 , 4 , 64) � Osburn–Sahu ’11 k k k n − k � 2 � 2 � 2 k � n � (12 , 4 , 16) Osburn–Sahu–S ’13 k k n � 2 � n � n �� k �� k + l � � (9 , 3 , − 27) open k,l k l l n � 2 � 2 � n + k � n (17 , 5 , 1) � Beukers, Coster ’87-’88 k k n Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 7 / 13
Sources for (non-super) congruences a ( np r ) ≡ a ( np r − 1 ) (mod p r ) (C) • a ( n ) is realizable if there is some map T : X → X such that a ( n ) = # { x ∈ X : T n x = x } . “points of period n ” In that case, (C) holds. Everest–van der Poorten–Puri–Ward ’02, Arias de Reyna ’05 In fact, up to a positivity condition, (C) characterizes realizability. Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 8 / 13
Sources for (non-super) congruences a ( np r ) ≡ a ( np r − 1 ) (mod p r ) (C) • a ( n ) is realizable if there is some map T : X → X such that a ( n ) = # { x ∈ X : T n x = x } . “points of period n ” In that case, (C) holds. Everest–van der Poorten–Puri–Ward ’02, Arias de Reyna ’05 In fact, up to a positivity condition, (C) characterizes realizability. • Let Λ( x ) ∈ Z p [ x ± 1 1 , . . . , x ± 1 d ] be a Laurent polynomial. If the Newton polyhedron of Λ contains the origin as its only interior point, then a ( n ) = ct Λ( x ) n satisfies (C). van Straten–Samol ’09 Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 8 / 13
Sources for (non-super) congruences a ( np r ) ≡ a ( np r − 1 ) (mod p r ) (C) • a ( n ) is realizable if there is some map T : X → X such that a ( n ) = # { x ∈ X : T n x = x } . “points of period n ” In that case, (C) holds. Everest–van der Poorten–Puri–Ward ’02, Arias de Reyna ’05 In fact, up to a positivity condition, (C) characterizes realizability. • Let Λ( x ) ∈ Z p [ x ± 1 1 , . . . , x ± 1 d ] be a Laurent polynomial. If the Newton polyhedron of Λ contains the origin as its only interior point, then a ( n ) = ct Λ( x ) n satisfies (C). van Straten–Samol ’09 � ∞ � a ( n ) � n T n • If a (1) = 1 , then (C) is equivalent to exp ∈ Z [[ T ]] . n =1 This is a natural condition in formal group theory . Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 8 / 13
Ap´ ery numbers as diagonals • Given a series � a ( n 1 , . . . , n d ) x n 1 1 · · · x n d F ( x 1 , . . . , x d ) = d , n 1 ,...,n d � 0 its diagonal coefficients are the coefficients a ( n, . . . , n ) . The Ap´ ery numbers are the diagonal coefficients of THM S 2013 1 . (1 − x 1 − x 2 )(1 − x 3 − x 4 ) − x 1 x 2 x 3 x 4 Multivariate Ap´ ery numbers and supercongruences of rational functions Armin Straub 9 / 13
Recommend
More recommend