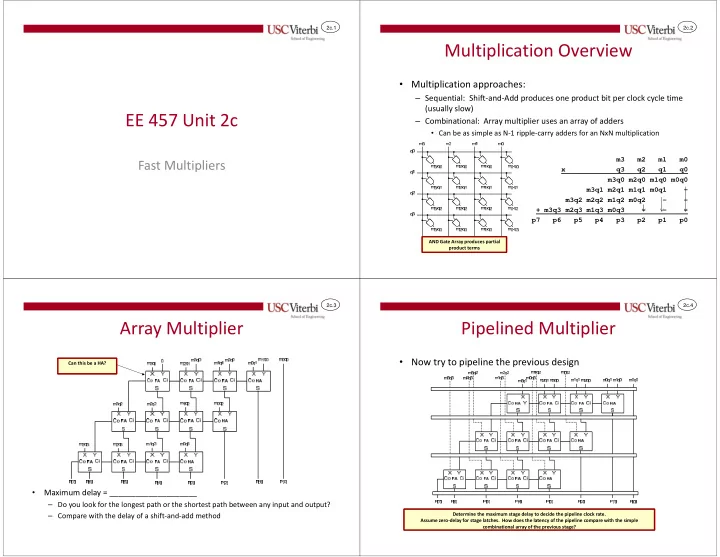

2c.1 2c.2 Multiplication Overview • Multiplication approaches: – Sequential: Shift-and-Add produces one product bit per clock cycle time (usually slow) EE 457 Unit 2c – Combinational: Array multiplier uses an array of adders • Can be as simple as N-1 ripple-carry adders for an NxN multiplication m3 m2 m1 m0 Fast Multipliers x q3 q2 q1 q0 m3q0 m2q0 m1q0 m0q0 m3q1 m2q1 m1q1 m0q1 - m3q2 m2q2 m1q2 m0q2 - - + m3q3 m2q3 m1q3 m0q3 - - - p7 p6 p5 p4 p3 p2 p1 p0 AND Gate Array produces partial product terms 2c.3 2c.4 Array Multiplier Pipelined Multiplier • Now try to pipeline the previous design Can this be a HA? • Maximum delay = ____________________ – Do you look for the longest path or the shortest path between any input and output? Determine the maximum stage delay to decide the pipeline clock rate. – Compare with the delay of a shift-and-add method Assume zero-delay for stage latches. How does the latency of the pipeline compare with the simple combinational array of the previous stage?
2c.5 2c.6 Carry-Save Multiplier Carry Save Adders • Instead of propagating the carries to the left in the same row, carries are • Consider the decimal addition of now sent down to the next stage to reduce stage delay and facilitate 47 + 96 + 58 = 201 pipelining • One way is to add ________ to get ____ and _____ m1q0 m0q0 m3q0 m2q0 0 0 0 m3q1 m1q1 m0q1 m2q1 Here the _____ column cannot be added ___________ is produced • X Y X Y X Y CSA’s Co Ci Co Ci Co Ci FA FA FA In the carry-save style, we add the ____ column and _____ column • S S S simultaneous m1q2 m0q2 m3q2 m2q2 X Y X Y X Y Co Ci Co Ci Co Ci FA FA FA S S S 1 1 4 7 4 7 m1q3 m0q3 m3q3 m2q3 The upper three stages are 3-bit + 9 6 9 6 X Y X Y X Y Carry Save Adders (CSA’s) each Co Ci Co Ci Co Ci 1 FA FA FA 1 4 3 + 5 8 with 2-gate delays. S S S 3 2 1 RCA + 5 8 2 1 1 The last stage is a Ripple Carry 2 Adder (RCA) which requires X Y X Y X Y 2 0 1 + 1 8 _ longer delay. It can be replaced Co Ci Co Ci Co Ci FA FA FA 0 5 4 6 2 0 1 by a CLA for larger multipliers. S S S 4 3 P[7] P[6] P[5] P[1] P[0] P[4] P[3] P[2] 2c.7 2c.8 Carry-Save (3,2) Adders 1-bit FA vs. 1-bit CSA • A carry save adder is also called a (3,2) • Any difference between an ordinary full adder and 1- adder or a (3,2) counter (refer to bit CSA? 0 1 0 1 Computer Arithmetic Algorithms by 1 0 0 1 Israel Koren) as it takes three vectors, + 1 0 1 1 adds them up, and reduces them to 1 0 0 1 _ Carry vector two vectors, namely a sum vector and a 0 1 1 1 Sum vector carry vector • 16-bit wide CSA takes ( more / equal / less ) time to • CSA’s are based on the principle that produce its outputs compared to an 8-bit wide CSA carries do not have to be added _______________, but can be • Carry-save adder ( is / is not ) useful in adding only 2 combined ______________ numbers • An n-bit CSA consist of n disjoint full adders
2c.9 2c.10 CSA Organization Wallace Tree Multiplier • Using the previous example as a • We can arrange our template, to build an NxN multiplier q7·M q6·M q5·M q4·M q3·M q2·M q1·M q0·M CSA’s in a _______ you need (n-1) of CSA CSA manner where ____ (n-1) bit CSAs followed by a CSA CSA partial product is final (n-1)-bit RCA • Delay = Delay of (n-1) CSA’s CSA added per CSA (after + Delay of (n-1) bit RCA the first level) CSA = ______________________ Propagation Adder • We can reduce the CSA component Product of the delay by organizing the CSA’s Note: The vectors (partial products) in a _____ (i.e. ___________ delay) need to be aligned before summing. These details are not shown in the block diagram. 2c.11 2c.12 Logic Delay Wallace Tree Discussion Consider the gate • • A 4-input OR gate reduces 4 literals to 1 (i.e. a factor of 4 arrangement for OR’ing reduction) 8 bits • A CSA reduces 3 vectors to 2 vectors (i.e. a factor of 1.5) Linear: • – This reduction factor may not be convenient to develop an efficient tree Delay = __ gates – to sum 16 or 32 partial products Tree • – Wallace tree may not achieve a great reduction in delay due to wastage of Depth of tree = ____ = – an extra level __ levels • Consider OR’ing 16-bits • Also not the Wallace tree shown earlier does not show… using 4-bit OR gates, – Size of buses how many levels would – What bits are “retired” progressivley you need? – Relative significance (alignment) of partial products – Size of the carry-propagate adder (e.g. RCA or CLA) needs to be figured out and overall delay estimated
2c.13 2c.14 10 9 8 7 6 5 4 3 2 1 0 10 9 8 7 6 5 4 3 2 1 0 Original 6x6 Matrix Reorganized 6x6 matrix 10 9 8 7 6 5 4 3 2 1 0 10 9 8 7 6 5 4 3 2 1 0 Level 1 CSA Level 2 CSA 10 9 8 7 6 5 4 3 2 1 0 11 10 9 8 7 6 5 4 3 2 1 0 Results of Level 1 Level 3 CSA 2c.15 Credits • These slides were derived from Gandhi Puvvada’s EE 457 Class Notes
Recommend
More recommend